LeetCode 106.从中序与后序遍历序列构造二叉树
LeetCode 106.从中序与后序遍历序列构造二叉树
1、题目
题目链接:106. 从中序与后序遍历序列构造二叉树
给定两个整数数组 inorder 和 postorder ,其中 inorder 是二叉树的中序遍历, postorder 是同一棵树的后序遍历,请你构造并返回这颗 二叉树 。
示例 1:
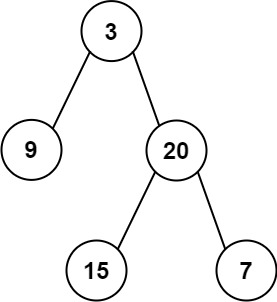
输入:inorder = [9,3,15,20,7], postorder = [9,15,7,20,3]
输出:[3,9,20,null,null,15,7]
示例 2:
输入:inorder = [-1], postorder = [-1]
输出:[-1]
提示:
- 1 <= inorder.length <= 3000
- postorder.length == inorder.length
- -3000 <= inorder[i], postorder[i] <= 3000
- inorder 和 postorder 都由 不同 的值组成
- postorder 中每一个值都在 inorder 中
- inorder 保证是树的中序遍历
- postorder 保证是树的后序遍历
2、递归
思路
首先解决这道题我们需要明确给定一棵二叉树,我们是如何对其进行中序遍历与后序遍历的:
中序遍历的顺序是每次遍历左孩子,再遍历根节点,最后遍历右孩子。
后序遍历的顺序是每次遍历左孩子,再遍历右孩子,最后遍历根节点。
那么如何根据两个顺序构造一个唯一的二叉树,就是以后序数组的最后一个元素为切割点,先切中序数组,根据中序数组,反过来再切后序数组。一层一层切下去,每次后序数组最后一个元素就是节点元素。
流程如图:
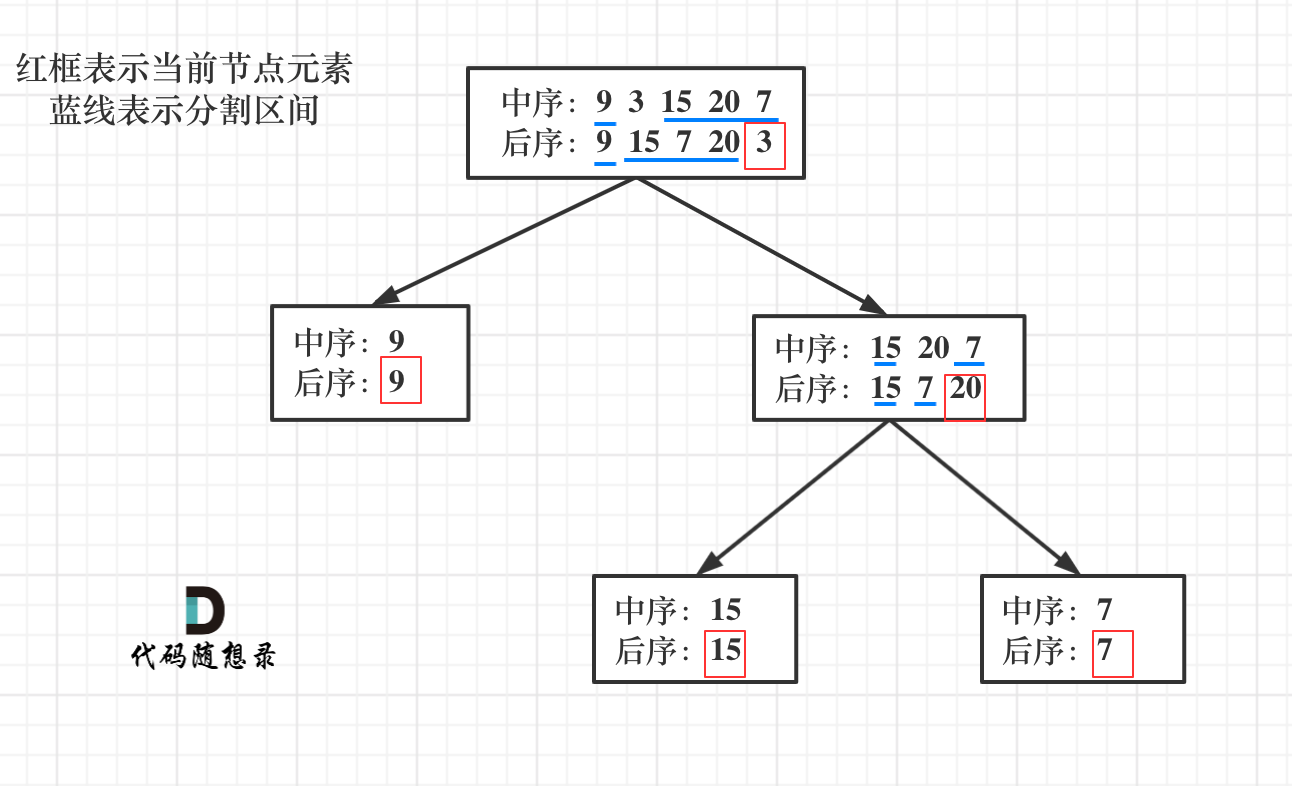
那么代码应该怎么写呢?
说到一层一层切割,就应该想到了递归。
来看一下一共分几步:
- 第一步:如果数组大小为零的话,说明是空节点了。
- 第二步:如果不为空,那么取后序数组最后一个元素作为节点元素。
- 第三步:找到后序数组最后一个元素在中序数组的位置,作为切割点
- 第四步:切割中序数组,切成中序左数组和中序右数组 (顺序别搞反了,一定是先切中序数组)
- 第五步:切割后序数组,切成后序左数组和后序右数组
- 第六步:递归处理左区间和右区间
不难写出如下代码:(先把框架写出来)
TreeNode* traversal (vector<int>& inorder, vector<int>& postorder) {// 第一步if (postorder.size() == 0) return NULL;// 第二步:后序遍历数组最后一个元素,就是当前的中间节点int rootValue = postorder[postorder.size() - 1];TreeNode* root = new TreeNode(rootValue);// 叶子节点if (postorder.size() == 1) return root;// 第三步:找切割点int delimiterIndex;for (delimiterIndex = 0; delimiterIndex < inorder.size(); delimiterIndex++) {if (inorder[delimiterIndex] == rootValue) break;}// 第四步:切割中序数组,得到 中序左数组和中序右数组// 第五步:切割后序数组,得到 后序左数组和后序右数组// 第六步root->left = traversal(中序左数组, 后序左数组);root->right = traversal(中序右数组, 后序右数组);return root;
}
难点大家应该发现了,就是如何切割,以及边界值找不好很容易乱套。
此时应该注意确定切割的标准,是左闭右开,还有左开右闭,还是左闭右闭,这个就是不变量,要在递归中保持这个不变量。
首先要切割中序数组,为什么先切割中序数组呢?
切割点在后序数组的最后一个元素,就是用这个元素来切割中序数组的,所以必要先切割中序数组。
中序数组相对比较好切,找到切割点(后序数组的最后一个元素)在中序数组的位置,然后切割,如下代码中我坚持左闭右开的原则:
// 找到中序遍历的切割点
int delimiterIndex;
for (delimiterIndex = 0; delimiterIndex < inorder.size(); delimiterIndex++) {if (inorder[delimiterIndex] == rootValue) break;
}// 左闭右开区间:[0, delimiterIndex)
vector<int> leftInorder(inorder.begin(), inorder.begin() + delimiterIndex);
// [delimiterIndex + 1, end)
vector<int> rightInorder(inorder.begin() + delimiterIndex + 1, inorder.end() );
接下来就要切割后序数组了。
首先后序数组的最后一个元素指定不能要了,这是切割点 也是 当前二叉树中间节点的元素,已经用了。
后序数组的切割点怎么找?
后序数组没有明确的切割元素来进行左右切割,不像中序数组有明确的切割点,切割点左右分开就可以了。
此时有一个很重的点,就是中序数组大小一定是和后序数组的大小相同的(这是必然)。
中序数组我们都切成了左中序数组和右中序数组了,那么后序数组就可以按照左中序数组的大小来切割,切成左后序数组和右后序数组。
代码如下:
// postorder 舍弃末尾元素,因为这个元素就是中间节点,已经用过了
postorder.resize(postorder.size() - 1);// 左闭右开,注意这里使用了左中序数组大小作为切割点:[0, leftInorder.size)
vector<int> leftPostorder(postorder.begin(), postorder.begin() + leftInorder.size());
// [leftInorder.size(), end)
vector<int> rightPostorder(postorder.begin() + leftInorder.size(), postorder.end());
此时,中序数组切成了左中序数组和右中序数组,后序数组切割成左后序数组和右后序数组。
接下来可以递归了,代码如下:
root->left = traversal(leftInorder, leftPostorder);
root->right = traversal(rightInorder, rightPostorder);
代码
完整代码如下:
class Solution {
public:TreeNode* traversal(vector<int>& inorder, vector<int>& postorder) {if (postorder.size() == 0) {return nullptr;}// 后序遍历的最后一个元素为根节点的值int rootValue = postorder[postorder.size() - 1];// 创建根节点TreeNode* root = new TreeNode(rootValue);if (postorder.size() == 1) {// 如果后序遍历数组只有一个元素,则直接返回根节点return root;}int delimiterIndex;// 在中序遍历数组中找到根节点的位置for (delimiterIndex = 0; delimiterIndex < postorder.size(); delimiterIndex++) {if (inorder[delimiterIndex] == rootValue) {break;}}// 切割中序遍历数组,得到左子树和右子树的中序遍历数组// 左闭右开区间:[0, delimiterIndex)vector<int> leftInorder(inorder.begin(), inorder.begin() + delimiterIndex);// 左闭右开区间:[delimiterIndex + 1, end)vector<int> rightInorder(inorder.begin() + delimiterIndex + 1, inorder.end());// postorder 舍弃末尾元素postorder.resize(postorder.size() - 1);// 切割后序遍历数组,得到左子树和右子树的后序遍历数组// 依然左闭右开,注意这里使用了左中序数组大小作为切割点// 左闭右开区间:[0, leftInorder.size())vector<int> leftPostorder(postorder.begin(), postorder.begin() + leftInorder.size());// 左闭右开区间:[leftInorder.size(), end)vector<int> rightPostorder(postorder.begin() + leftInorder.size(), postorder.end());// 递归构建左子树和右子树,并连接到根节点上root->left = traversal(leftInorder, leftPostorder);root->right = traversal(rightInorder, rightPostorder);return root;}TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {if (inorder.size() == 0 || postorder.size() == 0) {// 如果中序遍历或后序遍历的数组为空,则返回空指针return nullptr;}// 调用traversal函数构建树return traversal(inorder, postorder);}
};
复杂度分析
- 时间复杂度: O(n)
- 空间复杂度: O(n)
3、递归(带日志)
思路
相信大家自己就算是思路清晰, 代码写出来一定是各种问题,所以一定要加日志来调试,看看是不是按照自己思路来切割的,不要大脑模拟,那样越想越糊涂。
加了日志的代码如下:(加了日志的代码不要在leetcode上提交,容易超时)
代码
class Solution {
private:TreeNode* traversal (vector<int>& inorder, vector<int>& postorder) {if (postorder.size() == 0) return NULL;int rootValue = postorder[postorder.size() - 1];TreeNode* root = new TreeNode(rootValue);if (postorder.size() == 1) return root;int delimiterIndex;for (delimiterIndex = 0; delimiterIndex < inorder.size(); delimiterIndex++) {if (inorder[delimiterIndex] == rootValue) break;}vector<int> leftInorder(inorder.begin(), inorder.begin() + delimiterIndex);vector<int> rightInorder(inorder.begin() + delimiterIndex + 1, inorder.end() );postorder.resize(postorder.size() - 1);vector<int> leftPostorder(postorder.begin(), postorder.begin() + leftInorder.size());vector<int> rightPostorder(postorder.begin() + leftInorder.size(), postorder.end());// 以下为日志cout << "----------" << endl;cout << "leftInorder :";for (int i : leftInorder) {cout << i << " ";}cout << endl;cout << "rightInorder :";for (int i : rightInorder) {cout << i << " ";}cout << endl;cout << "leftPostorder :";for (int i : leftPostorder) {cout << i << " ";}cout << endl;cout << "rightPostorder :";for (int i : rightPostorder) {cout << i << " ";}cout << endl;root->left = traversal(leftInorder, leftPostorder);root->right = traversal(rightInorder, rightPostorder);return root;}
public:TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {if (inorder.size() == 0 || postorder.size() == 0) return NULL;return traversal(inorder, postorder);}
};
复杂度分析
- 时间复杂度: O(n)
- 空间复杂度: O(n)
4、递归(索引)
思路
此时应该发现了,如上的代码性能并不好,因为每层递归定义了新的vector(就是数组),既耗时又耗空间,但上面的代码是最好理解的,为了方便读者理解,所以用如上的代码来讲解。
下面给出用下标索引写出的代码版本:(思路是一样的,只不过不用重复定义vector了,每次用下标索引来分割)
代码
class Solution {
private:// 中序区间:[inorderBegin, inorderEnd),后序区间[postorderBegin, postorderEnd)TreeNode* traversal (vector<int>& inorder, int inorderBegin, int inorderEnd, vector<int>& postorder, int postorderBegin, int postorderEnd) {if (postorderBegin == postorderEnd) return NULL;int rootValue = postorder[postorderEnd - 1];TreeNode* root = new TreeNode(rootValue);if (postorderEnd - postorderBegin == 1) return root;int delimiterIndex;for (delimiterIndex = inorderBegin; delimiterIndex < inorderEnd; delimiterIndex++) {if (inorder[delimiterIndex] == rootValue) break;}// 切割中序数组// 左中序区间,左闭右开[leftInorderBegin, leftInorderEnd)int leftInorderBegin = inorderBegin;int leftInorderEnd = delimiterIndex;// 右中序区间,左闭右开[rightInorderBegin, rightInorderEnd)int rightInorderBegin = delimiterIndex + 1;int rightInorderEnd = inorderEnd;// 切割后序数组// 左后序区间,左闭右开[leftPostorderBegin, leftPostorderEnd)int leftPostorderBegin = postorderBegin;int leftPostorderEnd = postorderBegin + delimiterIndex - inorderBegin; // 终止位置是 需要加上 中序区间的大小size// 右后序区间,左闭右开[rightPostorderBegin, rightPostorderEnd)int rightPostorderBegin = postorderBegin + (delimiterIndex - inorderBegin);int rightPostorderEnd = postorderEnd - 1; // 排除最后一个元素,已经作为节点了root->left = traversal(inorder, leftInorderBegin, leftInorderEnd, postorder, leftPostorderBegin, leftPostorderEnd);root->right = traversal(inorder, rightInorderBegin, rightInorderEnd, postorder, rightPostorderBegin, rightPostorderEnd);return root;}
public:TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {if (inorder.size() == 0 || postorder.size() == 0) return NULL;// 左闭右开的原则return traversal(inorder, 0, inorder.size(), postorder, 0, postorder.size());}
};
复杂度分析
- 时间复杂度: O(n)
- 空间复杂度: O(n)
5、递归(索引带日志)
思路
那么这个版本写出来依然要打日志进行调试,打日志的版本如下:(该版本不要在leetcode上提交,容易超时)
代码
class Solution {
private:TreeNode* traversal (vector<int>& inorder, int inorderBegin, int inorderEnd, vector<int>& postorder, int postorderBegin, int postorderEnd) {if (postorderBegin == postorderEnd) return NULL;int rootValue = postorder[postorderEnd - 1];TreeNode* root = new TreeNode(rootValue);if (postorderEnd - postorderBegin == 1) return root;int delimiterIndex;for (delimiterIndex = inorderBegin; delimiterIndex < inorderEnd; delimiterIndex++) {if (inorder[delimiterIndex] == rootValue) break;}// 切割中序数组// 左中序区间,左闭右开[leftInorderBegin, leftInorderEnd)int leftInorderBegin = inorderBegin;int leftInorderEnd = delimiterIndex;// 右中序区间,左闭右开[rightInorderBegin, rightInorderEnd)int rightInorderBegin = delimiterIndex + 1;int rightInorderEnd = inorderEnd;// 切割后序数组// 左后序区间,左闭右开[leftPostorderBegin, leftPostorderEnd)int leftPostorderBegin = postorderBegin;int leftPostorderEnd = postorderBegin + delimiterIndex - inorderBegin; // 终止位置是 需要加上 中序区间的大小size// 右后序区间,左闭右开[rightPostorderBegin, rightPostorderEnd)int rightPostorderBegin = postorderBegin + (delimiterIndex - inorderBegin);int rightPostorderEnd = postorderEnd - 1; // 排除最后一个元素,已经作为节点了cout << "----------" << endl;cout << "leftInorder :";for (int i = leftInorderBegin; i < leftInorderEnd; i++) {cout << inorder[i] << " ";}cout << endl;cout << "rightInorder :";for (int i = rightInorderBegin; i < rightInorderEnd; i++) {cout << inorder[i] << " ";}cout << endl;cout << "leftpostorder :";for (int i = leftPostorderBegin; i < leftPostorderEnd; i++) {cout << postorder[i] << " ";}cout << endl;cout << "rightpostorder :";for (int i = rightPostorderBegin; i < rightPostorderEnd; i++) {cout << postorder[i] << " ";}cout << endl;root->left = traversal(inorder, leftInorderBegin, leftInorderEnd, postorder, leftPostorderBegin, leftPostorderEnd);root->right = traversal(inorder, rightInorderBegin, rightInorderEnd, postorder, rightPostorderBegin, rightPostorderEnd);return root;}
public:TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {if (inorder.size() == 0 || postorder.size() == 0) return NULL;return traversal(inorder, 0, inorder.size(), postorder, 0, postorder.size());}
};
复杂度分析
- 时间复杂度: O(n)
- 空间复杂度: O(n)
6、递归(使用unordered_map)
思路
为了高效查找根节点元素在中序遍历数组中的下标,我们选择创建哈希表来存储中序序列,即建立一个(元素,下标)键值对的哈希表。
代码
class Solution {int post_idx;unordered_map<int, int> idx_map;
public:TreeNode* helper(int in_left, int in_right, vector<int>& inorder, vector<int>& postorder){// 如果这里没有节点构造二叉树了,就结束if (in_left > in_right) {return nullptr;}// 选择 post_idx 位置的元素作为当前子树根节点int root_val = postorder[post_idx];TreeNode* root = new TreeNode(root_val);// 根据 root 所在位置分成左右两棵子树int index = idx_map[root_val];// 下标减一post_idx--;// 构造右子树root->right = helper(index + 1, in_right, inorder, postorder);// 构造左子树root->left = helper(in_left, index - 1, inorder, postorder);return root;}TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {// 从后序遍历的最后一个元素开始post_idx = (int)postorder.size() - 1;// 建立(元素,下标)键值对的哈希表int idx = 0;for (auto& val : inorder) {idx_map[val] = idx++;}return helper(0, (int)inorder.size() - 1, inorder, postorder);}
};
复杂度分析
- 时间复杂度: O(n)
- 空间复杂度: O(n)
7、迭代
思路
为了高效查找根节点元素在中序遍历数组中的下标,我们选择创建哈希表来存储中序序列,即建立一个(元素,下标)键值对的哈希表。
代码
class Solution {
public:TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {// 如果后序遍历数组为空,则返回空指针if (postorder.size() == 0) {return nullptr;}// 创建根节点,其值为后序遍历数组的最后一个元素auto root = new TreeNode(postorder[postorder.size() - 1]);// 创建一个栈,用于存储节点指针auto s = stack<TreeNode*>();// 将根节点入栈s.push(root);// 初始化中序遍历数组的索引int inorderIndex = inorder.size() - 1;// 从后序遍历数组的倒数第二个元素开始遍历for (int i = int(postorder.size()) - 2; i >= 0; i--) {// 当前遍历的后序遍历数组的值int postorderVal = postorder[i];// 获取栈顶节点auto node = s.top();// 如果栈顶节点的值不等于中序遍历数组当前索引的值if (node->val != inorder[inorderIndex]) {// 将当前后序遍历数组的值作为右子节点,并创建新的节点node->right = new TreeNode(postorderVal);// 将右子节点入栈s.push(node->right);} else {// 当栈不为空且栈顶节点的值等于中序遍历数组当前索引的值时while (!s.empty() && s.top()->val == inorder[inorderIndex]) {// 弹出栈顶节点node = s.top();s.pop();// 中序遍历数组索引减一inorderIndex--;}// 将当前后序遍历数组的值作为左子节点,并创建新的节点node->left = new TreeNode(postorderVal);// 将左子节点入栈s.push(node->left);}}// 返回根节点return root;}
};
复杂度分析
- 时间复杂度: O(n)
- 空间复杂度: O(n)
相关文章:

LeetCode 106.从中序与后序遍历序列构造二叉树
LeetCode 106.从中序与后序遍历序列构造二叉树 1、题目 题目链接:106. 从中序与后序遍历序列构造二叉树 给定两个整数数组 inorder 和 postorder ,其中 inorder 是二叉树的中序遍历, postorder 是同一棵树的后序遍历,请你构造并…...
函数,动态编译代码的艺术)
Python中的compile()函数,动态编译代码的艺术
关注公众号【一点sir】,领取编程资料。 简介 在Python编程中,compile()函数是一个强大的工具,它允许开发者将字符串形式的Python代码动态编译成字节码。这为执行动态生成或从外部源接收的代码提供了极大的灵活性。这些字节码随后可以被Pytho…...

【考研数学】汤家凤“免单“数学题被吐槽‘太难’,老汤回应「怎么还有脸笑」,网友:这些题有毒!
我看了汤家凤老师出的几道题,实际上对于考研的同学来说,确实是送分题 第一个是三角函数变换中的万能公式;第二个e^x的泰勒展开公式;第三个是第一类重要极限。只要复习过,那基本上都能正常做出来。 至于汤家凤老师说「…...
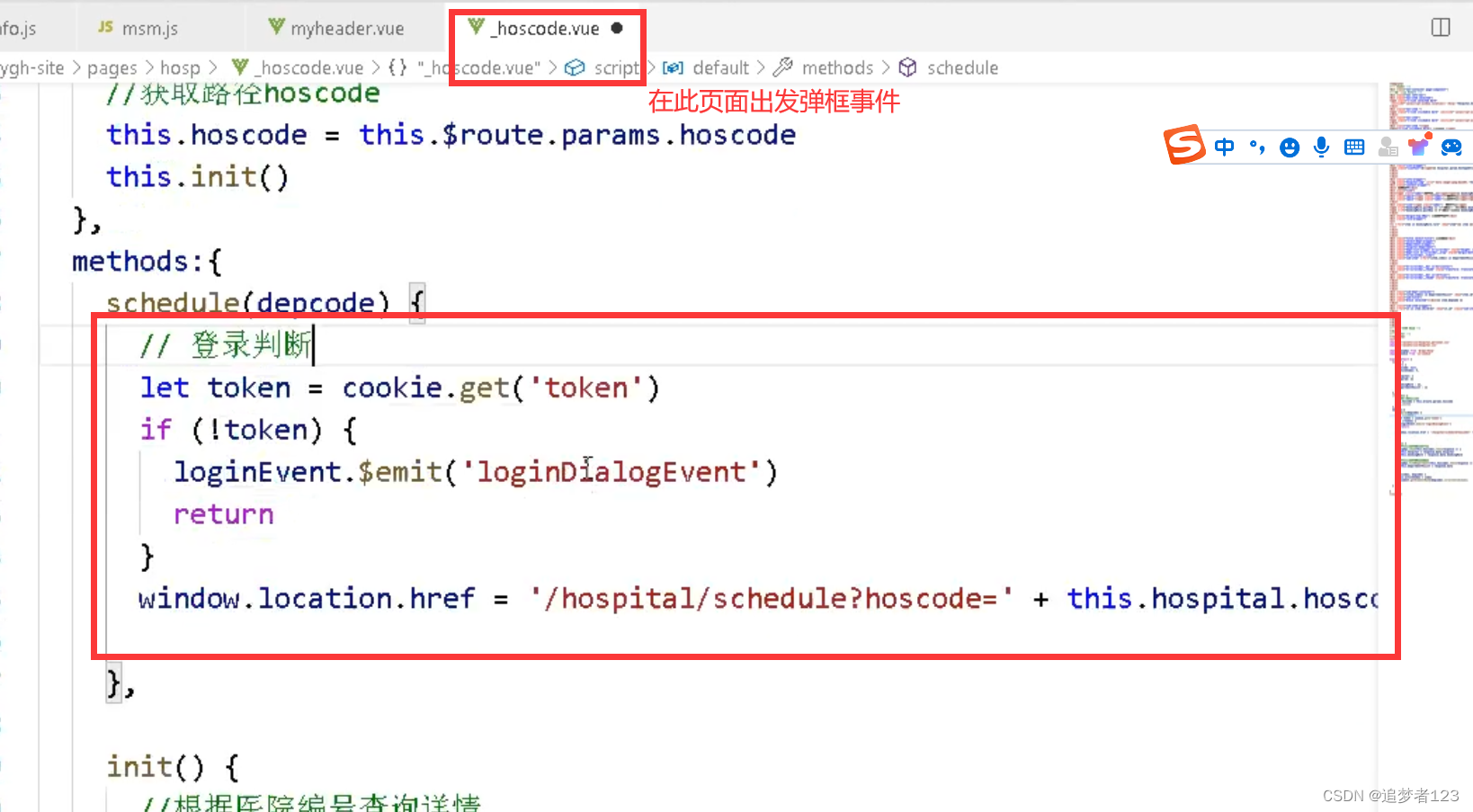
在另外一个页面,让另外一个页面弹框显示操作(调佣公共的弹框)
大概意思是,登录弹框在另外一个页面中,而当前页面不存在,在当前页面中判断如果token不存在,就弹框出登录的弹框 最后一行 window.location.href … 如果当前用户已登录,则执行后续操作(注意此处,可不要)...

如何利用IPIDEA代理IP优化数据采集效率?
一、 前言二、 IPIDEA介绍三、体验步骤四、实战训练五、结语 一、 前言 在全球化与信息化交织的当代社会,数据已成为驱动商业智慧与技术革新的核心引擎。网络,作为信息汇聚与交流的枢纽,不仅是人们获取知识的窗口,更是商业活动与技…...

Rpcx (一):详解【介绍、基础示例 demo】
一.rpcx介绍 1.1 rpc是什么 远程过程调用的通信协议。该协议允许运行于一台计算机的程序调用另一台计算机的子程序,而程序员无需额外地为这个交互作用编程。如果涉及的软件采用面向对象编程,那么远程过程调用亦可称作远程调用或远程方法调用。简单地说就是能使应用像调用本地…...
对数据进行标准化和归一化
数据的形式:保存在CSV中,第一列为姓名,第二列之后为特征。 标准化 输入文件的路径,设置保存转化后的文件路径 import pandas as pd from sklearn.preprocessing import StandardScaler# 读取CSV文件 data pd.read_csv(rC:\User…...
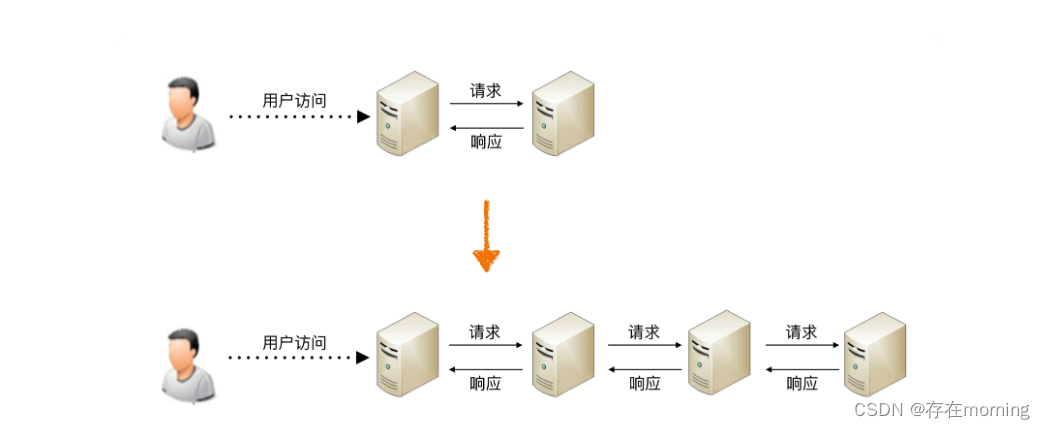
【从零开始学架构 架构基础】二 架构设计的复杂度来源:高性能复杂度来源
架构设计的复杂度来源其实就是架构设计要解决的问题,主要有如下几个:高性能、高可用、可扩展、低成本、安全、规模。复杂度的关键,就是新旧技术之间不是完全的替代关系,有交叉,有各自的特点,所以才需要具体…...

OpenHarmony 实战开发——3.1 Release + Linux 原厂内核Launcher起不来问题分析报告
1、关键字 Launcher 无法启动;原厂内核;Access Token ID; 2、问题描述 芯片:rk3566;rk3399 内核版本:Linux 4.19,是 RK 芯片原厂发布的 rk356x 4.19 稳定版内核 OH 版本:OpenHa…...
小猫咪邮件在线发送系统源码,支持添加附件
一款免登录发送邮件,支持发送附件,后台可添加邮箱,前台可选择发送邮箱 网站数据采取本地保存,所以使用前请给网站修改权限,否则很多功能将无法使用 安装教程: 1.上传服务器或者主机 2.登录后台,添加发送…...
是什么?)
Django REST framework(DRF)是什么?
Django REST framework(DRF)是什么? Django REST framework(简称DRF)是一个强大且灵活的工具包,用于构建Web API。它是基于Django(一个高级Python Web框架)构建的,提供了…...

用hMailServer+roundcubemail+宝塔安装配置一个自己的邮箱服务
用hMailServerroundcubemail安装配置一个自己的邮箱服务 1、准备工具与资料: 云服务器一台 基础配置就行 2核4G。域名一个 以下用lizipro.cn示例。hMailServer安装包roundcubemail安装包异常处理插件补丁: libmysql.zip 2、hMailServer服务安装&#…...

ctfshow 框架复现
文章目录 web 466web 467web 468web469web 470web 471web 472web 473web 474web 475web 476 web 466 Laravel5.4版本 ,提交数据需要base64编码 代码审计学习—Laravel5.4 - 先知社区 (aliyun.com) 用第二条链子 反序列化格式 /admin/序列化串base64<?php na…...
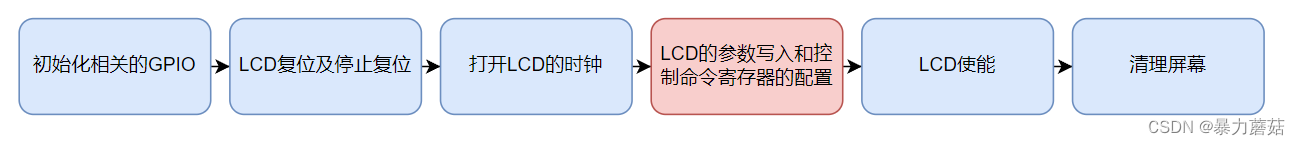
【Linux-IMX6ULL-DDR3简介测试-RGBLCD控制原理】
目录 1. DDR3 简介1.1 前要基本概念RAM & ROM 2. DDR3测试及初始化3. RGBLCD简介及控制原理3.1 RGBLCD简介3.2.1 RGB LCD时序3.2.2 像素时钟(800*400分辨率)3.2.2 显存(800*400分辨率) 3.3 RGBLCD的控制3.3.1 DOTCLK 硬件接口…...

贪心算法-----柠檬水找零
今日题目:leetcode860 题目链接:点击跳转题目 分析: 顾客只会给三种面值:5、10、20,先分类讨论 当收到5美元时:不用找零,面值5张数1当收到10美元时:找零5美元,面值5张数…...

MySQL技能树学习
在MySQL中,DDL(数据定义语言)用于定义数据库对象(如表、索引、视图等),DML(数据操纵语言)用于操作数据库中的数据(如插入、更新、删除数据),DQL&a…...

java 动态代理详解
cglib 动态代理 介绍 CGLIB是一个功能强大,高性能的代码生成包。它为没有实现接口的类提供代理,为JDK的动态代理提供了很好的补充。通常可以使用Java的动态代理创建代理,但当要代理的类没有实现接口或者为了更好的性能,CGLIB 是一…...

Web路径专题
文章目录 Web路径专题什么是路径?绝对路径相对路径 如何使用路径?使用base标签 注意事项小结 Web路径专题 在Web开发中,路径是一个非常重要的概念。路径用来定位资源的位置,包括文件、目录、网页等。在本文中,我们将介…...

解决vue3项目打包后部署后某些静态资源图片不加载问题
目录 问题 原因 解决方案 问题 开发完项目打包并部署 然后访问时发现导航栏背景图片没加载 打开浏览器控制台发现这张图片报错404 原因 可能是因为在部署后的服务器环境中对中文文件名的支持不完善。服务器在解析 URL 时可能无法正确识别或编码中文字符,导致无…...

传感网应用开发教程--AT指令访问新大陆云平台(ESP8266模块+物联网云+TCP)
实现目标 1、熟悉AT指令 2、熟悉新大陆云平台新建项目 3、具体目标:(1)注册新大陆云平台;(2)新建一个联网方案为WIFI的项目;(3)ESP8266模块,通过AT指令访问…...
)
IGP(Interior Gateway Protocol,内部网关协议)
IGP(Interior Gateway Protocol,内部网关协议) 是一种用于在一个自治系统(AS)内部传递路由信息的路由协议,主要用于在一个组织或机构的内部网络中决定数据包的最佳路径。与用于自治系统之间通信的 EGP&…...
【机器视觉】单目测距——运动结构恢复
ps:图是随便找的,为了凑个封面 前言 在前面对光流法进行进一步改进,希望将2D光流推广至3D场景流时,发现2D转3D过程中存在尺度歧义问题,需要补全摄像头拍摄图像中缺失的深度信息,否则解空间不收敛…...

鸿蒙中用HarmonyOS SDK应用服务 HarmonyOS5开发一个医院查看报告小程序
一、开发环境准备 工具安装: 下载安装DevEco Studio 4.0(支持HarmonyOS 5)配置HarmonyOS SDK 5.0确保Node.js版本≥14 项目初始化: ohpm init harmony/hospital-report-app 二、核心功能模块实现 1. 报告列表…...

基于matlab策略迭代和值迭代法的动态规划
经典的基于策略迭代和值迭代法的动态规划matlab代码,实现机器人的最优运输 Dynamic-Programming-master/Environment.pdf , 104724 Dynamic-Programming-master/README.md , 506 Dynamic-Programming-master/generalizedPolicyIteration.m , 1970 Dynamic-Programm…...

laravel8+vue3.0+element-plus搭建方法
创建 laravel8 项目 composer create-project --prefer-dist laravel/laravel laravel8 8.* 安装 laravel/ui composer require laravel/ui 修改 package.json 文件 "devDependencies": {"vue/compiler-sfc": "^3.0.7","axios": …...
sipsak:SIP瑞士军刀!全参数详细教程!Kali Linux教程!
简介 sipsak 是一个面向会话初始协议 (SIP) 应用程序开发人员和管理员的小型命令行工具。它可以用于对 SIP 应用程序和设备进行一些简单的测试。 sipsak 是一款 SIP 压力和诊断实用程序。它通过 sip-uri 向服务器发送 SIP 请求,并检查收到的响应。它以以下模式之一…...

代码随想录刷题day30
1、零钱兑换II 给你一个整数数组 coins 表示不同面额的硬币,另给一个整数 amount 表示总金额。 请你计算并返回可以凑成总金额的硬币组合数。如果任何硬币组合都无法凑出总金额,返回 0 。 假设每一种面额的硬币有无限个。 题目数据保证结果符合 32 位带…...
push [特殊字符] present
push 🆚 present 前言present和dismiss特点代码演示 push和pop特点代码演示 前言 在 iOS 开发中,push 和 present 是两种不同的视图控制器切换方式,它们有着显著的区别。 present和dismiss 特点 在当前控制器上方新建视图层级需要手动调用…...

Redis:现代应用开发的高效内存数据存储利器
一、Redis的起源与发展 Redis最初由意大利程序员Salvatore Sanfilippo在2009年开发,其初衷是为了满足他自己的一个项目需求,即需要一个高性能的键值存储系统来解决传统数据库在高并发场景下的性能瓶颈。随着项目的开源,Redis凭借其简单易用、…...
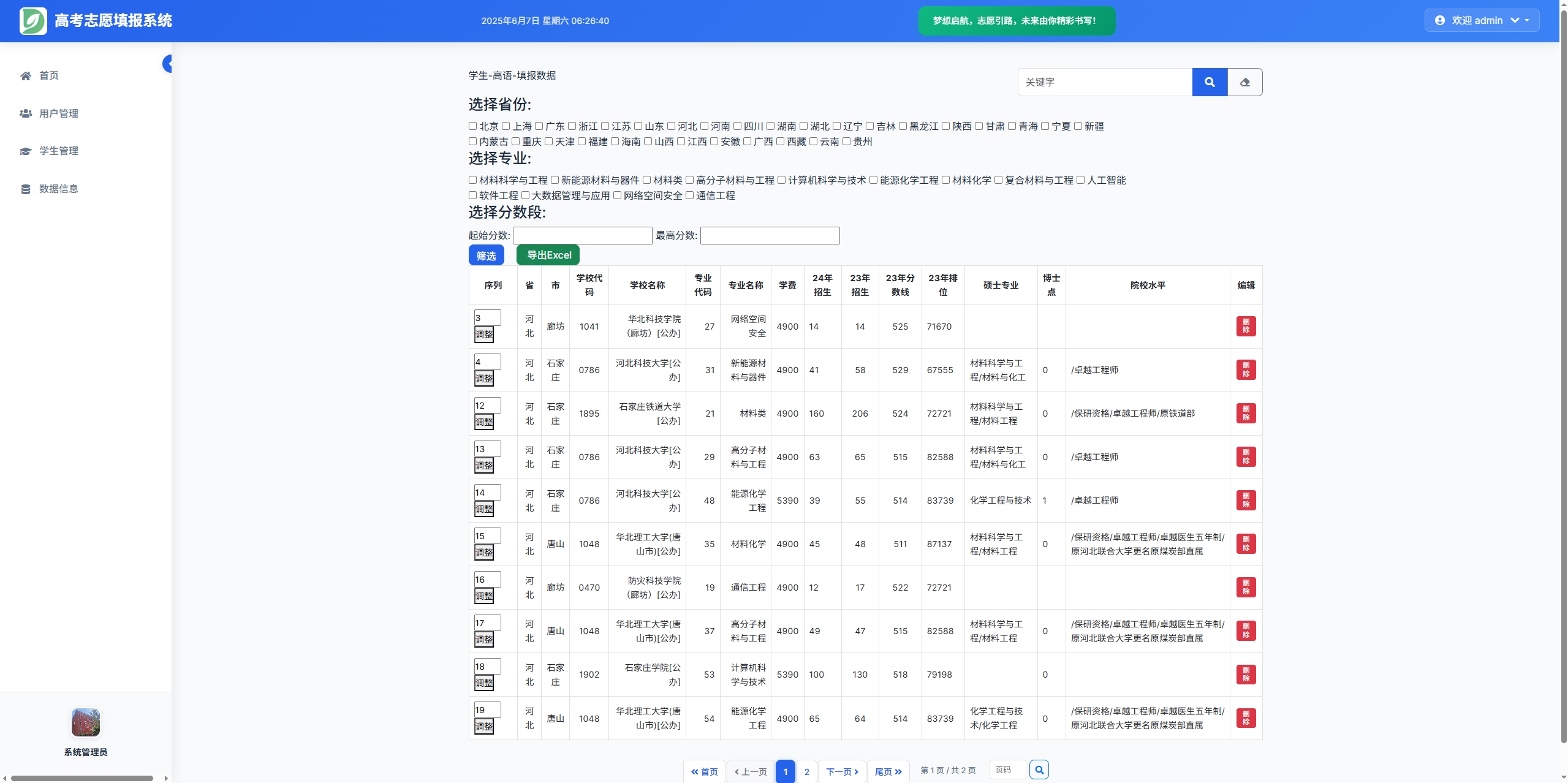
高考志愿填报管理系统---开发介绍
高考志愿填报管理系统是一款专为教育机构、学校和教师设计的学生信息管理和志愿填报辅助平台。系统基于Django框架开发,采用现代化的Web技术,为教育工作者提供高效、安全、便捷的学生管理解决方案。 ## 📋 系统概述 ### 🎯 系统定…...
