python 不相交集简介(并查集算法)【Introduction to Disjoint Set (Union-Find Algorithm)】

什么是不相交集数据结构?
如果两个集合没有任何共同元素,则它们被称为不相交集,集合的交集为空集。
存储不重叠或不相交元素子集的数据结构称为不相交集合数据结构。不相交集合数据结构支持以下操作:
1、将新集合添加到不相交集合中。
2、使用联合操作将不相交集合并为单个不相交集。
3、使用“查找”操作查找不相交集的代表。
4、检查两个集合是否不相交。
考虑这样一个情况,有许多人需要执行以下任务:
1、添加新的友谊关系,即一个人 x 成为另一个人 y 的朋友,即向集合中添加新元素。
2、判断个体x 是否是个体 y 的朋友(直接或间接朋友)
例子:
我们有 10 个人,比如 a、b、c、d、e、f、g、h、i、j
以下是需要添加的关系:
a <-> b
b <-> d
c <-> f
c <-> i
j <-> e
g <-> j
给定查询,例如 a 是否是 d 的朋友。我们基本上需要创建以下 4 个组,并在组项之间保持快速访问的连接:
G1 = {a, b, d}
G2 = {c, f, i}
G3 = {e, g, j}
G4 = {h}
判断 x 和 y 是否属于同一组,即判断 x 和 y 是否是直接/间接朋友。
根据个体所属的组别,将个体划分为不同的集合。此方法称为不相交集合并集,它维护不相交集合的集合,每个集合由其成员之一表示。
要回答上述问题,需要考虑两个关键点:
1、如何解析集合?最初,所有元素都属于不同的集合。在处理给定的关系后,我们选择一个成员作为代表。选择代表的方法有很多种,一种简单的方法是选择最大的索引。
2、检查两个人是否在同一组中?如果两个人的代表相同,那么他们就会成为朋友。
使用的数据结构包括:
数组:整数数组称为Parent[]。如果我们处理N 个项目,则数组的第 i 个元素代表第 i 个项目。更准确地说,Parent[] 数组的第 i 个元素是第 i 个项目的父级。这些关系创建一个或多个虚拟树。
树:它是一个不相交集。如果两个元素在同一棵树中,那么它们就在同一个不相交集。每棵树的根节点(或最顶端节点)称为集合的代表。每个集合始终有一个唯一的代表。识别代表的一个简单规则是,如果“i”是集合的代表,则Parent[i] = i。如果 i 不是其集合的代表,则可以通过沿树向上移动直到找到代表来找到它。
不相交集合数据结构上的操作:
查找
联合
1. 查找:
可以通过递归遍历父数组直到找到其自身的父节点来实现。
# Finds the representative of the set
# that i is an element of
def find(i):
# If i is the parent of itself
if (parent[i] == i):
# Then i is the representative of
# this set
return i
else:
# Else if i is not the parent of
# itself, then i is not the
# representative of his set. So we
# recursively call Find on its parent
return find(parent[i])
# The code is contributed by Nidhi goel
时间复杂度:这种方法效率低下,在最坏的情况下可能需要 O(n) 时间。
2. 联合:
它以两个元素作为输入,并使用查找操作找到它们的集合的代表,最后将其中一棵树(代表集合)放在另一棵树的根节点下。
# Unites the set that includes i
# and the set that includes j
def union(parent, rank, i, j):
# Find the representatives
# (or the root nodes) for the set
# that includes i
irep = find(parent, i)
# And do the same for the set
# that includes j
jrep = find(parent, j)
# Make the parent of i’s representative
# be j’s representative effectively
# moving all of i’s set into j’s set)
parent[irep] = jrep
时间复杂度:这种方法效率低下,在最坏的情况下可能导致长度为 O(n)的树。
优化(按等级/大小合并和路径压缩):
效率在很大程度上取决于哪棵树连接到另一棵树。有两种方法可以实现。第一种是按等级联合,它将树的高度视为一个因素;第二种是按大小联合,它将树的大小视为一个因素,同时将一棵树连接到另一棵树。此方法与路径压缩一起提供了几乎恒定时间的复杂性。
路径压缩(对 Find() 的修改):
它通过压缩树的高度来加速数据结构。这可以通过在Find操作中插入一个小的缓存机制来实现。查看代码了解更多详细信息:
# Finds the representative of the set that i
# is an element of.
def find(i):
# If i is the parent of itself
if Parent[i] == i:
# Then i is the representative
return i
else:
# Recursively find the representative.
result = find(Parent[i])
# We cache the result by moving i’s node
# directly under the representative of this
# set
Parent[i] = result
# And then we return the result
return result
# The code is contributed by Arushi Jindal.
时间复杂度:平均每次调用为 O(log n)。
按等级合并:
首先,我们需要一个新的整数数组,名为rank[] 。此数组的大小与父数组Parent[]相同。如果 i 代表一个集合,则rank[i]就是代表该集合的树的高度。 现在回想一下,在 Union 操作中,将两棵树中的哪一棵移动到另一棵之下并不重要。现在我们要做的是最小化结果树的高度。如果我们要合并两棵树(或集合),我们将它们称为左和右,那么这一切都取决于左的等级和右的等级。
1、如果左边的等级小于右边的等级,那么最好将左边移到右边的下方,因为这不会改变右边的等级(而将右边移到左边的下方会增加高度)。同样,如果右边的等级小于左边的等级,那么我们应该将右边移到左边的下方。
2、如果等级相等,那么哪棵树位于另一棵树之下并不重要,但结果的等级始终比树的等级大一。
class DisjointSet:
def __init__(self, size):
self.parent = [i for i in range(size)]
self.rank = [0] * size
# Function to find the representative (or the root node) of a set
def find(self, i):
# If i is not the representative of its set, recursively find the representative
if self.parent[i] != i:
self.parent[i] = self.find(self.parent[i]) # Path compression
return self.parent[i]
# Unites the set that includes i and the set that includes j by rank
def union_by_rank(self, i, j):
# Find the representatives (or the root nodes) for the set that includes i and j
irep = self.find(i)
jrep = self.find(j)
# Elements are in the same set, no need to unite anything
if irep == jrep:
return
# Get the rank of i's tree
irank = self.rank[irep]
# Get the rank of j's tree
jrank = self.rank[jrep]
# If i's rank is less than j's rank
if irank < jrank:
# Move i under j
self.parent[irep] = jrep
# Else if j's rank is less than i's rank
elif jrank < irank:
# Move j under i
self.parent[jrep] = irep
# Else if their ranks are the same
else:
# Move i under j (doesn't matter which one goes where)
self.parent[irep] = jrep
# Increment the result tree's rank by 1
self.rank[jrep] += 1
def main(self):
# Example usage
size = 5
ds = DisjointSet(size)
# Perform some union operations
ds.union_by_rank(0, 1)
ds.union_by_rank(2, 3)
ds.union_by_rank(1, 3)
# Find the representative of each element
for i in range(size):
print(f"Element {i} belongs to the set with representative {ds.find(i)}")
# Creating an instance and calling the main method
ds = DisjointSet(size=5)
ds.main()
按大小合并:
同样,我们需要一个新的整数数组,名为size[] 。此数组的大小与父数组Parent[]相同。如果 i 代表一个集合,则size[i]是代表该集合的树中元素的数量。 现在我们将两棵树(或集合)合并起来,我们将它们称为左树和右树,在这种情况下,一切都取决于左树(或集合)的大小和右树(或集合)的大小。
1、如果左边的尺寸小于右边的尺寸,那么最好将左边移到右边下方,并将右边的尺寸增加左边的尺寸。同样,如果右边的尺寸小于左边的尺寸,那么我们应该将右边移到左边下方,并将左边的尺寸增加右边的尺寸。
2、如果尺寸相等,那么哪棵树位于另一棵树下都没有关系。
# Python program for the above approach
class UnionFind:
def __init__(self, n):
# Initialize Parent array
self.Parent = list(range(n))
# Initialize Size array with 1s
self.Size = [1] * n
# Function to find the representative (or the root node) for the set that includes i
def find(self, i):
if self.Parent[i] != i:
# Path compression: Make the parent of i the root of the set
self.Parent[i] = self.find(self.Parent[i])
return self.Parent[i]
# Unites the set that includes i and the set that includes j by size
def unionBySize(self, i, j):
# Find the representatives (or the root nodes) for the set that includes i
irep = self.find(i)
# And do the same for the set that includes j
jrep = self.find(j)
# Elements are in the same set, no need to unite anything.
if irep == jrep:
return
# Get the size of i’s tree
isize = self.Size[irep]
# Get the size of j’s tree
jsize = self.Size[jrep]
# If i’s size is less than j’s size
if isize < jsize:
# Then move i under j
self.Parent[irep] = jrep
# Increment j's size by i's size
self.Size[jrep] += self.Size[irep]
# Else if j’s size is less than i’s size
else:
# Then move j under i
self.Parent[jrep] = irep
# Increment i's size by j's size
self.Size[irep] += self.Size[jrep]
# Example usage
n = 5
unionFind = UnionFind(n)
# Perform union operations
unionFind.unionBySize(0, 1)
unionFind.unionBySize(2, 3)
unionFind.unionBySize(0, 4)
# Print the representative of each element after unions
for i in range(n):
print("Element {}: Representative = {}".format(i, unionFind.find(i)))
# This code is contributed by Susobhan Akhuli
输出
元素 0:代表 = 0
元素 1:代表 = 0
元素 2:代表 = 2
元素 3:代表 = 2
元素 4:代表 = 0
时间复杂度:O(log n),无路径压缩。
下面是具有路径压缩和按等级合并的不相交集的完整实现。
# Python3 program to implement Disjoint Set Data
# Structure.
class DisjSet:
def __init__(self, n):
# Constructor to create and
# initialize sets of n items
self.rank = [1] * n
self.parent = [i for i in range(n)]
# Finds set of given item x
def find(self, x):
# Finds the representative of the set
# that x is an element of
if (self.parent[x] != x):
# if x is not the parent of itself
# Then x is not the representative of
# its set,
self.parent[x] = self.find(self.parent[x])
# so we recursively call Find on its parent
# and move i's node directly under the
# representative of this set
return self.parent[x]
# Do union of two sets represented
# by x and y.
def Union(self, x, y):
# Find current sets of x and y
xset = self.find(x)
yset = self.find(y)
# If they are already in same set
if xset == yset:
return
# Put smaller ranked item under
# bigger ranked item if ranks are
# different
if self.rank[xset] < self.rank[yset]:
self.parent[xset] = yset
elif self.rank[xset] > self.rank[yset]:
self.parent[yset] = xset
# If ranks are same, then move y under
# x (doesn't matter which one goes where)
# and increment rank of x's tree
else:
self.parent[yset] = xset
self.rank[xset] = self.rank[xset] + 1
# Driver code
obj = DisjSet(5)
obj.Union(0, 2)
obj.Union(4, 2)
obj.Union(3, 1)
if obj.find(4) == obj.find(0):
print('Yes')
else:
print('No')
if obj.find(1) == obj.find(0):
print('Yes')
else:
print('No')
# This code is contributed by ng24_7.
输出
Yes
No
时间复杂度:创建 n 个单项集的时间为 O(n)。两种技术(路径压缩和按等级/大小合并)的时间复杂度将达到接近常数时间。事实证明,最终的 摊销时间复杂度为 O(α(n)),其中 α(n) 是逆阿克曼函数,其增长非常稳定(当 n<10 600 ![]() 时,它甚至不会超过)。
时,它甚至不会超过)。
空间复杂度: O(n),因为我们需要在不相交集数据结构中存储 n 个元素。
相关文章:
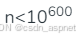
python 不相交集简介(并查集算法)【Introduction to Disjoint Set (Union-Find Algorithm)】
什么是不相交集数据结构? 如果两个集合没有任何共同元素,则它们被称为不相交集,集合的交集为空集。 存储不重叠或不相交元素子集的数据结构称为不相交集合数据结构。不相交集合数据结构支持以下操作: 1、将新集合添加到不相交集合…...
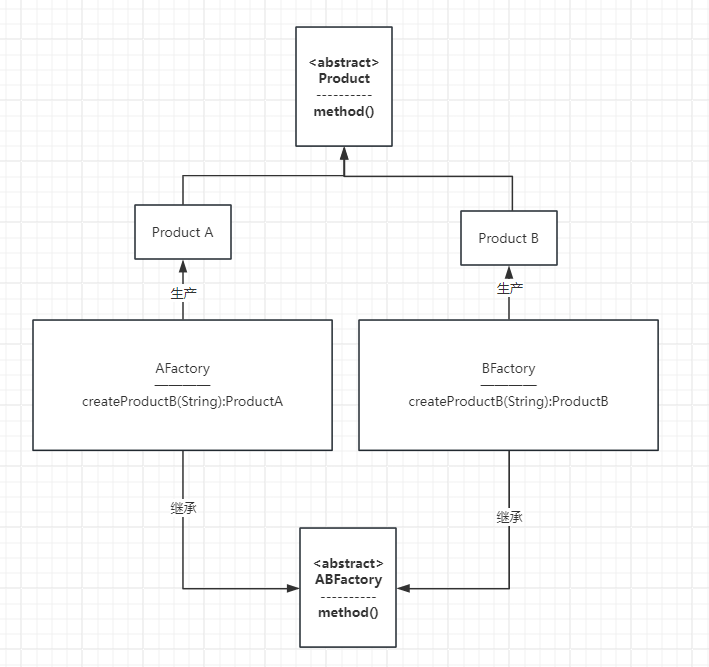
23种设计模式之工厂方法模式
文章目录 1. 简介2. 代码2.1 抽象类:Course.java2.2 产品A:JavaCourse.java2.3 产品B:PythonCourse.java2.4 工厂抽象类:CourseFactory.java2.5 产品A的工厂A:JavaCourseFactory.java2.6 产品B的工厂B:PyCo…...
Redis——事务
文章目录 Redis 事务Redis 的事务和 MySQL 事务的区别:事务操作MULTIEXECDISCARDWATCHUNWATCHwatch的实现原理 总结 Redis 事务 什么是事务 Redis 的事务和 MySQL 的事务 概念上是类似的. 都是把⼀系列操作绑定成⼀组. 让这⼀组能够批量执行 Redis 的事务和 MySQL 事务的区别:…...

Redis非关系型数据库操作命令大全
以下是 Redis 的常用操作命令大全,涵盖了键值操作、字符串、哈希、列表、集合、有序集合、发布/订阅、事务等多个方面的操作。 1. 通用键命令 命令说明SET key value设置指定 key 的值GET key获取指定 key 的值DEL key删除指定的 keyEXISTS key检查 key 是否存在E…...

基于SpringBoot+Vue+uniapp微信小程序的澡堂预订的微信小程序的详细设计和实现
项目运行截图 技术框架 后端采用SpringBoot框架 Spring Boot 是一个用于快速开发基于 Spring 框架的应用程序的开源框架。它采用约定大于配置的理念,提供了一套默认的配置,让开发者可以更专注于业务逻辑而不是配置文件。Spring Boot 通过自动化配置和约…...
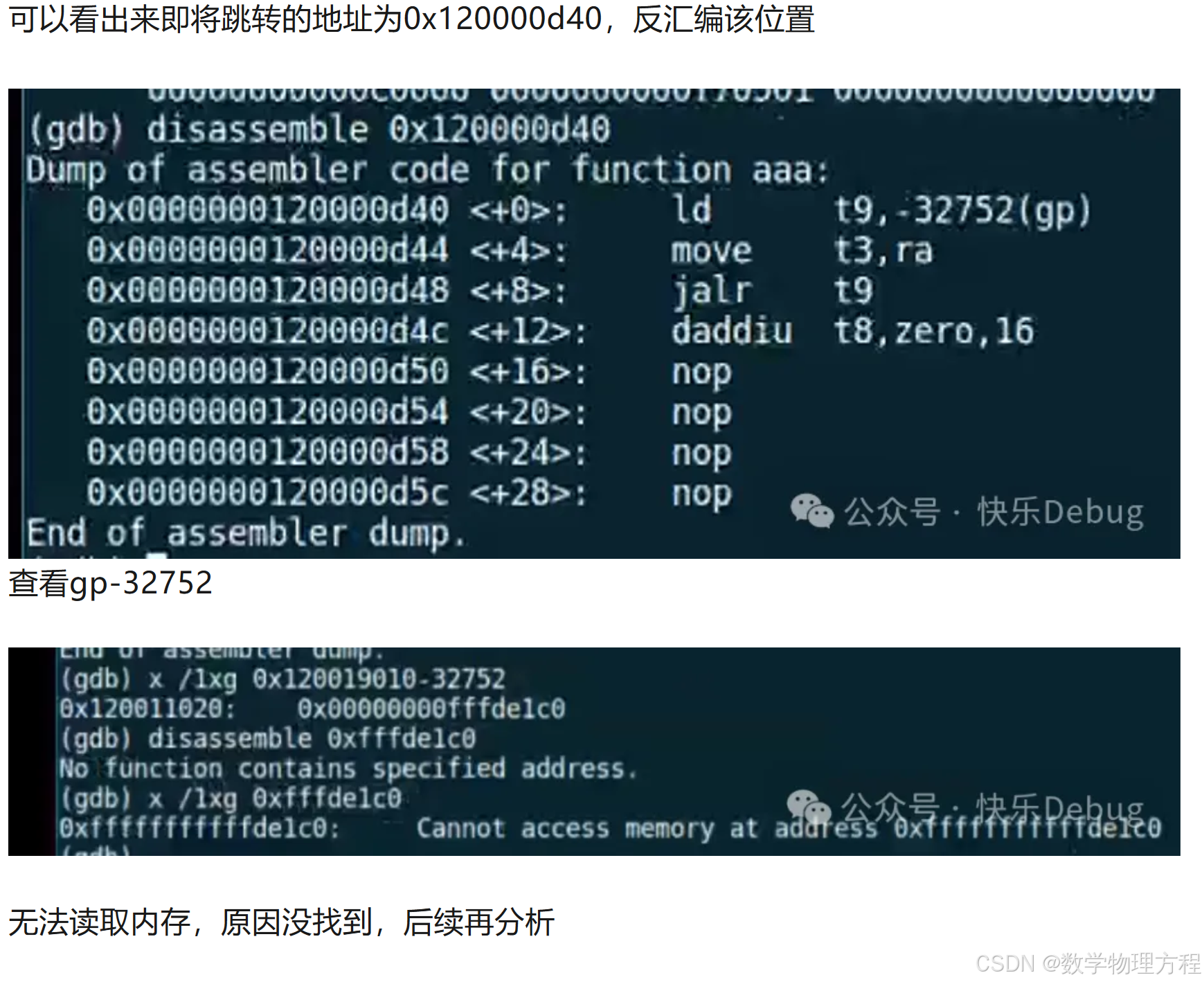
Linux mips架构链接库函数调用plt表汇编代码分析
linux调用共享库中的函数时通过plt表和got表实现位置无关代码,过程中涉及到lazy binding,即在第一调用外部函数时解析被调用的函数地址并将地址写入到got表,后续调用则不需要解析函数地址。这一部分和硬件架构有关,具体的是和cpu指…...
python 作业1
任务1: python为主的工作是很少的 学习的python的优势在于制作工具,制作合适的工具可以提高我们在工作中的工作效率的工具 提高我们的竞争优势。 任务2: 不换行 换行 任务3: 安装pycharm 进入相应网站Download PyCharm: The Python IDE for data science and we…...

Apache 出现 “403 forbidden“ 排查方法
1、检查运行 Apache 进程的用户没有对目录具备读取权限 如果该用户没有对 Directory 指定的目录具备适当的读取权限,就会导致 403 错误。 例如:使用用户apache启动Apache进程,但是apache用户对 Directory 指定的目录没有读取权限 2、检查…...

vue video播放m3u8监控视频
很关键的问题 vite创建的项目不需要import ‘videojs-contrib-hls’ 导入就报错 直接添加如下代码即可 html5: {vhs: {overrideNative: true},nativeVideoTracks: false,nativeAudioTracks: false,nativeTextTracks: false} 下面是完整组件示例 <template><div>…...

uniapp 获取签名证书 SHA1 自有证书签名打包
1.登录你的Dcloud 账户 2.找到我的应用菜单 3.点开某个应用 4.查看证书详情,里面有SHA1 和别名,密码,下载证书用于云打包,可以选择自有证书,输入别名,密码打包...
)
Open3d开发点云标注工具问题总结(二)
前面我们介绍了使用AABB方式来框选点云,但这种方式还是不够直观,我们的构想是设计一个和o3d.visualization.VisualizerWithEditing的点云框选方法一样的软件,因此,博主想到利用投影的形式进行解决: 具体的,…...
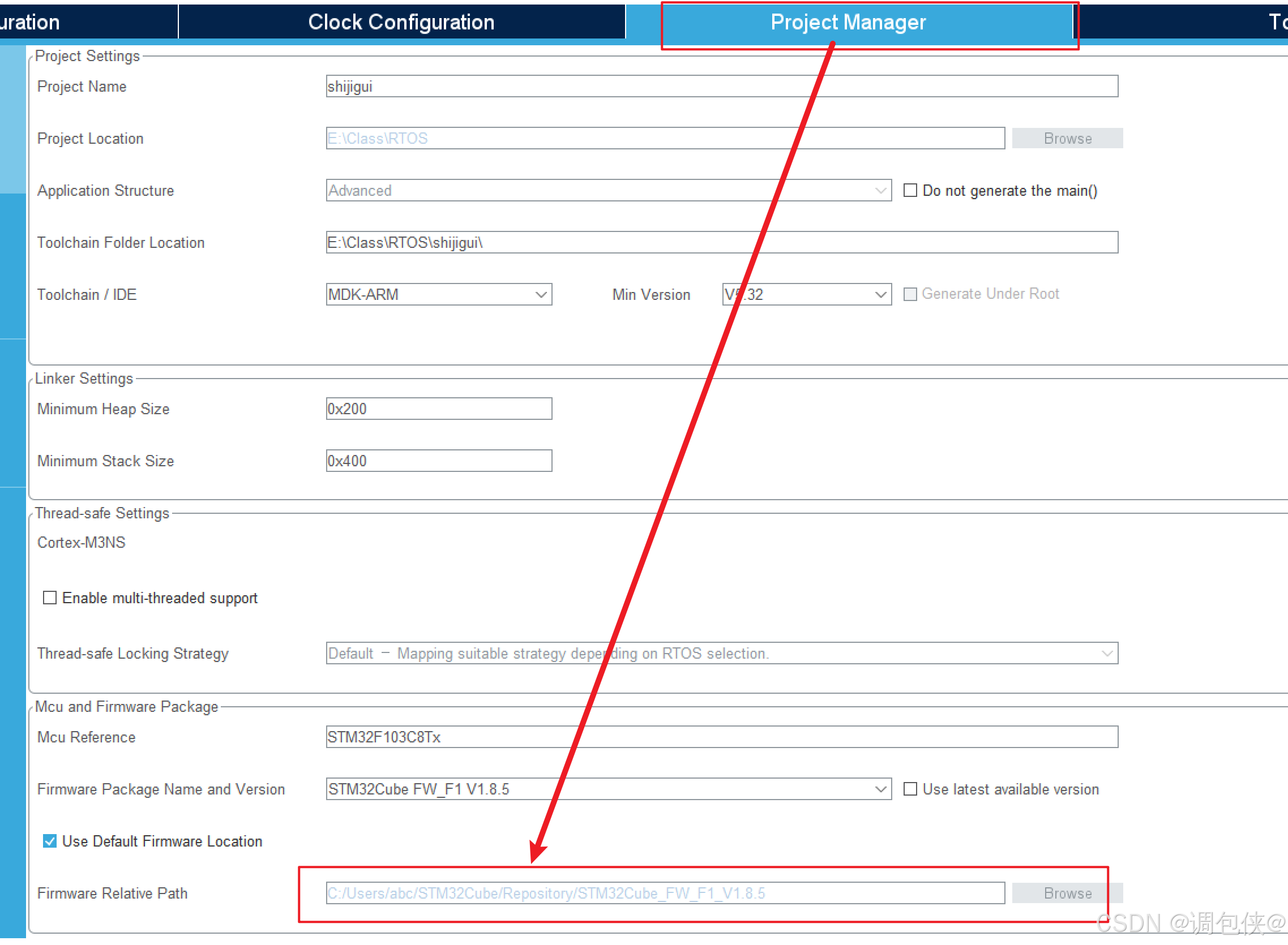
【FreeRTOS】
报错: 使用STM32cubemx自动生成freertos选项V2报错,V1不报错 …/Middlewares/Third_Party/FreeRTOS/Source/CMSIS_RTOS_V2/freertos_os2.h(31): 解决 修改cubemx配置,将V1.8.6改选为V1.8.5后编译不再报错...

洛谷 P4995:跳跳! ← 贪心算法
【题目来源】https://www.luogu.com.cn/problem/P4995【题目描述】你是一只小跳蛙,你特别擅长在各种地方跳来跳去。 这一天,你和朋友小 F 一起出去玩耍的时候,遇到了一堆高矮不同的石头,其中第 i 块的石头高度为 hi,地…...

代理 IP 在 AI 爬虫中的关键应用
现如今,人工智能(AI)的发展日新月异,而数据作为驱动 AI 发展的关键要素,其重要性不言而喻。AI 爬虫作为获取大量数据的重要工具,在数据收集过程中发挥着至关重要的作用。而代理 IP 在 AI 爬虫中有着广泛而重…...
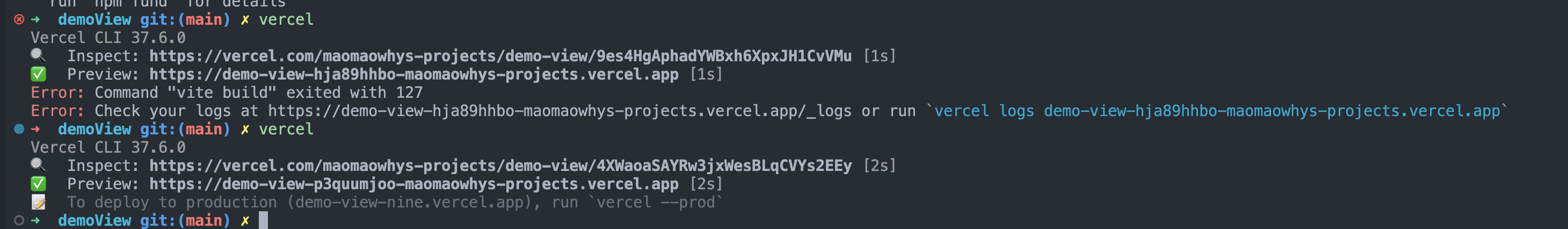
【Vercel】Vercel静态部署踩坑
背景 在现代的软件开发中,自动化部署是一个不可或缺的环节。Vercel作为一个流行的前端部署平台,提供了与GitHub的无缝集成,使得开发者能够在每次提交代码后自动触发部署流程。然而,自动化部署过程中可能会遇到一些挑战࿰…...
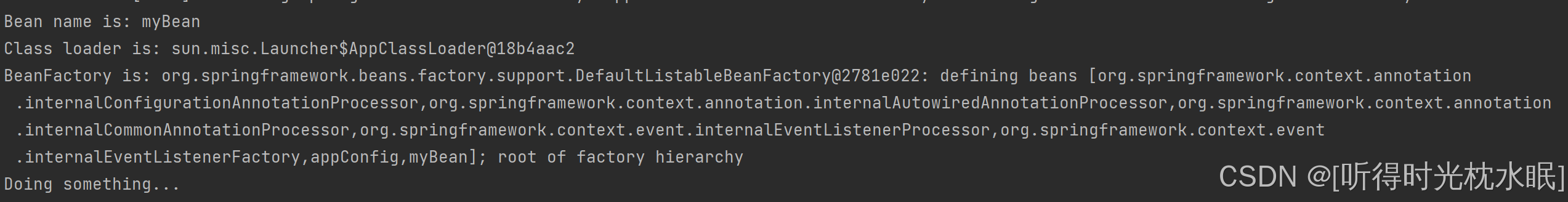
【Spring】关于Spring中aware相关接口的作用
Aware 接口的回调方法是在 Bean 实例化之后调用的。具体来说,这些回调方法是在依赖注入完成后,但在 Bean 完全初始化之前调用的。这是 Spring 容器管理 Bean 生命周期的一部分 完成了属性赋值之后,Spring会执行一些回调,包括&…...
动态内存管理及RAII的简单应用
目录 一.程序启动所关联的内存分区 二.动态内存的申请和释放 三.将RAII思想融入代码 四.RAII思想的简单应用 一.程序启动所关联的内存分区 .dll文件是Dynamic Link Library(动态链接库)文件的缩写,它是一种共享库文件,包含…...
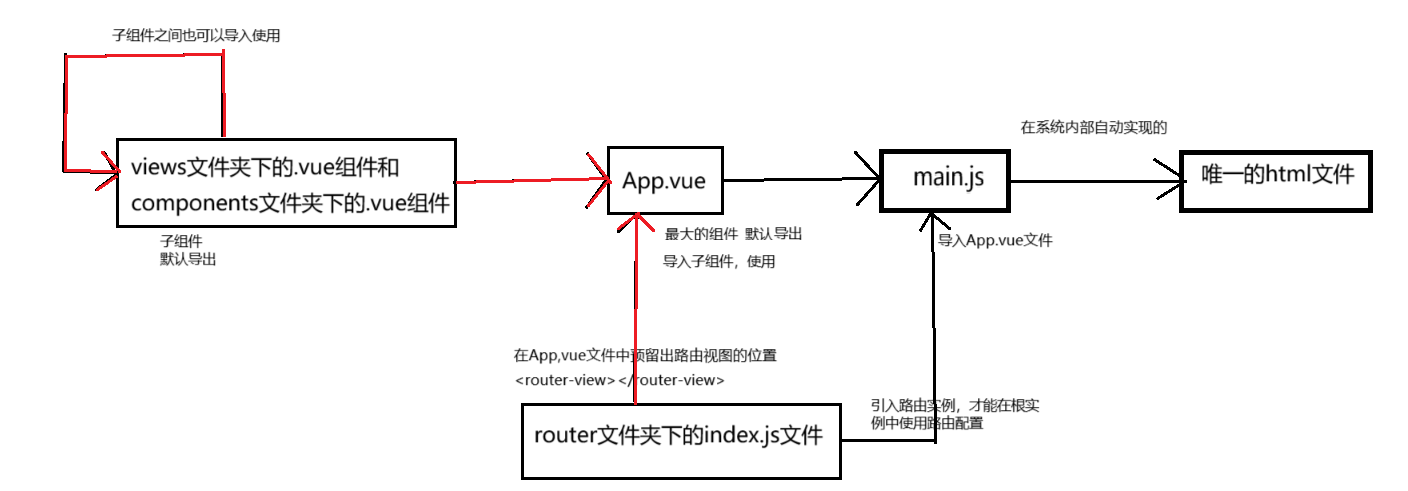
7、Vue2(一)
1.认识Vue 官网地址:https://v2.cn.vuejs.org/v2/guide/ Vue.js 是一套构建用户界面的渐进式框架。 Vue 2 是在2016年发布使用,2020是 vue3 才刚发布,时隔一年左右就已经将 vue3 作为了默认版本 尤雨溪,Vue.js和Vite的作者&…...

Chapter11
11.3 #include <stdio.h> #include <string.h> #define NUM_STUDENTS 40 #define NUM_SUBJECTS 3 // 学生结构体 typedef struct { int id; char name[50]; float scores[NUM_SUBJECTS]; float average; } Student; void inputData(Student studen…...
-----预训练)
LLAMA2入门(一)-----预训练
Llama 2 是预训练和微调的LLM系列,Llama 2 和 Llama 2-Chat 模型的参数规模达到 70B。Llama 2-Chat 模型专门为对话场景进行了优化。 这是一个系列的文章,会分别从LLAMA2的预训练,微调,安全性等方面进行讲解。 1.数据来源 数据…...

Docker 离线安装指南
参考文章 1、确认操作系统类型及内核版本 Docker依赖于Linux内核的一些特性,不同版本的Docker对内核版本有不同要求。例如,Docker 17.06及之后的版本通常需要Linux内核3.10及以上版本,Docker17.09及更高版本对应Linux内核4.9.x及更高版本。…...

C++:std::is_convertible
C++标志库中提供is_convertible,可以测试一种类型是否可以转换为另一只类型: template <class From, class To> struct is_convertible; 使用举例: #include <iostream> #include <string>using namespace std;struct A { }; struct B : A { };int main…...

基于ASP.NET+ SQL Server实现(Web)医院信息管理系统
医院信息管理系统 1. 课程设计内容 在 visual studio 2017 平台上,开发一个“医院信息管理系统”Web 程序。 2. 课程设计目的 综合运用 c#.net 知识,在 vs 2017 平台上,进行 ASP.NET 应用程序和简易网站的开发;初步熟悉开发一…...

反射获取方法和属性
Java反射获取方法 在Java中,反射(Reflection)是一种强大的机制,允许程序在运行时访问和操作类的内部属性和方法。通过反射,可以动态地创建对象、调用方法、改变属性值,这在很多Java框架中如Spring和Hiberna…...
selenium学习实战【Python爬虫】
selenium学习实战【Python爬虫】 文章目录 selenium学习实战【Python爬虫】一、声明二、学习目标三、安装依赖3.1 安装selenium库3.2 安装浏览器驱动3.2.1 查看Edge版本3.2.2 驱动安装 四、代码讲解4.1 配置浏览器4.2 加载更多4.3 寻找内容4.4 完整代码 五、报告文件爬取5.1 提…...

学校时钟系统,标准考场时钟系统,AI亮相2025高考,赛思时钟系统为教育公平筑起“精准防线”
2025年#高考 将在近日拉开帷幕,#AI 监考一度冲上热搜。当AI深度融入高考,#时间同步 不再是辅助功能,而是决定AI监考系统成败的“生命线”。 AI亮相2025高考,40种异常行为0.5秒精准识别 2025年高考即将拉开帷幕,江西、…...
)
Android第十三次面试总结(四大 组件基础)
Activity生命周期和四大启动模式详解 一、Activity 生命周期 Activity 的生命周期由一系列回调方法组成,用于管理其创建、可见性、焦点和销毁过程。以下是核心方法及其调用时机: onCreate() 调用时机:Activity 首次创建时调用。…...
VM虚拟机网络配置(ubuntu24桥接模式):配置静态IP
编辑-虚拟网络编辑器-更改设置 选择桥接模式,然后找到相应的网卡(可以查看自己本机的网络连接) windows连接的网络点击查看属性 编辑虚拟机设置更改网络配置,选择刚才配置的桥接模式 静态ip设置: 我用的ubuntu24桌…...

招商蛇口 | 执笔CID,启幕低密生活新境
作为中国城市生长的力量,招商蛇口以“美好生活承载者”为使命,深耕全球111座城市,以央企担当匠造时代理想人居。从深圳湾的开拓基因到西安高新CID的战略落子,招商蛇口始终与城市发展同频共振,以建筑诠释对土地与生活的…...
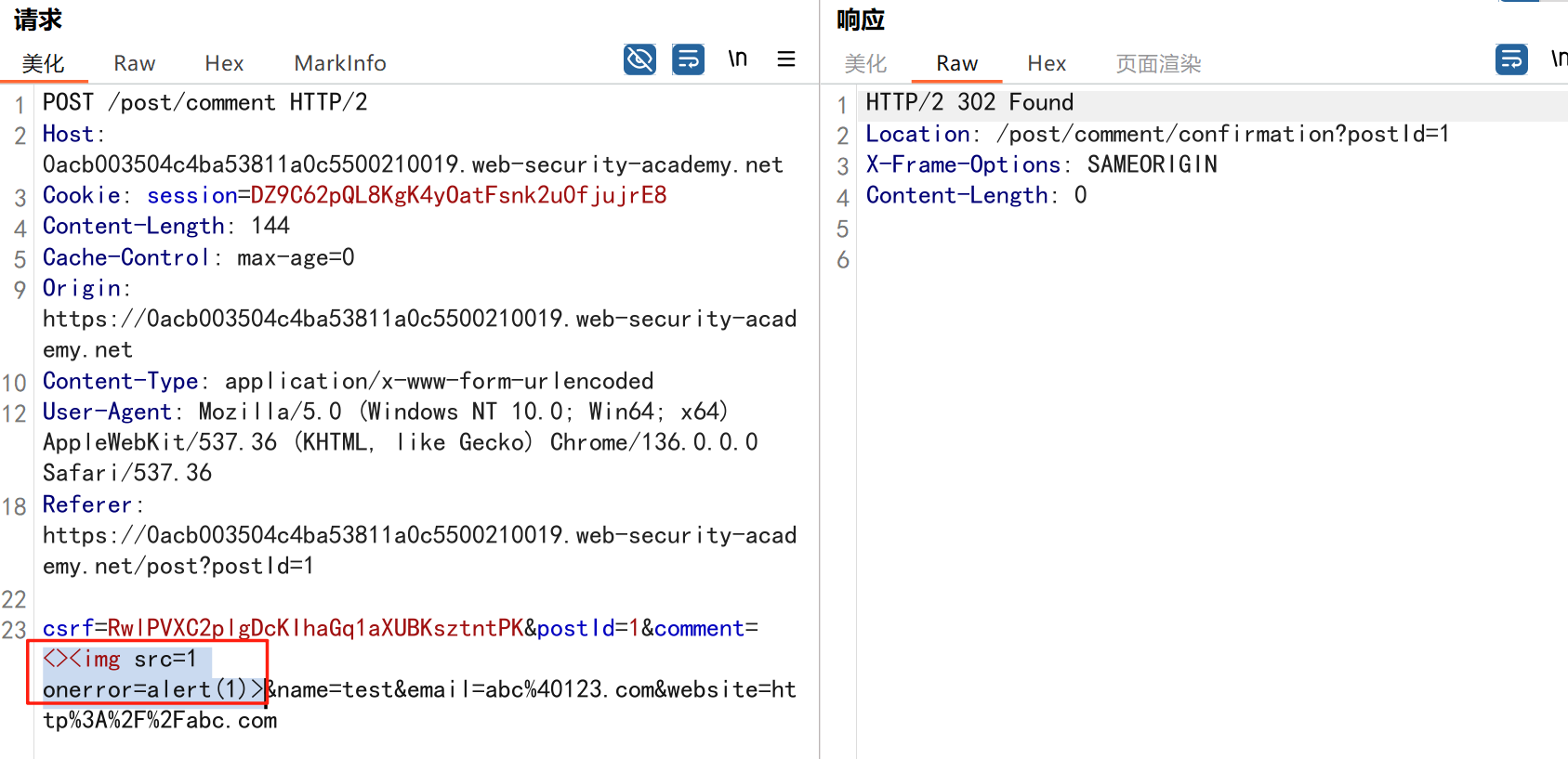
渗透实战PortSwigger靶场:lab13存储型DOM XSS详解
进来是需要留言的,先用做简单的 html 标签测试 发现面的</h1>不见了 数据包中找到了一个loadCommentsWithVulnerableEscapeHtml.js 他是把用户输入的<>进行 html 编码,输入的<>当成字符串处理回显到页面中,看来只是把用户输…...
