AK F.*ing leetcode 流浪计划之半平面求交
欢迎关注更多精彩
关注我,学习常用算法与数据结构,一题多解,降维打击。
本期话题:半平面求交
背景知识
学习资料
视频讲解
https://www.bilibili.com/video/BV1jL411C7Ct/?spm_id_from=333.1007.top_right_bar_window_history.content.click&vd_source=fb27f95f25902a2cc94d4d8e49f5f777
文本资料
https://oi-wiki.org//geometry/half-plane/
基本问题转化
在很多题目中,给定的线段是没有方向的。此时,我们需要先把所有的线段都转化成点加向量的方式。使得向量的左边为有效区域。这样就可以使用模板求解了。
要注意的问题
- 主要的问题是浮点型的判断大小问题。在排序和判断点与线的关系时都用到浮点型判断。有些题型会卡精度,能用整数判断尽量不要使用浮点判断。
- atan2计算比较耗时,可以事先保存。
代码模板
求多边形的核
题目链接:https://vjudge.net/problem/UVA-1571
多边形的核就是取核区域内任意一点,站在该可以观察到多边形内任意一点。
利用半平面求交可以得到多边形的核
#include<stdio.h>
#include<cmath>
#include <algorithm>
#include <vector>
#include <list>
#include <cstring>
#include <set>using namespace std;
const double EPS = 1e-14;const int N = 2e6 + 10;int cmp(double d) {if (abs(d) < EPS)return 0;if (d > 0)return 1;return -1;
}class Point {
public:double x, y;int id;Point() {}Point(double a, double b) :x(a), y(b) {}Point(const Point& p) :x(p.x), y(p.y), id(p.id) {}void in() {scanf("%lf %lf", &x, &y);}void out() {printf("%.16f %.16f\n", x, y);}double dis() {return sqrt(x * x + y * y);}double dis2() {return x * x + y * y;}Point operator -() const {return Point(-x, -y);}Point operator -(const Point& p) const {return Point(x - p.x, y - p.y);}Point operator +(const Point& p) const {return Point(x + p.x, y + p.y);}Point operator *(double d)const {return Point(x * d, y * d);}Point operator /(double d)const {return Point(x / d, y / d);}void operator -=(Point& p) {x -= p.x;y -= p.y;}void operator +=(Point& p) {x += p.x;y += p.y;}void operator *=(double d) {x *= d;y *= d;}void operator /=(double d) {this ->operator*= (1 / d);}bool operator<(const Point& a) const {return x < a.x || (abs(x - a.x) < EPS && y < a.y);}bool operator==(const Point& a) const {return abs(x - a.x) < EPS && abs(y - a.y) < EPS;}
};// 向量操作double cross(const Point& a, const Point& b) {return a.x * b.y - a.y * b.x;
}double dot(const Point& a, const Point& b) {return a.x * b.x + a.y * b.y;
}class Line {
public:Point front, tail;double ang;int u, v;Line() {}Line(const Point& a, const Point& b) :front(a), tail(b) {ang = atan2(front.y - tail.y, front.x - tail.x);}
};int cmp(const Line& a, const Line& b) {//if (a.u == b.u && a.v == b.v)return 0;return cmp(a.ang - b.ang);}// 点在直线哪一边>0 左边,<0边
double SideJudge(const Line& a, const Point& b) {//return cmp(cross(a.front - a.tail, b - a.tail));return cross(a.front - a.tail, b - a.tail);
}int LineSort(const Line& a, const Line& b) {int c = cmp(a, b);if (c)return c < 0;return cross(b.front - b.tail, a.front - b.tail) > 0;
}/*
点p 到 p+r 表示线段1
点q 到 q+s 表示线段2
线段1 上1点用 p' = p+t*r (0<=t<=1)
线段2 上1点用 q' = q+u*s (0<=u<=1)
让两式相等求交点 p+t*r = q+u*s
两边都叉乘s
(p+t*r)Xs = (q+u*s)Xs
pXs + t*rXs = qXs
t = (q-p)Xs/(rXs)
同理,
u = (p-q)Xr/(sXr) -> u = (q-p)Xr/(rXs)以下分4种情况:
1. 共线,sXr==0 && (q-p)Xr==0, 计算 (q-p)在r上的投影在r长度上的占比t0,
计算(q+s-p)在r上的投影在r长度上的占比t1,查看[t0, t1]是否与范围[0,1]有交集。
如果t0>t1, 则比较[t1, t0]是否与范围[0,1]有交集。
t0 = (q-p)*r/(r*r)
t1 = (q+s-p)*r/(r*r) = t0 + s · r / (r · r)
2. 平行sXr==0 && (q-p)Xr!=0
3. 0<=u<=1 && 0<=t<=1 有交点
4. 其他u, t不在0到范围内,没有交点。
*/
pair<double, double> intersection(const Point& q, const Point& s, const Point& p, const Point& r, bool &oneline) {// 计算 (q-p)Xrauto qpr = cross(q - p, r);auto qps = cross(q - p, s);auto rXs = cross(r, s);if (cmp(rXs) == 0) {oneline = true;return { -1, -1 }; // 平行或共线}// 求解t, u// t = (q-p)Xs/(rXs)auto t = qps / rXs;// u = (q-p)Xr/(rXs)auto u = qpr / rXs;return { u, t };
}Point LineCross(const Line& a, const Line& b, bool &f) {Point dira = a.front - a.tail;Point dirb = b.front - b.tail;bool oneline=false;auto p = intersection(a.tail, dira, b.tail, dirb, oneline);if (oneline)f = false;return a.tail + dira * p.first;
}class HalfPlane {
public:vector<Line> lines;vector<int> q;vector<Point> t;int len;HalfPlane() {lines.resize(N);q.resize(N);t.resize(N);}void reset() {len = 0;}void addLine(const Line& a) {lines[len++] = a;}bool run() {sort(lines.begin(), lines.begin() + len, LineSort);int l = -1, r = 0;q[0] = 0;for (int i = 1; i < len; ++i) {if (cmp(lines[i], lines[i - 1]) == 0)continue;while (r - l > 1 && SideJudge(lines[i], t[r]) < 0)r--;while (r - l > 1 && SideJudge(lines[i], t[l + 2]) < 0)l++;q[++r] = i;bool f=true;t[r] = LineCross(lines[q[r]], lines[q[r - 1]], f);}while (r - l > 1 && SideJudge(lines[q[l + 1]], t[r]) < 0)r--;//if (r - l > 1) {// bool f = true;// t[r + 1] = LineCross(lines[q[l + 1]], lines[q[r]], f);// r++;// if (!f)r -= 2;//} 统计交点//l++;//vector<Point> ans(r - l);//for (int i = 0; i < ans.size(); ++i) {// ans[i] = t[i + l + 1];//}return r-l>2;}
};Point oiPs[N * 2];
pair<int, int> ori[N * 2];
HalfPlane hp;int bigDevid(int a, int b) {for (int i = max(abs(a), abs(b)); i >= 1; i--) {if (a % i == 0 && b % i == 0)return i;}return 1;
}void solve() {int n, m = 1;//FILE* fp = fopen("ans.txt", "w");while (scanf("%d", &n) != EOF && n) {int a, b;for (int i = 0; i < n; ++i) {scanf("%d %d", &a, &b);oiPs[i] = Point(a, b);ori[i] = { a,b };}oiPs[n] = oiPs[0];ori[n] = ori[0];hp.reset();for (int i = 0; i < n; ++i) {hp.addLine(Line(oiPs[i+1], oiPs[i]));hp.lines[i].u = ori[i+1].first - ori[i].first;hp.lines[i].v = ori[i+1].second - ori[i].second;int bd = bigDevid(hp.lines[i].u, hp.lines[i].v);hp.lines[i].u /= bd;hp.lines[i].v /= bd;}auto ps = hp.run();if (ps)puts("1");else puts("0");m++;}
}int main() {solve();return 0;}/*
4
0 0
0 1
1 1
1 0
8
0 0
3 0
4 3
2 2
3 4
4 4
4 5
0 5
08
0 0
0 1
1 1
1 2
0 2
0 3
3 3
3 0
*/
练习一
链接:https://www.luogu.com.cn/problem/P4196
求多个凸多边形的交面积。
对每条边进行半平面求交,再利用三角形求多边形面积。
#include<stdio.h>
#include<cmath>
#include <algorithm>
#include <vector>
#include <list>
#include <cstring>
#include <set>using namespace std;
const double EPS = 1e-14;const int N = 2e6 + 10;int cmp(double d) {if (abs(d) < EPS)return 0;if (d > 0)return 1;return -1;
}class Point {
public:double x, y;int id;Point() {}Point(double a, double b) :x(a), y(b) {}Point(const Point& p) :x(p.x), y(p.y), id(p.id) {}void in() {scanf("%lf %lf", &x, &y);}void out() {printf("%.16f %.16f\n", x, y);}double dis() {return sqrt(x * x + y * y);}double dis2() {return x * x + y * y;}Point operator -() const {return Point(-x, -y);}Point operator -(const Point& p) const {return Point(x - p.x, y - p.y);}Point operator +(const Point& p) const {return Point(x + p.x, y + p.y);}Point operator *(double d)const {return Point(x * d, y * d);}Point operator /(double d)const {return Point(x / d, y / d);}void operator -=(Point& p) {x -= p.x;y -= p.y;}void operator +=(Point& p) {x += p.x;y += p.y;}void operator *=(double d) {x *= d;y *= d;}void operator /=(double d) {this ->operator*= (1 / d);}bool operator<(const Point& a) const {return x < a.x || (abs(x - a.x) < EPS && y < a.y);}bool operator==(const Point& a) const {return abs(x - a.x) < EPS && abs(y - a.y) < EPS;}
};// 向量操作double cross(const Point& a, const Point& b) {return a.x * b.y - a.y * b.x;
}double dot(const Point& a, const Point& b) {return a.x * b.x + a.y * b.y;
}class Line {
public:Point front, tail;double ang;int u, v;Line() {}Line(const Point& a, const Point& b) :front(a), tail(b) {ang = atan2(front.y - tail.y, front.x - tail.x);}
};int cmp(const Line& a, const Line& b) {//if (a.u == b.u && a.v == b.v)return 0;return cmp(a.ang - b.ang);}// 点在直线哪一边>0 左边,<0边
double SideJudge(const Line& a, const Point& b) {//return cmp(cross(a.front - a.tail, b - a.tail));return cross(a.front - a.tail, b - a.tail);
}int LineSort(const Line& a, const Line& b) {int c = cmp(a, b);if (c)return c < 0;return cross(b.front - b.tail, a.front - b.tail) > 0;
}/*
点p 到 p+r 表示线段1
点q 到 q+s 表示线段2
线段1 上1点用 p' = p+t*r (0<=t<=1)
线段2 上1点用 q' = q+u*s (0<=u<=1)
让两式相等求交点 p+t*r = q+u*s
两边都叉乘s
(p+t*r)Xs = (q+u*s)Xs
pXs + t*rXs = qXs
t = (q-p)Xs/(rXs)
同理,
u = (p-q)Xr/(sXr) -> u = (q-p)Xr/(rXs)以下分4种情况:
1. 共线,sXr==0 && (q-p)Xr==0, 计算 (q-p)在r上的投影在r长度上的占比t0,
计算(q+s-p)在r上的投影在r长度上的占比t1,查看[t0, t1]是否与范围[0,1]有交集。
如果t0>t1, 则比较[t1, t0]是否与范围[0,1]有交集。
t0 = (q-p)*r/(r*r)
t1 = (q+s-p)*r/(r*r) = t0 + s · r / (r · r)
2. 平行sXr==0 && (q-p)Xr!=0
3. 0<=u<=1 && 0<=t<=1 有交点
4. 其他u, t不在0到范围内,没有交点。
*/
pair<double, double> intersection(const Point& q, const Point& s, const Point& p, const Point& r, bool &oneline) {// 计算 (q-p)Xrauto qpr = cross(q - p, r);auto qps = cross(q - p, s);auto rXs = cross(r, s);if (cmp(rXs) == 0) {oneline = true;return { -1, -1 }; // 平行或共线}// 求解t, u// t = (q-p)Xs/(rXs)auto t = qps / rXs;// u = (q-p)Xr/(rXs)auto u = qpr / rXs;return { u, t };
}Point LineCross(const Line& a, const Line& b, bool &f) {Point dira = a.front - a.tail;Point dirb = b.front - b.tail;bool oneline=false;auto p = intersection(a.tail, dira, b.tail, dirb, oneline);if (oneline)f = false;return a.tail + dira * p.first;
}class HalfPlane {
public:vector<Line> lines;void addLine(const Line& a) {lines.push_back(a);}vector<Point> run() {sort(lines.begin(), lines.end(), LineSort);vector<int> q(lines.size() + 10);vector<Point> t(lines.size() + 10);int l = -1, r = 0;q[0] = 0;for (int i = 1; i < lines.size(); ++i) {if (cmp(lines[i], lines[i - 1]) == 0)continue;while (r - l > 1 && SideJudge(lines[i], t[r]) < 0)r--;while (r - l > 1 && SideJudge(lines[i], t[l + 2]) < 0)l++;q[++r] = i;bool f = true;t[r] = LineCross(lines[q[r]], lines[q[r - 1]], f);}while (r - l > 1 && SideJudge(lines[q[l + 1]], t[r]) < 0)r--;if (r - l > 1) {bool f = true;t[r + 1] = LineCross(lines[q[l + 1]], lines[q[r]], f);r++;}// 统计交点l++;vector<Point> ans(r - l);for (int i = 0; i < ans.size(); ++i) {ans[i] = t[i + l + 1];}return ans;}
};Point oiPs[N];void solve() {int n, m;scanf("%d", &n);HalfPlane hp;int a, b;while (n--) {scanf("%d", &m);for (int i = 0; i < m; ++i) {scanf("%d%d", &a, &b);oiPs[i].x = a;oiPs[i].y = b;}oiPs[m] = oiPs[0];for (int i = 0; i < m; ++i) {hp.addLine(Line(oiPs[i + 1], oiPs[i]));}}auto keyPoints = hp.run();double ans = 0;for (int i = 2; i < keyPoints.size(); ++i) {ans += cross(keyPoints[i - 1] - keyPoints[0], keyPoints[i] - keyPoints[0]);}printf("%.3f\n", ans / 2);
}int main() {solve();return 0;}/*
3
3
-1 2
-2 1
-1 13
1 1
2 1
1 23
1 1
3 0
2 2*/
本人码农,希望通过自己的分享,让大家更容易学懂计算机知识。创作不易,帮忙点击公众号的链接。

相关文章:

AK F.*ing leetcode 流浪计划之半平面求交
欢迎关注更多精彩 关注我,学习常用算法与数据结构,一题多解,降维打击。 本期话题:半平面求交 背景知识 学习资料 视频讲解 https://www.bilibili.com/video/BV1jL411C7Ct/?spm_id_from333.1007.top_right_bar_window_history…...

docker搭建zokeeper集群、kafka集群
三台机器,ip分别为ip1,ip2,ip3 一、安装docker集群 1、三台机器分别拉取镜像 docker pull wurstmeister/zookeeper 2、三台机器分别运行容器 (1)第一台 docker run -d --restartalways --log-driver json-file --log-opt max-size100m --lo…...
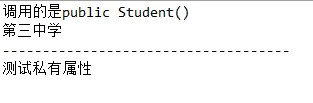
【java学习—十四】反射机制调用指定方法、指定属性(5)
文章目录 1. 调用指定方法2. 调用指定属性 1. 调用指定方法 通过反射,调用类中的方法,通过 Method 类完成。步骤: ①通过 Class 类的 getMethod(String name,Class...parameterTypes) 方法取得一个 Method 对象,并设置此…...

PC端微信@所有人逻辑漏洞
(一)过程 这个漏洞是PC端微信,可以越权让非管理员艾特所有人,具体步骤如下 第一步:找一个自己的群(要有艾特所有人的权限)“123”是我随便输入的内容,可以更改,然后按c…...
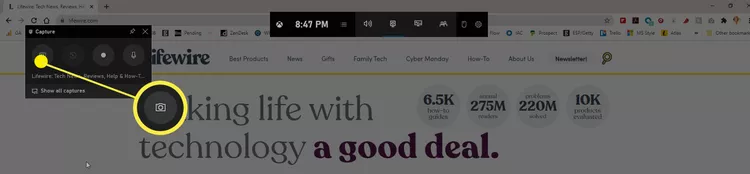
如何在Windows 10中进行屏幕截图
本文介绍如何在Windows 10中捕获屏幕截图,包括使用键盘组合、使用Snipping Tool、Snipp&Sketch Tool或Windows游戏栏。 使用打印屏幕在Windows 10中捕获屏幕截图 在Windows 10中捕获屏幕截图的最简单方法是按下键盘上的PrtScWindows键盘组合。你将看到屏幕短暂…...

【nlp】2.4 GRU模型
GRU模型 1 GRU介绍2 GRU的内部结构图2.1 GRU结构分析2.2 Bi-GRU介绍2.3 使用Pytorch构建GRU模型2.4 GRU优缺点3 RNN及其变体1 GRU介绍 GRU(Gated Recurrent Unit)也称门控循环单元结构, 它也是传统RNN的变体, 同LSTM一样能够有效捕捉长序列之间的语义关联, 缓解梯度消失或爆…...

国科云:浅谈DNS缓存投毒常见类型和防御策略
为了提升解析效率减轻各级服务器的解析压力,DNS系统中引入了缓存机制,但这同样也带来了较大的安全隐患,为攻击者利用DNS缓存进行投毒攻击创造了条件,对DNS系统的安全造成了巨大破坏。本文国科云将分析缓存投毒的两种主要类型&…...
之tcpdump)
Linux命令(120)之tcpdump
linux命令之tcpdump 1.tcpdump介绍 linux命令tcpdump是用来将网络中传送的数据包完全截获下来以进行相关分析,常用的分析工具是wireshark 2.tcpdump用法 tcpdump [参数] tcpdump参数 参数说明-i指定端口-n指定协议-t在输出的每一行不打印时间戳-s抓取数据包时&a…...

2311rust对接C
原文 为了与其他语言通信,Rust提供了(FFI)外部函数接口.FFI是Rust和C间的函数调用,与C函数调用有相同性能的零成本抽象. FFI绑定还可利用(如所有权和借用)语言功能来提供强制指针和其他资源协议的安全接口. Rust与C对话 从Rust调用C代码的简单示例开始.如下为C代码: int do…...
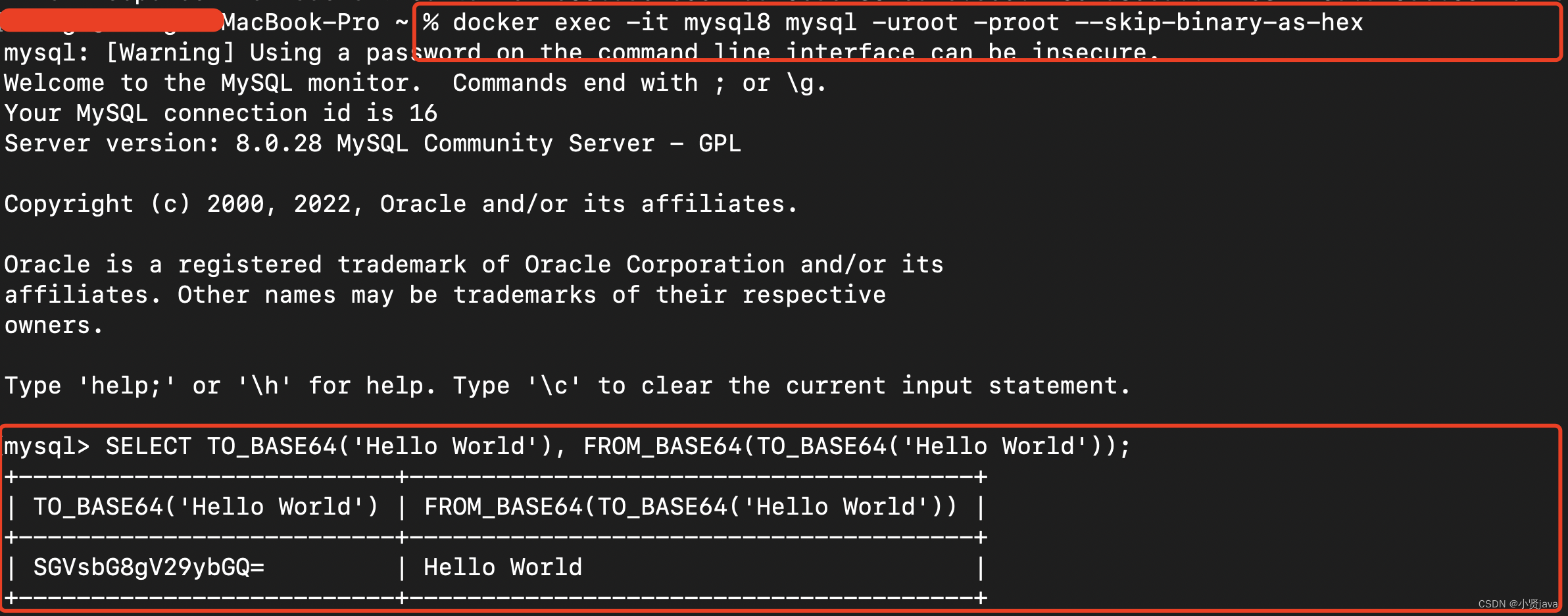
MYSQL字符串函数详解和实战(字符串函数大全,内含示例)
MySQL提供了许多字符串函数,用于处理和操作字符串数据。以下是一些常用的MYSQL字符串函数。 建议收藏以备后续用到查阅参考。 目录 一、CONCAT 拼接字符串 二、CONCAT_WS 拼接字符串 三、SUBSTR 取子字符串 四、SUBSTRING 取子字符串 五、SUBSTRING_INDEX 取子…...

从C语言到C++_40(多线程相关)C++线程接口+线程安全问题加锁(shared_ptr+STL+单例)
目录 1. C多线程 1.1 thread库 1.2 mutex库 1.3 RAII锁 1.4 atomicCAS 1.5 condition_variable 1.6 分别打印奇数和偶数 2. shared_ptr线程安全 2.1 库里面的shared_ptr使用 2.2 shared_ptr加锁代码 3. 单例模式线程安全 3.1 懒汉模式线程安全问题 3.2 懒汉模式最…...
)
Angular 指令介绍及使用(三)
Angular 指令概述 在 Angular 中,指令是一种机制,用于扩展和修改组件的行为和外观。指令可以由开发者自定义,也可以是 Angular 框架自带的一些内置指令。通过使用指令,我们可以在 HTML 模板中通过属性或元素名来操作组件。 Angu…...
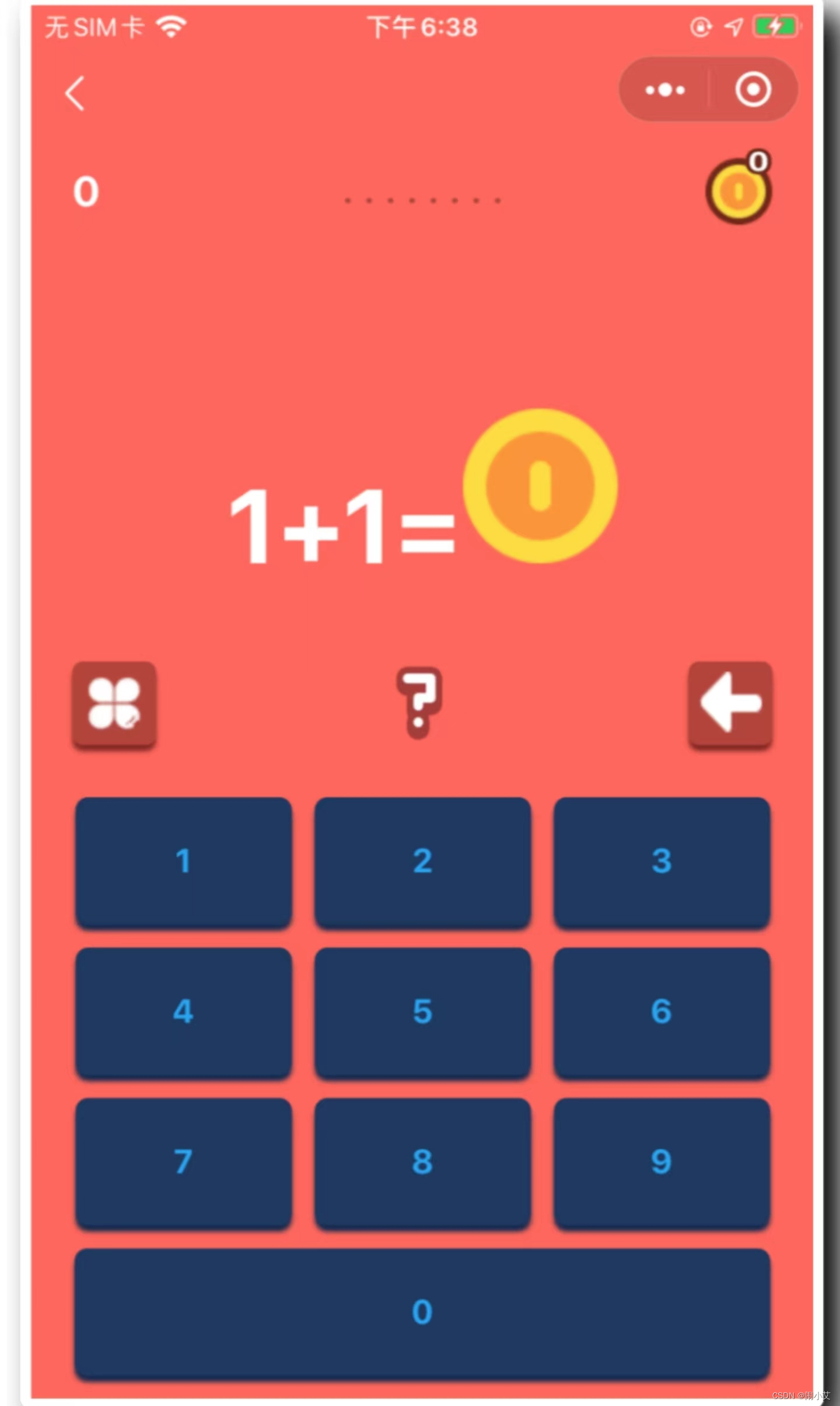
小学生加减乘除闯关运算练习流量主微信小程序开发
小学生加减乘除闯关运算练习流量主微信小程序开发 经过本次更新,我们增加了新的功能和特性,以提升用户体验和运算练习的趣味性: 能量石与激励视频:用户可以通过观看激励视频来获取能量石,这些能量石可以用于解锁收费…...

普通测径仪升级的智能测径仪 增添11大实用功能!
普通测径仪能对各种钢材进行非接触式的外径及椭圆度在线检测,测量数据准确且无损,可测、监测、超差提示、系统分析等。在此基础上,为测径仪进行了进一步升级制成智能测径仪,为其增添更多智能化模块,让其使用更加方便。…...

vue做的一个一点就转的转盘(音乐磁盘),点击停止时会在几秒内缓慢停止,再次点击按钮可以再次旋转,
先看效果: 代码:主要部分我会红线画出来 css:部分: 源码: vue部分: <template><div class"song-lyric"><div><div class"type"><div class"right">&l…...
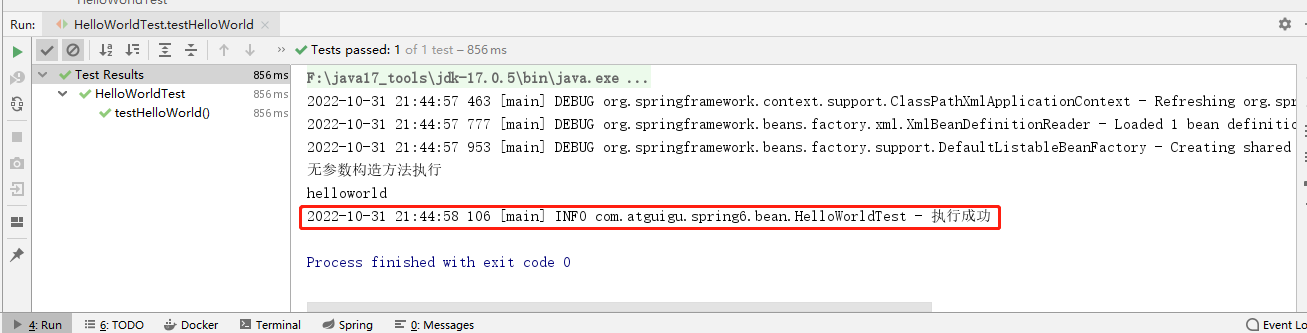
Spring6(一):入门案例
文章目录 1. 概述1.1 Spring简介1.2 Spring 的狭义和广义1.3 Spring Framework特点1.4 Spring模块组成 2 入门2.1 构建模块2.2 程序开发2.2.1 引入依赖2.2.2 创建java类2.2.3 创建配置文件2.2.4 创建测试类测试 2.3 程序分析2.4 启用Log4j2日志框架2.4.1 引入Log4j2依赖2.4.2 加…...

Linux中报错no space device解决思路
1,df -h :查看所有文件下的磁盘使用情况。注意,查询的最后一栏属性就是分区所在的目录路径 2,进到具体的文件下,接着命令:du -sh * | grep G 搜索G以上的文本。 没搜到内容的话,使用命令du -sh…...

vue3使用element-plus
安装 # NPM $ npm install element-plus --save# Yarn $ yarn add element-plus# pnpm $ pnpm install element-plus 全局引入 main.js // main.ts import { createApp } from vue import ElementPlus from element-plus//引入ElementPlus所有组件 import element-plus/dis…...

高质量实时渲染笔记
文章目录 Real-time shadows1 自遮挡问题2 解决阴影detach问题?3 Aliasing4 近似积分5 percentage closer soft shadows(PCSS)percenta closer filtering(PCF)PCSS的思想 6 Variance Soft Shadow Mapping (VSSM)步骤Moment Shadow Mapping 7 Distance field shadow …...
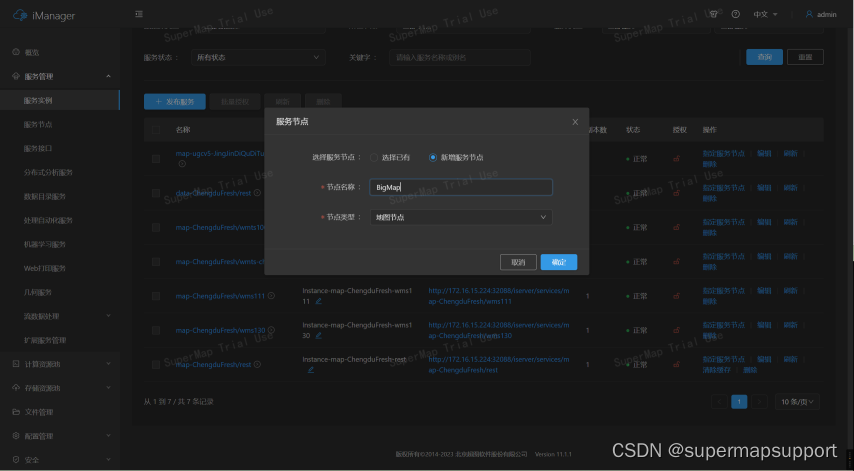
云原生下GIS服务规划与设计
作者:lisong 目录 背景云原生环境下GIS服务的相关概念GIS服务在云原生环境下的规划调度策略GIS服务在云原生环境下的调度手段GIS服务在云原生环境下的服务规划调度实践 背景 作为云原生GIS系统管理人员,在面对新建的云GIS系统时,通常需要应对…...
)
React Native 开发环境搭建(全平台详解)
React Native 开发环境搭建(全平台详解) 在开始使用 React Native 开发移动应用之前,正确设置开发环境是至关重要的一步。本文将为你提供一份全面的指南,涵盖 macOS 和 Windows 平台的配置步骤,如何在 Android 和 iOS…...

java 实现excel文件转pdf | 无水印 | 无限制
文章目录 目录 文章目录 前言 1.项目远程仓库配置 2.pom文件引入相关依赖 3.代码破解 二、Excel转PDF 1.代码实现 2.Aspose.License.xml 授权文件 总结 前言 java处理excel转pdf一直没找到什么好用的免费jar包工具,自己手写的难度,恐怕高级程序员花费一年的事件,也…...
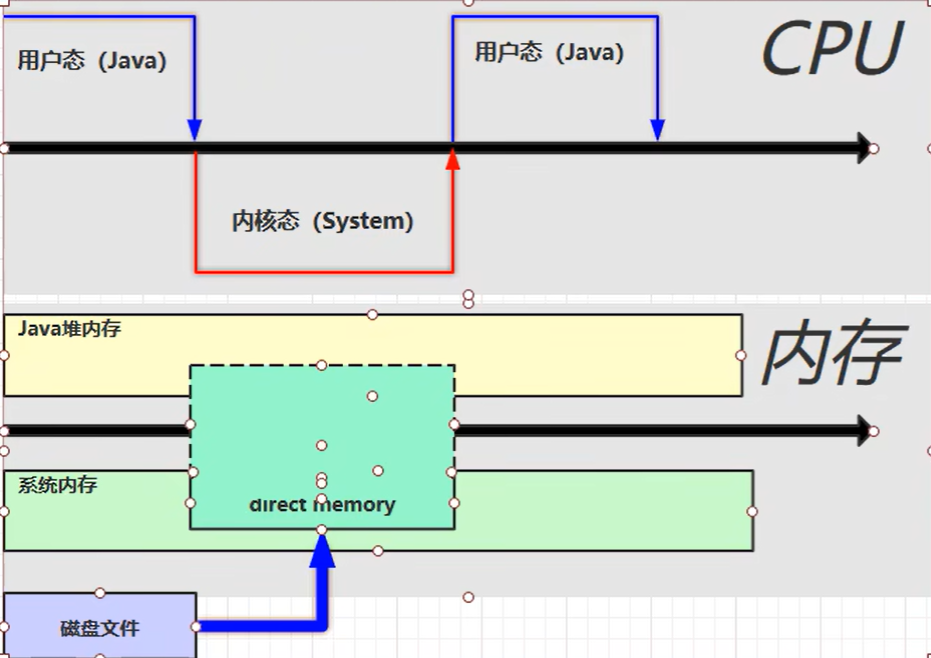
【JVM】- 内存结构
引言 JVM:Java Virtual Machine 定义:Java虚拟机,Java二进制字节码的运行环境好处: 一次编写,到处运行自动内存管理,垃圾回收的功能数组下标越界检查(会抛异常,不会覆盖到其他代码…...

服务器硬防的应用场景都有哪些?
服务器硬防是指一种通过硬件设备层面的安全措施来防御服务器系统受到网络攻击的方式,避免服务器受到各种恶意攻击和网络威胁,那么,服务器硬防通常都会应用在哪些场景当中呢? 硬防服务器中一般会配备入侵检测系统和预防系统&#x…...

C# 类和继承(抽象类)
抽象类 抽象类是指设计为被继承的类。抽象类只能被用作其他类的基类。 不能创建抽象类的实例。抽象类使用abstract修饰符声明。 抽象类可以包含抽象成员或普通的非抽象成员。抽象类的成员可以是抽象成员和普通带 实现的成员的任意组合。抽象类自己可以派生自另一个抽象类。例…...

uniapp微信小程序视频实时流+pc端预览方案
方案类型技术实现是否免费优点缺点适用场景延迟范围开发复杂度WebSocket图片帧定时拍照Base64传输✅ 完全免费无需服务器 纯前端实现高延迟高流量 帧率极低个人demo测试 超低频监控500ms-2s⭐⭐RTMP推流TRTC/即构SDK推流❌ 付费方案 (部分有免费额度&#x…...
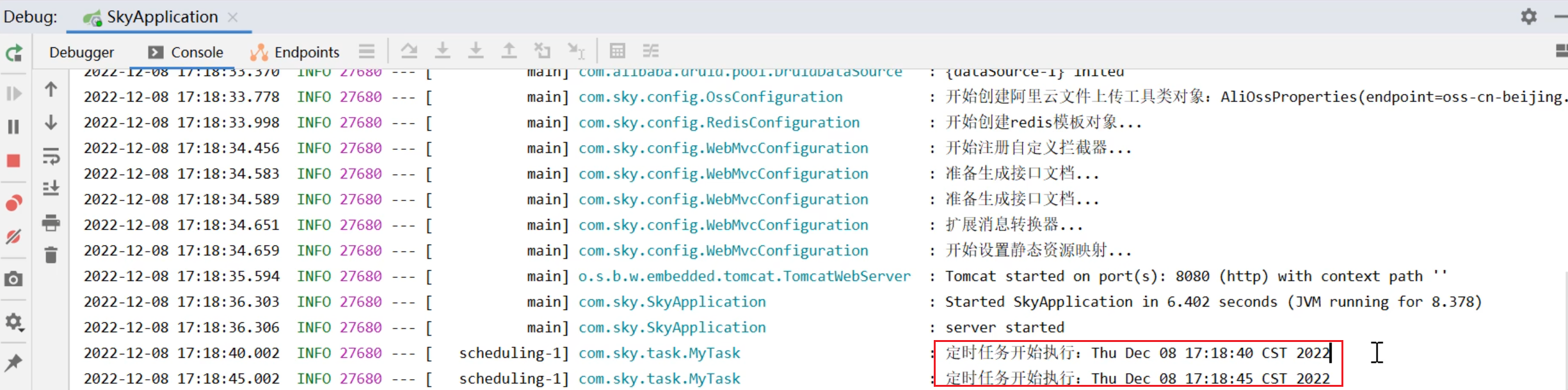
SpringTask-03.入门案例
一.入门案例 启动类: package com.sky;import lombok.extern.slf4j.Slf4j; import org.springframework.boot.SpringApplication; import org.springframework.boot.autoconfigure.SpringBootApplication; import org.springframework.cache.annotation.EnableCach…...
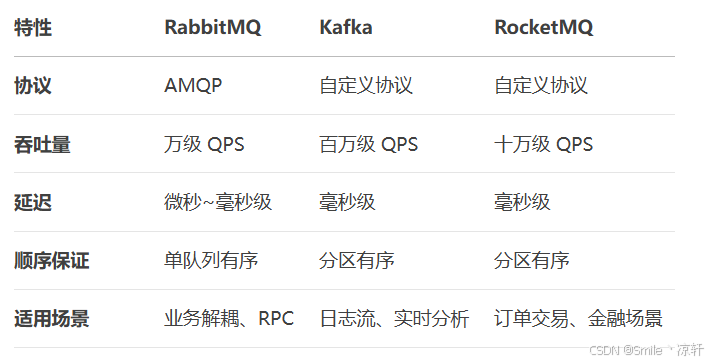
技术栈RabbitMq的介绍和使用
目录 1. 什么是消息队列?2. 消息队列的优点3. RabbitMQ 消息队列概述4. RabbitMQ 安装5. Exchange 四种类型5.1 direct 精准匹配5.2 fanout 广播5.3 topic 正则匹配 6. RabbitMQ 队列模式6.1 简单队列模式6.2 工作队列模式6.3 发布/订阅模式6.4 路由模式6.5 主题模式…...

站群服务器的应用场景都有哪些?
站群服务器主要是为了多个网站的托管和管理所设计的,可以通过集中管理和高效资源的分配,来支持多个独立的网站同时运行,让每一个网站都可以分配到独立的IP地址,避免出现IP关联的风险,用户还可以通过控制面板进行管理功…...

三分算法与DeepSeek辅助证明是单峰函数
前置 单峰函数有唯一的最大值,最大值左侧的数值严格单调递增,最大值右侧的数值严格单调递减。 单谷函数有唯一的最小值,最小值左侧的数值严格单调递减,最小值右侧的数值严格单调递增。 三分的本质 三分和二分一样都是通过不断缩…...
