【傅里叶级数与傅里叶变换】数学推导——1、基础知识点回顾及[Part1:三角函数的正交性]介绍
文章内容来自DR_CAN关于傅里叶变换的视频,本篇文章提供了一些基础知识点,比如三角函数常用的导数、三角函数换算公式等。
文章全部链接:
基础知识点
Part1:三角函数系的正交性
Part2:T=2π的周期函数的傅里叶级数展开
Part3:周期为T=2L的函数展开
Part4:傅里叶级数的复数形式
Part5:从傅里叶级数推导傅里叶变换
总结
文章目录
- 基础知识点
- Part1:三角函数系的正交性
基础知识点
设 α \alpha α为任意角
f ( 2 k π + α ) = f α ( k ∈ Z ) ,其中 f 为 s i n 或 c o s 或 t a n 或 c o t s i n ( π + α ) = − s i n α c o s ( π + α ) = − c o s α t a n ( π + α ) = t a n α c o t ( π + α ) = c o t α s i n ( − α ) = − s i n α c o s ( − α ) = c o s α t a n ( π + α ) = − t a n α c o t ( π + α ) = − c o t α s i n ( π − α ) = s i n α c o s ( π − α ) = − c o s α t a n ( π − α ) = − t a n α c o t ( π − α ) = − c o t α s i n ( 2 π − α ) = − s i n α c o s ( 2 π − α ) = c o s α t a n ( 2 π − α ) = − t a n α c o t ( 2 π − α ) = − c o t α \begin{align} & f(2k \pi + \alpha) = f \alpha (k \in Z) ,其中f为 sin或cos或tan或cot \\ \\ & sin(\pi + \alpha) = -sin \alpha \\ & cos(\pi + \alpha) = -cos \alpha \\ & tan(\pi + \alpha) = tan \alpha \\ & cot(\pi + \alpha) = cot \alpha \\ \\ & sin(- \alpha) = - sin \alpha \\ & cos(- \alpha) = cos \alpha \\ & tan(\pi + \alpha) = -tan \alpha \\ & cot(\pi + \alpha) = -cot \alpha \\ \\ & sin(\pi - \alpha) = sin \alpha \\ & cos(\pi - \alpha) = - cos \alpha \\ & tan(\pi - \alpha) = - tan \alpha \\ & cot(\pi - \alpha) = -cot \alpha \\ \\ & sin(2 \pi - \alpha) = -sin \alpha \\ & cos(2 \pi - \alpha) = cos \alpha \\ & tan(2 \pi - \alpha) = -tan \alpha \\ & cot(2 \pi - \alpha) = -cot \alpha & \end{align} f(2kπ+α)=fα(k∈Z),其中f为sin或cos或tan或cotsin(π+α)=−sinαcos(π+α)=−cosαtan(π+α)=tanαcot(π+α)=cotαsin(−α)=−sinαcos(−α)=cosαtan(π+α)=−tanαcot(π+α)=−cotαsin(π−α)=sinαcos(π−α)=−cosαtan(π−α)=−tanαcot(π−α)=−cotαsin(2π−α)=−sinαcos(2π−α)=cosαtan(2π−α)=−tanαcot(2π−α)=−cotα
积化和差公式:
sin α cos β = sin ( α + β ) + sin ( α − β ) 2 cos α sin β = sin ( α + β ) − sin ( α − β ) 2 cos α cos β = cos ( α + β ) + cos ( α − β ) 2 sin α sin β = − cos ( α + β ) − cos ( α − β ) 2 \begin{align} & \sin \alpha \cos \beta ={\sin(\alpha +\beta )+\sin(\alpha -\beta ) \over 2} \\ & \cos \alpha \sin \beta ={\sin(\alpha +\beta )-\sin(\alpha -\beta ) \over 2} \\ & \cos \alpha \cos \beta ={\cos(\alpha +\beta )+\cos(\alpha -\beta ) \over 2}\\ & \sin \alpha \sin \beta =-{\cos(\alpha +\beta )-\cos(\alpha -\beta ) \over 2} & \end{align} sinαcosβ=2sin(α+β)+sin(α−β)cosαsinβ=2sin(α+β)−sin(α−β)cosαcosβ=2cos(α+β)+cos(α−β)sinαsinβ=−2cos(α+β)−cos(α−β)
和差化积公式:
s i n α + s i n β = 2 s i n α + β 2 c o s α − β 2 s i n α − s i n β = 2 c o s α + β 2 s i n α − β 2 c o s α + c o s β = 2 c o s α + β 2 c o s α − β 2 c o s α − c o s β = − 2 s i n α + β 2 s i n α − β 2 \begin{align} & sin \alpha + sin \beta = 2sin\frac {\alpha + \beta} {2} cos \frac {\alpha - \beta} {2} \\ & sin \alpha - sin \beta = 2cos\frac {\alpha + \beta} {2} sin \frac {\alpha - \beta} {2} \\ & cos \alpha + cos \beta = 2 cos\frac {\alpha + \beta} {2} cos \frac {\alpha - \beta} {2} \\ & cos \alpha - cos \beta = - 2 sin\frac {\alpha + \beta} {2} sin \frac {\alpha - \beta} {2} & \end{align} sinα+sinβ=2sin2α+βcos2α−βsinα−sinβ=2cos2α+βsin2α−βcosα+cosβ=2cos2α+βcos2α−βcosα−cosβ=−2sin2α+βsin2α−β
倍角公式:
s i n 2 α = s i n α c o s α + s i n α c o s α = 2 s i n α c o s α c o s 2 α = c o s 2 α − s i n 2 α = 2 c o s 2 α − 1 = 1 − 2 s i n 2 α c o s 2 α = 1 + c o s 2 α 2 s i n 2 α = 1 − c o s 2 α 2 t a n 2 α = 2 t a n α 1 − t a n 2 α \begin{align} & sin 2 \alpha = sin \alpha cos \alpha + sin \alpha cos \alpha = 2 sin \alpha cos \alpha \\ & cos 2 \alpha = cos^2 \alpha - sin ^2 \alpha =2 cos ^ 2 \alpha -1 = 1- 2 sin^2 \alpha \\ & cos ^ 2 \alpha = \frac{1 + cos 2 \alpha}{2} \\ & sin^ 2 \alpha = \frac{1 - cos 2 \alpha}{2} \\ & tan 2 \alpha = \frac {2 tan \alpha} {1 - tan ^2 \alpha} & \end{align} sin2α=sinαcosα+sinαcosα=2sinαcosαcos2α=cos2α−sin2α=2cos2α−1=1−2sin2αcos2α=21+cos2αsin2α=21−cos2αtan2α=1−tan2α2tanα
三角函数导数:
正弦函数: ( s i n x ) ′ = c o s x 余弦函数: ( c o s x ) ′ = − s i n x 正切函数: ( t a n x ) ′ = s e c 2 x 余切函数: ( c o t x ) ′ = − c s c 2 x \begin{align} & 正弦函数: (sin x)' = cosx \\ & 余弦函数: (cos x)' = -sinx \\ & 正切函数: (tan x )' = sec^2 x \\ & 余切函数: (cot x)' = -csc^2x & \end{align} 正弦函数:(sinx)′=cosx余弦函数:(cosx)′=−sinx正切函数:(tanx)′=sec2x余切函数:(cotx)′=−csc2x
积分性质:
性质 1 :如果 c 是常数, ∫ a b c d x = c ( b − a ) ; 性质 2 :如果 c 是常数, ∫ z b c f ( x ) d x = c ∫ a b f ( x ) d x ; 性质 3 : ∫ a b [ f ( x ) ± g ( x ) ] d x = ∫ a b f ( x ) d x ± ∫ z b g ( x ) d x ; \begin{align} & 性质1:如果c是常数,\int_{a}^{b} cdx = c(b-a); \\ & 性质2:如果c是常数,\int_z^b cf(x)dx = c \int_a^b f(x)dx; \\ & 性质3:\int_{a}^{b}[f(x) \pm g(x)] dx = \int_a^b f(x)dx \pm \int_z^b g(x)dx; & \end{align} 性质1:如果c是常数,∫abcdx=c(b−a);性质2:如果c是常数,∫zbcf(x)dx=c∫abf(x)dx;性质3:∫ab[f(x)±g(x)]dx=∫abf(x)dx±∫zbg(x)dx;
Part1:三角函数系的正交性
三角函数系集合: { 0 , 1 , s i n x , c o s x , s i n 2 x , c o s 2 x , . . . , s i n n x , c o s n x , . . . } \{0,1,sin x, cos x, sin 2x, cos 2x, ...,sin nx, cos nx, ...\} {0,1,sinx,cosx,sin2x,cos2x,...,sinnx,cosnx,...},即为 { s i n n x , c o s n x } \{sin nx, cos nx\} {sinnx,cosnx},其中 n = 0 , 1 , 2 , . . . n = 0, 1,2,... n=0,1,2,...。
对于两个向量 a ⃗ \vec{a} a和 b ⃗ \vec {b} b,如果它们的内积等于0,表示这两个向量是垂直的,具有正交性。即 a ⃗ ⋅ b ⃗ = 0 \vec{a} \cdot \vec{b} = 0 a⋅b=0,如下示意图, a ⃗ ⋅ b ⃗ = 2 ∗ − 1 + 1 ∗ 2 = 0 \vec{a} \cdot \vec{b} = 2*-1 + 1*2 = 0 a⋅b=2∗−1+1∗2=0。
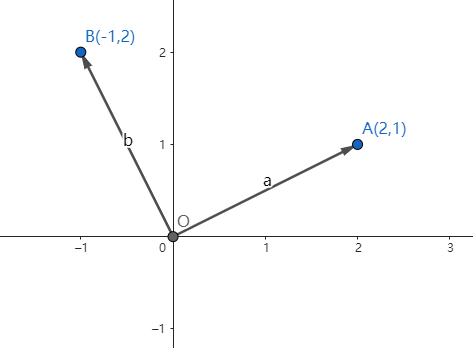
若向量 a ⃗ \vec{a} a和向量 b ⃗ \vec{b} b中包含三个元素,分别是 ( a 1 , a 2 , a 3 ) (a_1, a_2, a_3) (a1,a2,a3)和 ( b 1 , b 2 , b 3 ) (b_1, b_2, b_3) (b1,b2,b3),如果 a ⃗ ⋅ b ⃗ = a 1 b 1 + a 2 b 2 + a 3 b 3 = 0 \vec{a} \cdot \vec{b} = a_1 b_1 + a_2 b_2 + a_3 b_3 = 0 a⋅b=a1b1+a2b2+a3b3=0,那么 a ⃗ \vec{a} a与 b ⃗ \vec {b} b正交。
再推广到 a ⃗ \vec a a和 b ⃗ \vec b b中有 n n n个元素,分别为 { a 1 , a 2 , a 3 , . . . , a n } \{ a_1, a_2, a_3, ..., a_n \} {a1,a2,a3,...,an}和 { b 1 , b 2 , b 3 , . . . , b n } \{ b_1, b_2, b_3, ... , b_n \} {b1,b2,b3,...,bn},如果 a ⃗ ⋅ b ⃗ = a 1 b 1 + a 2 b 2 + a 3 b 3 + . . a n b n = ∑ i = 1 n a i b i = 0 \vec a \cdot \vec b = a_1 b_1 + a_2 b_2 + a_3 b_3 + .. a_n b_n = \sum_{i=1} ^ {n} a_i b_i = 0 a⋅b=a1b1+a2b2+a3b3+..anbn=∑i=1naibi=0,那么 a ⃗ \vec a a与 b ⃗ \vec b b正交。
再扩展,如果 a = f ( x ) a = f(x) a=f(x), b = g ( x ) b = g(x) b=g(x),在一个区间内如 [ x 0 , x 1 ] [x_0, x_1] [x0,x1]计算 a ⋅ b a \cdot b a⋅b即 f ( x ) ⋅ g ( x ) f(x) \cdot g(x) f(x)⋅g(x),也就是计算在区间 [ x 0 , x 1 ] [x_0, x_1] [x0,x1]内的面积和,相当于求积分 ∫ x 0 x 1 f ( x ) g ( x ) d x \int_{x_0}^{x_1} f(x) g(x)dx ∫x0x1f(x)g(x)dx。如果 ∫ x 0 x 1 f ( x ) g ( x ) d x = 0 \int_{x_0}^{x_1} f(x) g(x)dx=0 ∫x0x1f(x)g(x)dx=0,就可以说 f ( x ) f(x) f(x)和 g ( x ) g(x) g(x)这两个函数正交。
再说回到三角函数的正交性的定义:从三角函数系集合中任取两项,在 [ − π , π ] [-\pi, \pi] [−π,π]之间,这两项的积分等于0。
即:
∫ − π π s i n ( n x ) c o s ( m x ) d x = 0 ∫ − π π c o s ( n x ) s i n ( m x ) d x = 0 ∫ − π π s i n ( n x ) s i n ( m x ) d x = 0 ∫ − π π c o s ( n x ) c o s ( m x ) d x = 0 \begin{align} & \int_{- \pi}^{\pi} sin (nx) cos (mx) dx = 0 \\ & \int_{- \pi}^{\pi} cos (nx) sin (mx) dx = 0 \\ & \int_{- \pi}^{\pi} sin (nx) sin (mx) dx = 0 \\ & \int_{- \pi}^{\pi} cos (nx) cos (mx) dx = 0 & \end{align} ∫−ππsin(nx)cos(mx)dx=0∫−ππcos(nx)sin(mx)dx=0∫−ππsin(nx)sin(mx)dx=0∫−ππcos(nx)cos(mx)dx=0
接下来证明三角函数的正交性。
当 m ≠ n m \ne n m=n时,由积化和差公式 s i n α c o s β = 1 2 [ s i n ( α + β ) + s i n ( α − β ) ] sin \alpha cos \beta = \frac{1}{2} [sin(\alpha + \beta) + sin(\alpha - \beta)] sinαcosβ=21[sin(α+β)+sin(α−β)]、复合导数求导、三角函数常用导数公式以及积分性质可证:
∫ − π π s i n ( n x ) c o s ( m x ) d x = 1 2 [ ∫ − π π s i n ( n + m ) x d x + ∫ − π π s i n ( n − m ) x d x ] = 1 2 [ ( − 1 n + m c o s ( n + m ) x ∣ − π π ) + ( − 1 n − m c o s ( n − m ) x ∣ − π π ) ] = 1 2 [ ( − 1 n + m ) [ c o s ( n + m ) π − c o s ( − ( n + m ) π ) ] + ( − 1 n − m ) [ c o s ( n − m ) π − c o s ( − ( n − m ) π ) ] ] 有 c o s ( − α ) = c o s α ,很容易得出上式为 0 , 即: ∫ − π π s i n ( n x ) c o s ( m x ) d x = 0 \begin{align} &\int_{- \pi}^{\pi} sin (nx) cos (mx) dx \\ & = \frac {1}{2} \left [ \int_{- \pi}^{\pi} sin(n + m)x dx + \int_{- \pi}^{\pi}sin(n-m)x dx \right] \\ & = \frac{1}{2} \left[ (- \frac{1}{n+m} cos(n+m)x |_{- \pi}^{\pi}) + (- \frac{1}{n-m} cos(n-m)x |_{- \pi}^{\pi}) \right] \\ & = \frac{1}{2} \left [(- \frac{1}{n+m}) \left[ cos(n+m) \pi - cos(-(n+m)\pi) \right] + (- \frac{1}{n-m} ) \left[ cos(n-m) \pi - cos(-(n-m)\pi) \right] \right ] \\ & 有cos (-\alpha) = cos \alpha,很容易得出上式为0 , 即: \int_{- \pi}^{\pi} sin (nx) cos (mx) dx = 0 & \end{align} ∫−ππsin(nx)cos(mx)dx=21[∫−ππsin(n+m)xdx+∫−ππsin(n−m)xdx]=21[(−n+m1cos(n+m)x∣−ππ)+(−n−m1cos(n−m)x∣−ππ)]=21[(−n+m1)[cos(n+m)π−cos(−(n+m)π)]+(−n−m1)[cos(n−m)π−cos(−(n−m)π)]]有cos(−α)=cosα,很容易得出上式为0,即:∫−ππsin(nx)cos(mx)dx=0
当 m ≠ n m \ne n m=n时,由积化和差公式 c o s α s i n β = 1 2 [ s i n ( α + β ) − s i n ( α − β ) ] cos\alpha sin\beta = \frac{1}{2} [sin(\alpha + \beta) - sin(\alpha - \beta)] cosαsinβ=21[sin(α+β)−sin(α−β)]、复合导数求导、三角函数常用导数公式以及积分性质可证:
∫ − π π c o s ( n x ) s i n ( m x ) d x = 1 2 [ ∫ − π π s i n ( n + m ) x d x − ∫ − π π s i n ( n − m ) x d x ] = 1 2 [ ( − 1 n + m ) c o s ( n + m ) x ∣ − π π ) − ( − 1 n − m ) c o s ( n − m ) x ∣ − π π ) ] = 1 2 [ ( − 1 n + m ) [ c o s ( n + m ) π − c o s ( − ( n + m ) π ) ] − ( − 1 n − m ) [ c o s ( n − m ) π − c o s ( − ( n − m ) π ) ] ] 有 c o s ( − α ) = c o s α ,很容易得出上式为 0 , 即: ∫ − π π c o s ( n x ) s i n ( m x ) d x = 0 \begin{align} & \int_{- \pi}^{\pi} cos (nx) sin (mx) dx \\ & =\frac {1}{2} \left [ \int_{- \pi}^{\pi} sin(n + m)x dx - \int_{- \pi}^{\pi}sin(n-m)x dx \right] \\ & = \frac{1}{2} \left[ (- \frac{1}{n+m}) cos(n+m)x |_{- \pi}^{\pi}) - (- \frac{1}{n-m} )cos(n-m)x |_{- \pi}^{\pi}) \right] \\ & = \frac{1}{2} \left [(- \frac{1}{n+m}) \left[ cos(n+m) \pi - cos(-(n+m)\pi) \right] - (- \frac{1}{n-m} ) \left[ cos(n-m) \pi - cos(-(n-m)\pi) \right] \right ] \\ & 有cos (-\alpha) = cos \alpha,很容易得出上式为0 , 即: \int_{- \pi}^{\pi} cos (nx) sin (mx) dx = 0 & \end{align} ∫−ππcos(nx)sin(mx)dx=21[∫−ππsin(n+m)xdx−∫−ππsin(n−m)xdx]=21[(−n+m1)cos(n+m)x∣−ππ)−(−n−m1)cos(n−m)x∣−ππ)]=21[(−n+m1)[cos(n+m)π−cos(−(n+m)π)]−(−n−m1)[cos(n−m)π−cos(−(n−m)π)]]有cos(−α)=cosα,很容易得出上式为0,即:∫−ππcos(nx)sin(mx)dx=0
当 m ≠ n m \ne n m=n时,由积化和差公式 c o s α c o s β = 1 2 [ c o s ( α + β ) + c o s ( α − β ) ] cos\alpha cos\beta = \frac{1}{2} [cos(\alpha + \beta) + cos(\alpha - \beta)] cosαcosβ=21[cos(α+β)+cos(α−β)]、复合导数求导、三角函数常用导数公式以及积分性质可证:
∫ − π π c o s ( n x ) c o s ( m x ) d x = 1 2 [ ∫ − π π c o s ( n + m ) x d x + ∫ − π π c o s ( n − m ) x d x ] = 1 2 [ 1 n + m s i n ( n + m ) x ∣ − π π ) + 1 n − m s i n ( n − m ) x ∣ − π π ) ] = 1 2 [ 1 n + m [ s i n ( n + m ) π − s i n ( − ( n + m ) π ) ] + 1 n − m [ s i n ( n − m ) π − s i n ( − ( n − m ) π ) ] ] 有 s i n ( − α ) = − s i n α , 又有 ∣ s i n k π + α ∣ = ∣ s i n α ∣ , 上式中 α = 0 , s i n ( ( n + m ) ) π = s i n ( ( n − m ) π ) = 0 很容易得出上式为 0 , 即: ∫ − π π c o s ( n x ) c o s ( m x ) d x = 0 \begin{align} & \int_{- \pi}^{\pi} cos (nx) cos (mx) dx \\ &=\frac {1}{2} \left [ \int_{- \pi}^{\pi} cos(n + m)x dx + \int_{- \pi}^{\pi}cos(n-m)x dx \right] \\ & = \frac{1}{2} \left[ \frac{1}{n+m} sin(n+m)x |_{- \pi}^{\pi}) + \frac{1}{n-m} sin(n-m)x |_{- \pi}^{\pi}) \right] \\ & = \frac{1}{2} \left [ \frac{1}{n+m} \left[ sin(n+m) \pi - sin(-(n+m)\pi) \right] + \frac{1}{n-m} \left[ sin(n-m) \pi - sin(-(n-m)\pi) \right] \right ] \\ & 有 sin (-\alpha) = - sin \alpha,又有|sin k \pi + \alpha| = |sin \alpha|,\\ & 上式中\alpha=0, sin ( (n+m)) \pi = sin((n-m) \pi) = 0很容易得出上式为0 , 即: \\ & \int_{- \pi}^{\pi} cos (nx) cos (mx) dx = 0 & \end{align} ∫−ππcos(nx)cos(mx)dx=21[∫−ππcos(n+m)xdx+∫−ππcos(n−m)xdx]=21[n+m1sin(n+m)x∣−ππ)+n−m1sin(n−m)x∣−ππ)]=21[n+m1[sin(n+m)π−sin(−(n+m)π)]+n−m1[sin(n−m)π−sin(−(n−m)π)]]有sin(−α)=−sinα,又有∣sinkπ+α∣=∣sinα∣,上式中α=0,sin((n+m))π=sin((n−m)π)=0很容易得出上式为0,即:∫−ππcos(nx)cos(mx)dx=0
当 m ≠ n m \ne n m=n时,由积化和差公式 s i n α s i n β = 1 2 [ c o s ( α + β ) − c o s ( α − β ) ] sin\alpha sin\beta = \frac{1}{2} [cos(\alpha + \beta) - cos(\alpha - \beta)] sinαsinβ=21[cos(α+β)−cos(α−β)]、复合导数求导、三角函数常用导数公式以及积分性质可证:
∫ − π π s i n ( n x ) s i n ( m x ) d x = 1 2 [ ∫ − π π c o s ( n + m ) x d x − ∫ − π π c o s ( n − m ) x d x ] = 1 2 [ 1 n + m s i n ( n + m ) x ∣ − π π ) − 1 n − m s i n ( n − m ) x ∣ − π π ) ] = 1 2 [ 1 n + m [ s i n ( n + m ) π − s i n ( − ( n + m ) π ) ] − 1 n − m [ s i n ( n − m ) π − s i n ( − ( n − m ) π ) ] ] 有 ∣ s i n ( k π + α ) ∣ = ∣ s i n α ∣ , 上式中 α = 0 , s i n ( ( n + m ) ) π = s i n ( ( n − m ) π ) = 0 很容易得出上式为 0 , 即: ∫ − π π s i n ( n x ) s i n ( m x ) d x = 0 \begin{align} \int_{- \pi}^{\pi} sin (nx) sin (mx) dx &=\frac {1}{2} \left [ \int_{- \pi}^{\pi} cos(n + m)x dx - \int_{- \pi}^{\pi}cos(n-m)x dx \right] \\ & = \frac{1}{2} \left[ \frac{1}{n+m} sin(n+m)x |_{- \pi}^{\pi}) - \frac{1}{n-m} sin(n-m)x |_{- \pi}^{\pi}) \right] \\ & = \frac{1}{2} \left [ \frac{1}{n+m} \left[ sin(n+m) \pi - sin(-(n+m)\pi) \right] - \frac{1}{n-m} \left[ sin(n-m) \pi - sin(-(n-m)\pi) \right] \right ] \\ & 有 | sin (k \pi + \alpha)| = | sin \alpha |, 上式中\alpha=0, sin ( (n+m)) \pi = sin( (n-m) \pi) = 0很容易得出上式为0 , 即: \\ & \int_{- \pi}^{\pi} sin (nx) sin (mx) dx = 0 & \end{align} ∫−ππsin(nx)sin(mx)dx=21[∫−ππcos(n+m)xdx−∫−ππcos(n−m)xdx]=21[n+m1sin(n+m)x∣−ππ)−n−m1sin(n−m)x∣−ππ)]=21[n+m1[sin(n+m)π−sin(−(n+m)π)]−n−m1[sin(n−m)π−sin(−(n−m)π)]]有∣sin(kπ+α)∣=∣sinα∣,上式中α=0,sin((n+m))π=sin((n−m)π)=0很容易得出上式为0,即:∫−ππsin(nx)sin(mx)dx=0
当 m = n m=n m=n时, 由三角函数平方公式 s i n 2 α = 1 2 ( 1 − c o s 2 α ) sin ^ 2 \alpha = \frac{1}{2} (1 - cos 2 \alpha) sin2α=21(1−cos2α)、复合导数求导、三角函数常用导数公式以及积分性质可证:
∫ − π π s i n ( n x ) s i n ( n x ) d x = 1 2 [ ∫ − π π ( 1 − c o s 2 n x ) d x ] = 1 2 [ ∫ − π π 1 d x − ∫ − π π c o s 2 n x d x ] 如果 m = n = 0 ,上式 = 0 ; 如果 m = n ≠ 0 , 可以假定 c o s 2 n x = c o s 0 x c o s 2 n x , 根据三角函数的正交性质,那么右边 ∫ − π π c o s 2 n x d x = 0 ,上式即为 1 2 [ x ∣ − π π ] = 1 2 2 π = π \begin{align} &\int_{-\pi}^{\pi} sin (nx) sin (nx) dx = \frac{1}{2} \left [ \int_{-\pi}^{\pi} (1 - cos2nx) dx \right ] = \frac{1}{2} \left [ \int_{-\pi}^{\pi} 1 dx - \int_{-\pi}^{\pi} cos2nx dx \right ] \\ & 如果m=n=0,上式=0;\\ & 如果m=n \ne 0, 可以假定cos2nx = cos 0x cos 2nx,\\ & 根据三角函数的正交性质,那么右边\int_{-\pi}^{\pi} cos2nx dx = 0 ,上式即为 \\ & \frac{1}{2} \left[ x|_{-\pi}^{\pi}\right] = \frac{1}{2} 2\pi = \pi & \end{align} ∫−ππsin(nx)sin(nx)dx=21[∫−ππ(1−cos2nx)dx]=21[∫−ππ1dx−∫−ππcos2nxdx]如果m=n=0,上式=0;如果m=n=0,可以假定cos2nx=cos0xcos2nx,根据三角函数的正交性质,那么右边∫−ππcos2nxdx=0,上式即为21[x∣−ππ]=212π=π
当 m = n m=n m=n时, 由三角函数平方公式 c o s 2 α = 1 2 ( 1 + c o s 2 α ) cos^ 2 \alpha = \frac{1}{2} (1 + cos 2 \alpha) cos2α=21(1+cos2α)、复合导数求导、三角函数常用导数公式以及积分性质可证:
∫ − π π c o s ( n x ) c o s ( n x ) d x = 1 2 [ ∫ − π π ( 1 + c o s 2 n x ) d x ] = 1 2 [ ∫ − π π 1 d x + ∫ − π π c o s 2 n x d x ] 如果 m = n = 0 ,上式变为 1 2 [ ∫ − π π 2 d x ] = x ∣ − π π = 2 π ; 如果 m = n ≠ 0 , 假定 c o s 2 n x = c o s 0 x c o s 2 n x ,根据三角函数的正交性质, 那么右边 ∫ − π π c o s 2 n x d x = 0 ,上式即为 1 2 [ x ∣ − π π ] = 1 2 2 π = π \begin{align} & \int_{-\pi}^{\pi} cos (nx) cos (nx) dx = \frac{1}{2} \left [ \int_{-\pi}^{\pi} (1 + cos2nx) dx \right ] = \frac{1}{2} \left [ \int_{-\pi}^{\pi} 1 dx + \int_{-\pi}^{\pi} cos2nx dx \right ] \\ & 如果m=n=0,上式变为\frac{1}{2} \left [ \int_{-\pi}^{\pi} 2 dx \right ] = x|_{-\pi}^{\pi} = 2\pi;\\ & 如果m=n \ne 0, 假定cos2nx = cos 0x cos 2nx,根据三角函数的正交性质,\\ & 那么右边\int_{-\pi}^{\pi} cos2nx dx = 0 ,上式即为 \\ & \frac{1}{2} \left[ x|_{-\pi}^{\pi}\right] = \frac{1}{2} 2\pi = \pi & \end{align} ∫−ππcos(nx)cos(nx)dx=21[∫−ππ(1+cos2nx)dx]=21[∫−ππ1dx+∫−ππcos2nxdx]如果m=n=0,上式变为21[∫−ππ2dx]=x∣−ππ=2π;如果m=n=0,假定cos2nx=cos0xcos2nx,根据三角函数的正交性质,那么右边∫−ππcos2nxdx=0,上式即为21[x∣−ππ]=212π=π
当 m = n m=n m=n时, 由积化和差公式 s i n α c o s β = 1 2 [ s i n ( α + β ) + s i n ( α − β ) ] sin \alpha cos \beta = \frac{1}{2} [sin(\alpha + \beta) + sin(\alpha - \beta)] sinαcosβ=21[sin(α+β)+sin(α−β)]、复合导数求导、三角函数常用导数公式以及积分性质可证:
∫ − π π s i n ( n x ) c o s ( n x ) d x = ∫ − π π c o s ( n x ) s i n ( n x ) d x = 1 2 [ ∫ − π π s i n 2 n x d x + ∫ − π π s i n 0 x d x ] 当 m = n = 0 时,左边变为 ∫ − π π 0 d x = 0 ,右边计算也为 0 ,所以 ∫ − π π s i n ( n x ) c o s ( n x ) d x = 0 当 m = n ≠ 0 时,左边可以看作 ∫ − π π c o s 0 x s i n 2 n x d x = 0 ,所以 ∫ − π π s i n ( n x ) c o s ( n x ) d x = 0 \begin{align} & \int_{- \pi}^{\pi} sin (nx) cos (nx) dx = \int_{- \pi}^{\pi} cos (nx) sin (nx) dx =\frac {1}{2} \left [ \int_{- \pi}^{\pi} sin2nx dx + \int_{- \pi}^{\pi}sin0x dx \right] \\ & 当m=n=0时,左边变为\int_{- \pi}^{\pi} 0 dx = 0,右边计算也为0,所以 \int_{- \pi}^{\pi} sin (nx) cos (nx) dx = 0 \\ & 当m=n \ne 0时, 左边可以看作\int_{- \pi}^{\pi} cos0x sin 2nxdx = 0,所以 \int_{- \pi}^{\pi} sin (nx) cos (nx) dx = 0 & \end{align} ∫−ππsin(nx)cos(nx)dx=∫−ππcos(nx)sin(nx)dx=21[∫−ππsin2nxdx+∫−ππsin0xdx]当m=n=0时,左边变为∫−ππ0dx=0,右边计算也为0,所以∫−ππsin(nx)cos(nx)dx=0当m=n=0时,左边可以看作∫−ππcos0xsin2nxdx=0,所以∫−ππsin(nx)cos(nx)dx=0
总结如下:
∫ − π π s i n ( n x ) c o s ( m x ) d x = 0 ∫ − π π c o s ( n x ) s i n ( m x ) d x = 0 ∫ − π π c o s ( n x ) c o s ( m x ) d x = { 0 , m ≠ n 2 π , m = n = 0 π m = n ≠ 0 ∫ − π π s i n ( n x ) s i n ( m x ) d x = { 0 , m ≠ n 或 m = n = 0 π m = n ≠ 0 \begin{align} & \int_{- \pi}^{\pi} sin (nx) cos (mx) dx = 0 \\ & \int_{- \pi}^{\pi} cos (nx) sin (mx) dx = 0 \\ & \int_{- \pi}^{\pi} cos (nx) cos (mx) dx = \left\{\begin{matrix} 0, & m \ne n \\ 2 \pi, & m = n =0 \\ \pi & m = n \ne 0 \end{matrix}\right.\\ & \int_{- \pi}^{\pi} sin (nx) sin (mx) dx = \left\{\begin{matrix} 0, & m \ne n 或 m=n=0\\ \pi & m = n \ne 0 \end{matrix}\right. & \end{align} ∫−ππsin(nx)cos(mx)dx=0∫−ππcos(nx)sin(mx)dx=0∫−ππcos(nx)cos(mx)dx=⎩ ⎨ ⎧0,2π,πm=nm=n=0m=n=0∫−ππsin(nx)sin(mx)dx={0,πm=n或m=n=0m=n=0
相关文章:

【傅里叶级数与傅里叶变换】数学推导——1、基础知识点回顾及[Part1:三角函数的正交性]介绍
文章内容来自DR_CAN关于傅里叶变换的视频,本篇文章提供了一些基础知识点,比如三角函数常用的导数、三角函数换算公式等。 文章全部链接: 基础知识点 Part1:三角函数系的正交性 Part2:T2π的周期函数的傅里叶级数展开 P…...
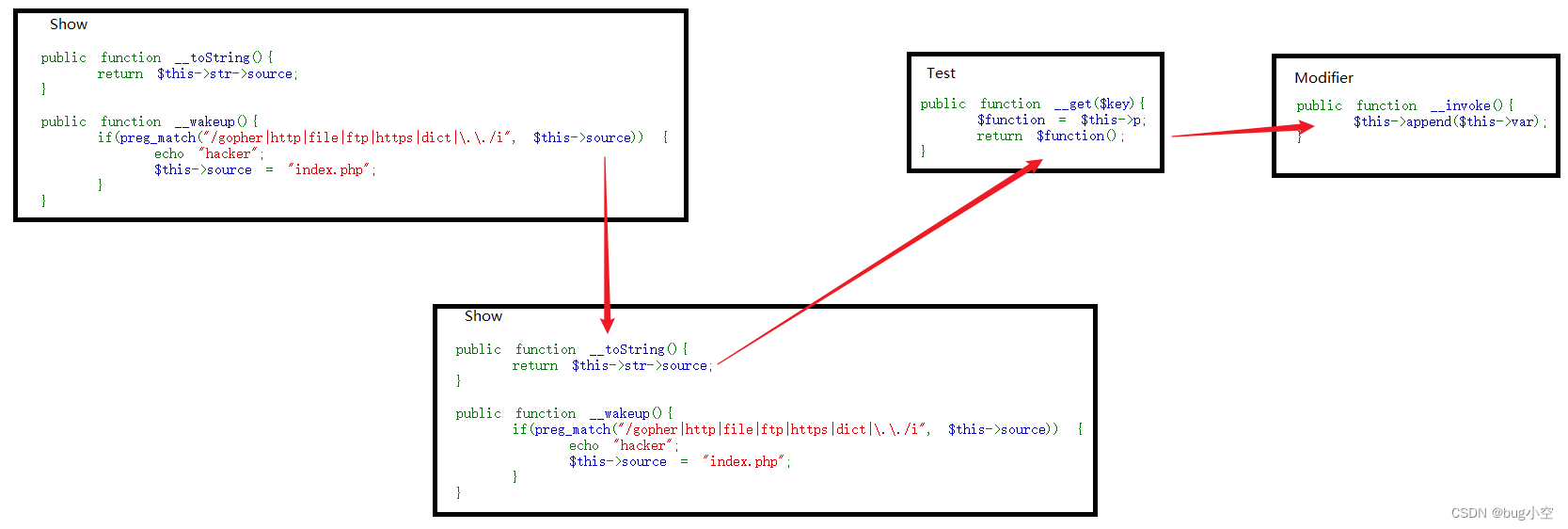
BUUCTF [MRCTF2020]Ezpop解题思路
题目代码 Welcome to index.php <?php //flag is in flag.php //WTF IS THIS? //Learn From https://ctf.ieki.xyz/library/php.html#%E5%8F%8D%E5%BA%8F%E5%88%97%E5%8C%96%E9%AD%94%E6%9C%AF%E6%96%B9%E6%B3%95 //And Crack It! class Modifier {protected $var;publi…...
【IMX6ULL驱动开发学习】07.驱动程序分离的思想之平台总线设备驱动模型和设备树
一、驱动程序分离的思想 【IMX6ULL驱动开发学习】05.字符设备驱动开发模板(包括读写函数、poll机制、异步通知、定时器、中断、自动创建设备节点和环形缓冲区)_阿龙还在写代码的博客-CSDN博客 之前编写驱动程序的代码存在不少弊端:移植性差…...

深度学习中的python语法笔记总结
解释 torch中的 .clamp(min0) 在PyTorch中,torch.clamp将张量中的元素限制在指定的范围内。 torch.clamp(min0)会将张量中的每个元素与0进行比较,并将小于0的元素替换为0。其他大于等于0的元素则保持不变。 clamp函数原理 def clamp(x, lower, upper)…...
Reids 的整合使用
大家好 , 我是苏麟 , 今天带来强大的Redis . REmote DIctionary Server(Redis) 是一个由 Salvatore Sanfilippo 写的 key-value 存储系统,是跨平台的非关系型数据库。 Redis 是一个开源的使用 ANSI C 语言编写、遵守 BSD 协议、支持网络、可基于内存、分布式、可选…...
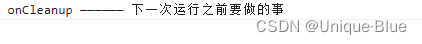
Vue3 —— watchEffect 高级侦听器
该文章是在学习 小满vue3 课程的随堂记录示例均采用 <script setup>,且包含 typescript 的基础用法 前言 Vue3 中新增了一种特殊的监听器 watchEffect,它的类型是: function watchEffect(effect: (onCleanup: OnCleanup) > void,o…...

Java异步子线程读取主线程参数的若干好玩场景
在开发过程中,我们难免会因为性能、实时响应等,需要异步处理的一些事务,并且在子线程中有时我们还需要获取主线程相关的参数。下面有若干方案可以实现上述场景,但会出现一定的问题。 场景1-基础场景 在主线程中开启子线程&#x…...

Android 视频开发
在 Android 平台上进行视频开发,您需要掌握以下关键知识点,以确保能够成功地开发和调试视频应用程序: Android视频架构: 了解 Android 的视频系统架构,包括视频捕获、编码、解码、渲染和显示等。 视频格式和编解码&am…...
【计算机网络篇】UDP协议
✅作者简介:大家好,我是小杨 📃个人主页:「小杨」的csdn博客 🐳希望大家多多支持🥰一起进步呀! UDP协议 1,UDP 简介 UDP(User Datagram Protocol)是一种无连…...

LeetCode 2682. 找出转圈游戏输家
【LetMeFly】2682.找出转圈游戏输家 力扣题目链接:https://leetcode.cn/problems/find-the-losers-of-the-circular-game/ n 个朋友在玩游戏。这些朋友坐成一个圈,按 顺时针方向 从 1 到 n 编号。从第 i 个朋友的位置开始顺时针移动 1 步会到达第 (i …...
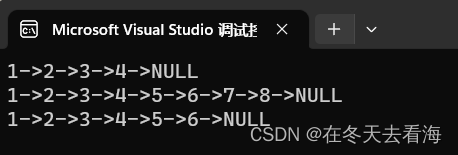
数据结构单链表
单链表 1 链表的概念及结构 概念:链表是一种物理存储结构上非连续、非顺序的存储结构,数据元素的逻辑顺序是通过链表中的指针链 接次序实现的 。 在我们开始讲链表之前,我们是写了顺序表,顺序表就是类似一个数组的东西࿰…...
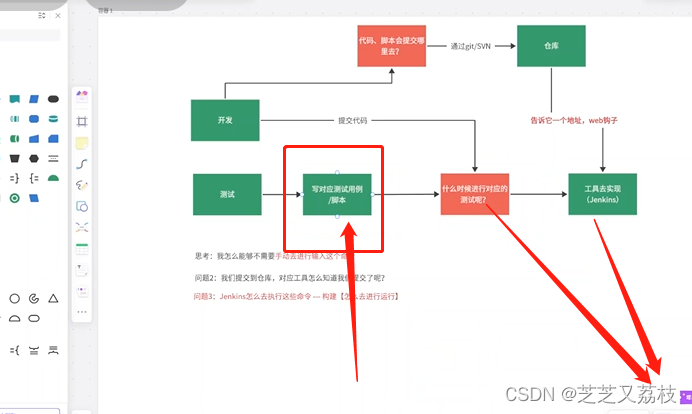
自定义WEB框架结合Jenkins实现全自动测试
自定义WEB框架结合Jenkins实现全自动测试 allure生成 allure生成 1.allure–纯命令运行 -固定的–稍微记住对应的单词即可。2 安装,2个步骤: 1.下载allure包,然后配置环境变量。 https://github.com/allure-framework/allure2/releases/tag/2.22.4 2.在…...

PHP加密与安全的最佳实践
PHP加密与安全的最佳实践 概述 在当今信息时代,数据安全是非常重要的。对于开发人员而言,掌握加密和安全的最佳实践是必不可少的。PHP作为一种常用的后端开发语言,提供了许多功能强大且易于使用的加密和安全性相关函数和类。本文将介绍一些P…...
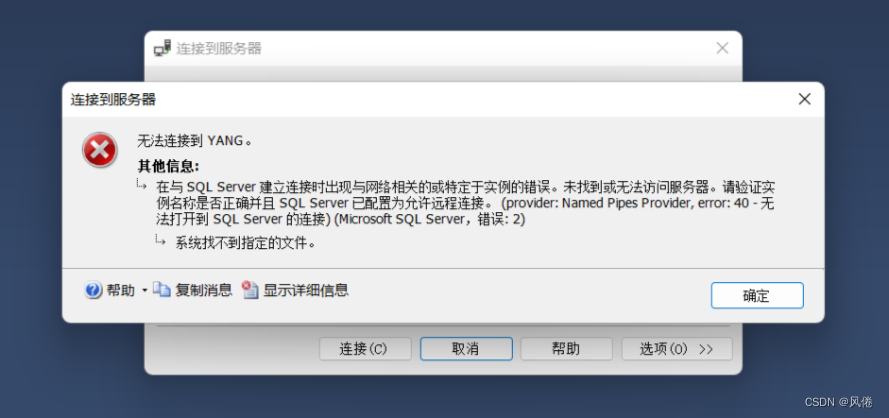
SQL Server数据库无法连接
问题如下: 原因:sql server服务器未开启 解决方法:以管理员身份打开cmd,输入:net start mssqlserver。...

videojs 播放视频
背景:在项目中使用第三方插件videojs进行播放视频,点击事件更改播放的数据源。 一、视频相关理论 (一)、背景 网络流媒体的呈现形式分为两种:直播点播 (二)、流媒体的3种协议 分类:HTTPHLSRTMP定义:基于HTTP的流媒体…...

vue强制刷新变量
在前端开发中,我们经常需要变量的值实时响应到界面上。Vue就是一个非常强大的前端框架,它的数据绑定能够非常好地实现变量与界面的同步更新。但是有时候,我们需要强制刷新某个变量的值,以便界面能及时地反映出它的变化。本文将介绍…...

[QCA6174]QCA6174 5G WiFi DFS处理逻辑分析及雷达误检率高优化规避
DFS认证信息 WIFI DFS测试要求 Master设备需要测试的项目 4.6.2.1 Channel Availability Check 信道可用性检查 定义其作为雷达脉冲检测机制,当雷达脉冲出现时所占用的信道需要能被设备检测到已经被占用。当相关信道未被占用时,这些信道被称为Avaliable Channel可用信道 …...
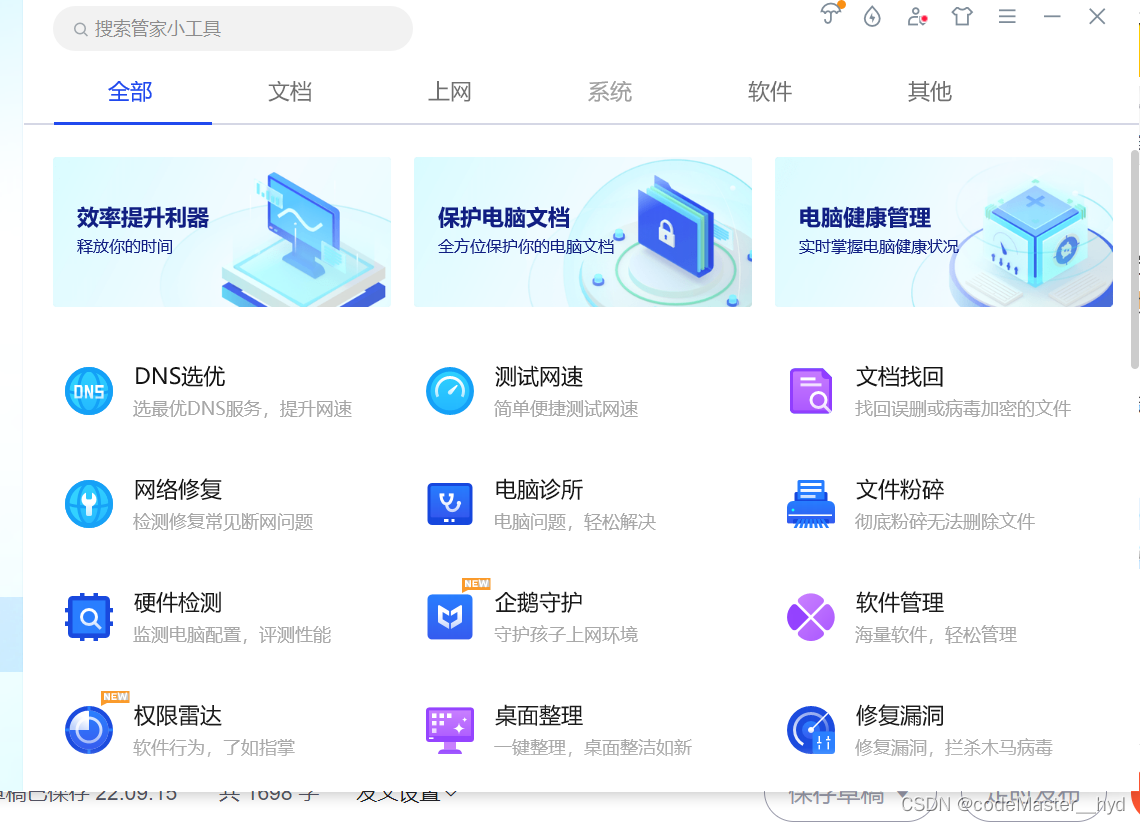
预防SQL漏洞注入和规避网络攻击
前言: 虽然近些年SQL注入已经被各类的安全开发框架规避了绝大部分,但SQL注入作为一种最原始的攻击手段之一,破坏力仍然十分强大,因为它直捣黄龙数据中心。所以未雨绸缪,各位不可不重视。 预防SQL注入方法措施: 服务器…...
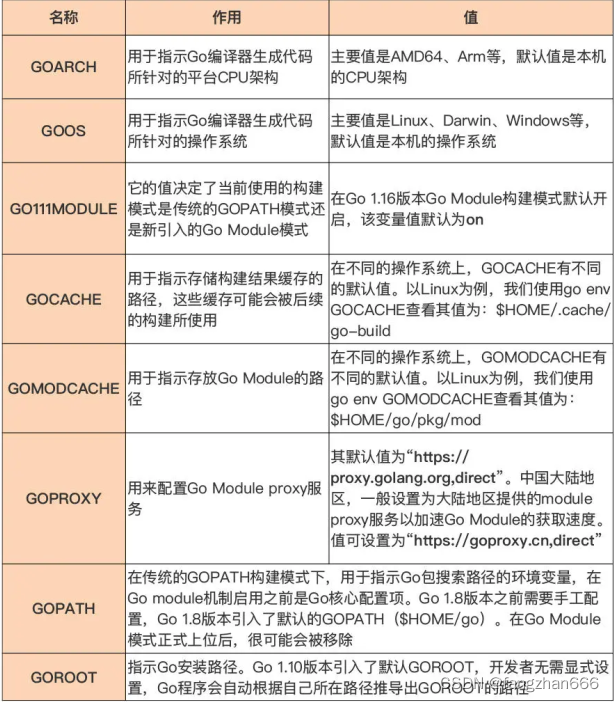
《Go 语言第一课》课程学习笔记(一)
配好环境:选择一种最适合你的 Go 安装方法 选择 Go 版本 一般情况下,建议采用最新版本。因为 Go 团队发布的 Go 语言稳定版本的平均质量一直是很高的,少有影响使用的重大 bug。可以根据不同实际项目需要或开源社区的情况使用不同的版本。 有…...
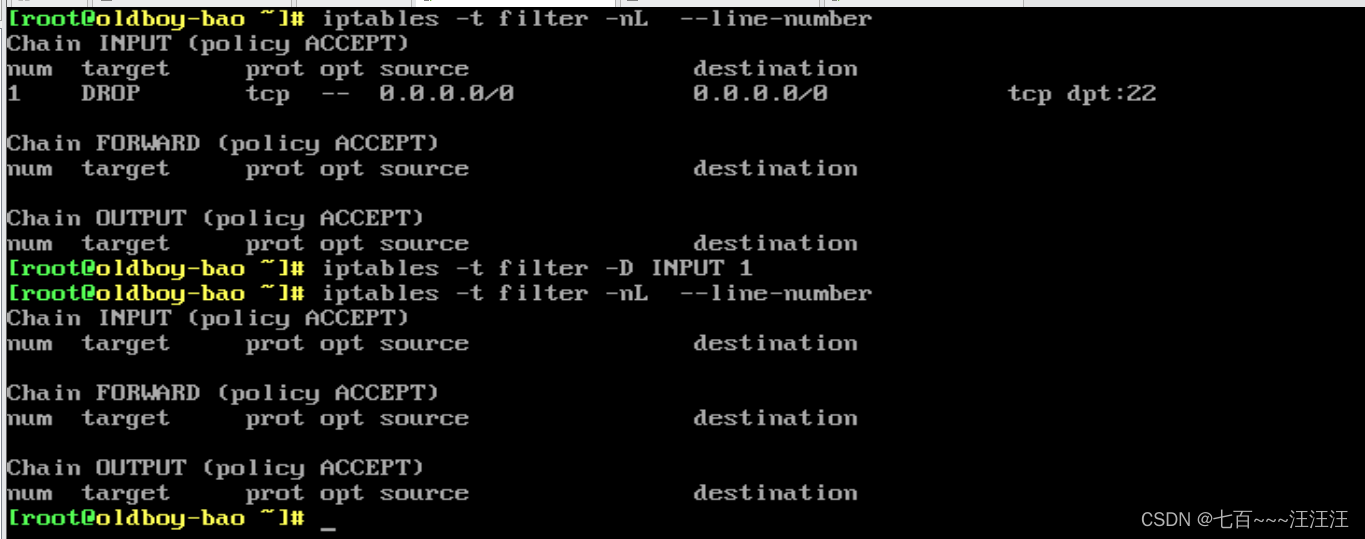
网络安全 Day29-运维安全项目-iptables防火墙
iptables防火墙 1. 防火墙概述2. 防火墙2.1 防火墙种类及使用说明2.2 必须熟悉的名词2.3 iptables 执行过程※※※※※2.4 表与链※※※※※2.4.1 简介2.4.2 每个表说明2.4.2.1 filter表 :star::star::star::star::star:2.4.2.2 nat表 2.5 环境准备及命令2.6 案例01:…...
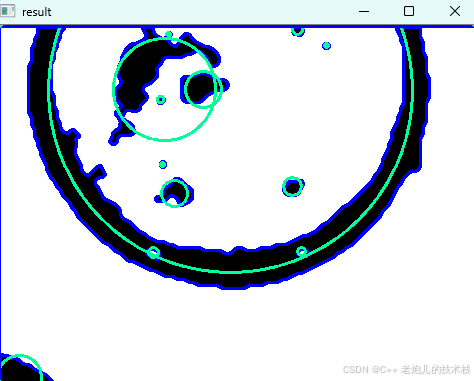
利用最小二乘法找圆心和半径
#include <iostream> #include <vector> #include <cmath> #include <Eigen/Dense> // 需安装Eigen库用于矩阵运算 // 定义点结构 struct Point { double x, y; Point(double x_, double y_) : x(x_), y(y_) {} }; // 最小二乘法求圆心和半径 …...
)
uniapp 对接腾讯云IM群组成员管理(增删改查)
UniApp 实战:腾讯云IM群组成员管理(增删改查) 一、前言 在社交类App开发中,群组成员管理是核心功能之一。本文将基于UniApp框架,结合腾讯云IM SDK,详细讲解如何实现群组成员的增删改查全流程。 权限校验…...

vscode里如何用git
打开vs终端执行如下: 1 初始化 Git 仓库(如果尚未初始化) git init 2 添加文件到 Git 仓库 git add . 3 使用 git commit 命令来提交你的更改。确保在提交时加上一个有用的消息。 git commit -m "备注信息" 4 …...

【HTML-16】深入理解HTML中的块元素与行内元素
HTML元素根据其显示特性可以分为两大类:块元素(Block-level Elements)和行内元素(Inline Elements)。理解这两者的区别对于构建良好的网页布局至关重要。本文将全面解析这两种元素的特性、区别以及实际应用场景。 1. 块元素(Block-level Elements) 1.1 基本特性 …...

OPENCV形态学基础之二腐蚀
一.腐蚀的原理 (图1) 数学表达式:dst(x,y) erode(src(x,y)) min(x,y)src(xx,yy) 腐蚀也是图像形态学的基本功能之一,腐蚀跟膨胀属于反向操作,膨胀是把图像图像变大,而腐蚀就是把图像变小。腐蚀后的图像变小变暗淡。 腐蚀…...

听写流程自动化实践,轻量级教育辅助
随着智能教育工具的发展,越来越多的传统学习方式正在被数字化、自动化所优化。听写作为语文、英语等学科中重要的基础训练形式,也迎来了更高效的解决方案。 这是一款轻量但功能强大的听写辅助工具。它是基于本地词库与可选在线语音引擎构建,…...
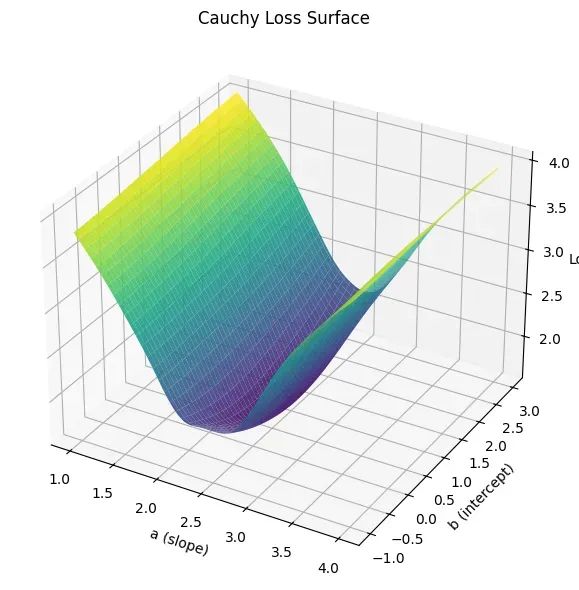
让回归模型不再被异常值“带跑偏“,MSE和Cauchy损失函数在噪声数据环境下的实战对比
在机器学习的回归分析中,损失函数的选择对模型性能具有决定性影响。均方误差(MSE)作为经典的损失函数,在处理干净数据时表现优异,但在面对包含异常值的噪声数据时,其对大误差的二次惩罚机制往往导致模型参数…...

【分享】推荐一些办公小工具
1、PDF 在线转换 https://smallpdf.com/cn/pdf-tools 推荐理由:大部分的转换软件需要收费,要么功能不齐全,而开会员又用不了几次浪费钱,借用别人的又不安全。 这个网站它不需要登录或下载安装。而且提供的免费功能就能满足日常…...

Web中间件--tomcat学习
Web中间件–tomcat Java虚拟机详解 什么是JAVA虚拟机 Java虚拟机是一个抽象的计算机,它可以执行Java字节码。Java虚拟机是Java平台的一部分,Java平台由Java语言、Java API和Java虚拟机组成。Java虚拟机的主要作用是将Java字节码转换为机器代码&#x…...
Python 实现 Web 静态服务器(HTTP 协议)
目录 一、在本地启动 HTTP 服务器1. Windows 下安装 node.js1)下载安装包2)配置环境变量3)安装镜像4)node.js 的常用命令 2. 安装 http-server 服务3. 使用 http-server 开启服务1)使用 http-server2)详解 …...
