AtCoder Beginner Contest 292——A-E题讲解
蒟蒻来讲题,还望大家喜。若哪有问题,大家尽可提!
Hello, 大家好哇!本初中生蒟蒻讲解一下AtCoder Beginner Contest 292这场比赛的A-E题!
===========================================================================================
A题
原题
Problem Statement
You are given a string SSS consisting of lowercase English letters.
Uppercase each character of SSS and print the resulting string TTT.
Constraints
SSS is a string consisting of lowercase English letters whose length is between 111 and 100100100, inclusive.
Input
The input is given from Standard Input in the following format:
SSS
Output
Print TTT.
Sample Input 1
abc
Sample Output 1
ABC
Uppercase each character of abc, and you have ABC.
Sample Input 2
a
Sample Output 2
A
Sample Input 3
abcdefghjiklnmoqprstvuwxyz
Sample Output 3
ABCDEFGHJIKLNMOQPRSTVUWXYZ
思路
水题一道,不多写啦!
代码
/*
------------------Welcome to Your Code--------------
Name:
Contest:AtCoder Beginner Contest 292
Wishes:AK!
------------------Start Writing!!!------------------
*/
#include <iostream>
#define endl '\n'
#define pb(i) push_back(i)using namespace std;inline int read()
{int w = 1, s = 0;char c = getchar();while (c < '0' || c > '9'){if (c == '-') w = -1;c = getchar();}while (c >= '0' && c <= '9') s = s * 10 + c - '0', c = getchar();return w * s;
}int main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);string s;cin >> s;for (auto c : s)cout << char(c - 'a' + 'A');return 0;
}
B题
原题
Problem Statement
NNN players numbered 111 to NNN will play a soccer game.
When a player commits an offense, that player will receive a yellow card or a red card.
A player who satisfies one of the following conditions will be removed from the game.
Accumulates two yellow cards.
Receives a red card.
Once a player is removed, that player will no longer receive any cards.
You will watch this game. Initially, the players have not received any cards.
There will be QQQ events. Correctly answer the questions asked in the events.
There are three kinds of events, which are given in the format c x from the input, where ccc is 111, 222, or 333. The events are as follows.
1 x: Player xxx receives a yellow card.
2 x: Player xxx receives a red card.
3 x: You are asked whether player xxx has been removed from the game. Answer Yes or No.
Constraints
1≤N≤1001 \leq N \leq 1001≤N≤100
1≤Q≤1001 \leq Q \leq 1001≤Q≤100
1≤x≤N1 \leq x \leq N1≤x≤N in all events.
There is at least one event of the third kind.
A player who has been removed will no longer receive any cards.
All values in the input are integers.
Input
The input is given from Standard Input in the following format, where eventi\text{event}_ieventi denotes the iii-th event.
NNN QQQ
event1\text{event}_1event1
event2\text{event}_2event2
⋮\vdots⋮
eventQ\text{event}_QeventQ
Each event is in one of the following formats:
1 xxx
2 xxx
3 xxx
Output
Print XXX lines, where XXX is the number of events of the third kind in the input.
The iii-th line should contain Yes if, for the iii-th event of the third kind, player xxx has been removed from the game, and No otherwise.
Sample Input 1
3 9
3 1
3 2
1 2
2 1
3 1
3 2
1 2
3 2
3 3
Sample Output 1
No
No
Yes
No
Yes
No
Here are all the events in chronological order.
In the 111-st event, you are asked whether player 111 has been removed from the game. Player 111 has not been removed, so you should print No.
In the 222-nd event, you are asked whether player 222 has been removed from the game. Player 222 has not been removed, so you should print No.
In the 333-rd event, player 222 receives a yellow card.
In the 444-th event, player 111 receives a red card and is removed from the game.
In the 555-th event, you are asked whether player 111 has been removed from the game. Player 111 has been removed, so you should print Yes.
In the 666-th event, you are asked whether player 222 has been removed from the game. Player 222 has not been removed, so you should print No.
In the 777-th event, player 222 receives a yellow card and is removed from the game.
In the 888-th event, you are asked whether player 222 has been removed from the game. Player 222 has been removed, so you should print Yes.
In the 999-th event, you are asked whether player 333 has been removed from the game. Player 333 has not been removed, so you should print No.
思路
也比较水,不讲啦,直接看代码吧!
代码
/*
------------------Welcome to Your Code--------------
Name:
Contest:AtCoder Beginner Contest 292
Wishes:AK!
------------------Start Writing!!!------------------
*/
#include <iostream>
#define endl '\n'
#define pb(i) push_back(i)using namespace std;const int N = 1e2 + 10;double st[N];inline int read()
{int w = 1, s = 0;char c = getchar();while (c < '0' || c > '9'){if (c == '-') w = -1;c = getchar();}while (c >= '0' && c <= '9') s = s * 10 + c - '0', c = getchar();return w * s;
}int main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);int n, q;cin >> n >> q;while (q --){int op, x;cin >> op >> x;if (op == 1) st[x] += 0.5;else if (op == 2) st[x] ++;else{cout << (st[x] >= 1 ? "Yes" : "No") << endl;}}return 0;
}
C题
原题
Problem Statement
You are given a positive integer NNN.
Find the number of quadruples of positive integers (A,B,C,D)(A,B,C,D)(A,B,C,D) such that AB+CD=NAB + CD = NAB+CD=N.
Under the constraints of this problem, it can be proved that the answer is at most 9×10189 \times 10^{18}9×1018.
Constraints
2≤N≤2×1052 \leq N \leq 2 \times 10^52≤N≤2×105
NNN is an integer.
Input
The input is given from Standard Input in the following format:
NNN
Output
Print the answer.
Sample Input 1
4
Sample Output 1
8
Here are the eight desired quadruples.
(A,B,C,D)=(1,1,1,3)(A,B,C,D)=(1,1,1,3)(A,B,C,D)=(1,1,1,3)
(A,B,C,D)=(1,1,3,1)(A,B,C,D)=(1,1,3,1)(A,B,C,D)=(1,1,3,1)
(A,B,C,D)=(1,2,1,2)(A,B,C,D)=(1,2,1,2)(A,B,C,D)=(1,2,1,2)
(A,B,C,D)=(1,2,2,1)(A,B,C,D)=(1,2,2,1)(A,B,C,D)=(1,2,2,1)
(A,B,C,D)=(1,3,1,1)(A,B,C,D)=(1,3,1,1)(A,B,C,D)=(1,3,1,1)
(A,B,C,D)=(2,1,1,2)(A,B,C,D)=(2,1,1,2)(A,B,C,D)=(2,1,1,2)
(A,B,C,D)=(2,1,2,1)(A,B,C,D)=(2,1,2,1)(A,B,C,D)=(2,1,2,1)
(A,B,C,D)=(3,1,1,1)(A,B,C,D)=(3,1,1,1)(A,B,C,D)=(3,1,1,1)
Sample Input 2
292
Sample Output 2
10886
Sample Input 3
19876
Sample Output 3
2219958
思路
这道题我们可以枚举ABABAB,假设为iii,那么CDCDCD就是n−in - in−i,之后先计算约数的个数,如果是个奇数,那么就说明中间有个数是相同的,所以要特判。最后答案就把各种情况列出来相加(令nab为AB的约数的个数,ncd为CD的约数的个数):
- 若nab,ncd都为偶数,则:此时的方案数=nab×ncd=nab\times ncd=nab×ncd
- 若nab,ncd都为奇数,则:此时的方案数=(nab−1)(ncd−1)+na+nc+1=(nab-1)(ncd-1)+na+nc+1=(nab−1)(ncd−1)+na+nc+1,可能有点难理解,自己推一下就可以啦!(若还是不懂,联系我即可)
- 若nab为偶数,ncd为奇数,则:此时的方案数=nab(ncd−1)+nc∗2=nab(ncd-1)+nc*2=nab(ncd−1)+nc∗2
最后,把所有的方案数相加,就是最终的答案了!
代码(时间复杂度:O(NN)O(N\sqrt{N})O(NN))
/*
------------------Welcome to Your Code--------------
Name:
Contest:AtCoder Beginner Contest 292
Wishes:AK!
------------------Start Writing!!!------------------
*/
#include <iostream>
#include <cmath>
#define endl '\n'
#define pb(i) push_back(i)using namespace std;int res;inline int read()
{int w = 1, s = 0;char c = getchar();while (c < '0' || c > '9'){if (c == '-') w = -1;c = getchar();}while (c >= '0' && c <= '9') s = s * 10 + c - '0', c = getchar();return w * s;
}int get_divides(int n) //求约数的个数
{int counts = 0;for (int i = 1; i * i <= n; i++){if (n % i == 0){if (i * i == n) counts++;elsecounts += 2;}}return counts;
}int main()
{int n;cin >> n;long long ans = 0;for (int i = 1; i < n; i ++){int ab = i, cd = n - i;int na,nab = get_divides(ab);bool fab= 0;int nc,ncd = get_divides(cd);bool fcd= 0;if(nab % 2) na = nab -1,fab =1;else na = nab;if(ncd % 2) nc = ncd -1,fcd =1;else nc = ncd;if(fab ){if(fcd){ans += na * nc + na + nc + 1;}else{ans += na * nc + nc * 2 ;}}else ans += na * nc;}cout << ans << endl;return 0;
}
D题
原题
Problem Statement
You are given an undirected graph with NNN vertices numbered 111 to NNN and MMM edges numbered 111 to MMM. Edge iii connects vertex uiu_iui and vertex viv_ivi.
Determine whether every connected component in this graph satisfies the following condition.
The connected component has the same number of vertices and edges.
Notes
An undirected graph is a graph with edges without direction.
A subgraph of a graph is a graph formed from a subset of vertices and edges of that graph.
A graph is connected when one can travel between every pair of vertices in the graph via edges.
A connected component is a connected subgraph that is not part of any larger connected subgraph.
Constraints
1≤N≤2×1051 \leq N \leq 2 \times 10^51≤N≤2×105
0≤M≤2×1050 \leq M \leq 2 \times 10^50≤M≤2×105
1≤ui≤vi≤N1 \leq u_i \leq v_i \leq N1≤ui≤vi≤N
All values in the input are integers.
Input
The input is given from Standard Input in the following format:
NNN MMM
u1u_1u1 v1v_1v1
⋮\vdots⋮
uMu_MuM vMv_MvM
Output
If every connected component satisfies the condition, print Yes; otherwise, print No.
Sample Input 1
3 3
2 3
1 1
2 3
Sample Output 1
Yes
The graph has a connected component formed from just vertex 111, and another formed from vertices 222 and 333.
The former has one edge (edge 222), and the latter has two edges (edges 111 and 333), satisfying the condition.
Sample Input 2
5 5
1 2
2 3
3 4
3 5
1 5
Sample Output 2
Yes
Sample Input 3
13 16
7 9
7 11
3 8
1 13
11 11
6 11
8 13
2 11
3 3
8 12
9 11
1 11
5 13
3 12
6 9
1 10
Sample Output 3
No
思路
这道题我们就是判断每一个连通块是否点数和边数相等,所以我们可以用**洪水填充(Flood Fill)**算法,当然可以用DFS做!
代码
/*
------------------Welcome to Your Code--------------
Name:
Contest:AtCoder Beginner Contest 292
Wishes:AK!
------------------Start Writing!!!------------------
*/
#include <iostream>
#include <vector>using namespace std;const int N = 2e5 + 10;int n, m, edge, vert;
vector<int> fg[N];
bool ft[N];inline int read()
{int w = 1, s = 0;char c = getchar();while (c < '0' || c > '9'){if (c == '-') w = -1;c = getchar();}while (c >= '0' && c <= '9') s = s * 10 + c - '0', c = getchar();return w * s;
}void dfs(int u)
{for (auto c : fg[u]){if (!ft[c]){ft[c] = 1;edge ++, vert ++;dfs(c);}else edge ++;}
}int main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);cin >> n >> m;int x, y;for (int i = 1; i <= m; i ++)cin >> x >> y, fg[x].push_back(y), fg[y].push_back(x);for (int i = 1; i <= n; i ++)if (!ft[i]){vert = 1;edge = 0;ft[i] = 1;dfs(i); //找当前连通块if (edge / 2 != vert) //因为我是用的无向边,所以真正的边数要除以2{cout << "No" << endl;return 0;}}cout << "Yes" << endl;return 0;
}
E题
原题
Problem Statement
You are given a simple directed graph with NNN vertices numbered 111 to NNN and MMM edges numbered 111 to MMM. Edge iii is a directed edge from vertex uiu_iui to vertex viv_ivi.
You may perform the following operation zero or more times.
Choose distinct vertices xxx and yyy such that there is no directed edge from vertex xxx to vertex yyy, and add a directed edge from vertex xxx to vertex yyy.
Find the minimum number of times you need to perform the operation to make the graph satisfy the following condition.
For every triple of distinct vertices aaa, bbb, and ccc, if there are directed edges from vertex aaa to vertex bbb and from vertex bbb to vertex ccc, there is also a directed edge from vertex aaa to vertex ccc.
Constraints
3≤N≤20003 \leq N \leq 20003≤N≤2000
0≤M≤20000 \leq M \leq 20000≤M≤2000
1≤ui,vi≤N1 \leq u_i ,v_i \leq N1≤ui,vi≤N
ui≠viu_i \neq v_iui=vi
(ui,vi)≠(uj,vj)(u_i,v_i) \neq (u_j,v_j)(ui,vi)=(uj,vj) if i≠ji \neq ji=j.
All values in the input are integers.
Input
The input is given from Standard Input in the following format:
NNN MMM
u1u_1u1 v1v_1v1
⋮\vdots⋮
uMu_MuM vMv_MvM
Output
Print the answer.
Sample Input 1
4 3
2 4
3 1
4 3
Sample Output 1
3
Initially, the condition is not satisfied because, for instance, for vertices 222, 444, and 333, there are directed edges from vertex 222 to vertex 444 and from vertex 444 to vertex 333, but not from vertex 222 to vertex 333.
You can make the graph satisfy the condition by adding the following three directed edges:
one from vertex 222 to vertex 333,
one from vertex 222 to vertex 111, and
one from vertex 444 to vertex 111.
On the other hand, the condition cannot be satisfied by adding two or fewer edges, so the answer is 333.
Sample Input 2
292 0
Sample Output 2
0
Sample Input 3
5 8
1 2
2 1
1 3
3 1
1 4
4 1
1 5
5 1
Sample Output 3
12
思路
这道题我们完全可以把每个点能到的点的个数都加起来,在减去原来就有的边数,就是我们没有建出来的边数,所以求出这个没有建的边数即可!
代码
/*
------------------Welcome to Your Code--------------
Name:
Contest:AtCoder Beginner Contest 292
Wishes:AK!
------------------Start Writing!!!------------------
*/
#pragma GCC optimize(2)
#pragma GCC optimize(3,"Ofast","inline")
#include <iostream>
#include <vector>
#include <cstring>
#include <queue>
#define endl '\n'
#define pb(i) push_back(i)using namespace std;const int N = 2e3 + 10;int n, m;
bool edge[N][N];
vector<int> g[N];
int x, y;
int turn[N];
bool st[N];inline int read()
{int w = 1, s = 0;char c = getchar();while (c < '0' || c > '9'){if (c == '-') w = -1;c = getchar();}while (c >= '0' && c <= '9') s = s * 10 + c - '0', c = getchar();return w * s;
}int bfs(int u)
{memset(st, 0, sizeof st);queue<int> q;q.push(u);st[u] = 1;int res = 0;while (q.size()){int t = q.front();q.pop();for (auto c : g[t])if (!st[c]){q.push(c);res ++;st[c] = 1;}}return res;
}int main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);cin >> n >> m;for (int i = 1; i <= m; i ++)cin >> x >> y, g[x].pb(y), edge[x][y] = 1;int ans = 0;for (int i = 1; i <= n; i ++)ans += bfs(i);cout << ans - m << endl;return 0;
}
今天就到这里了!
大家有什么问题尽管提,我都会尽力回答的!
吾欲您伸手,点的小赞赞。吾欲您喜欢,点得小关注!
相关文章:

AtCoder Beginner Contest 292——A-E题讲解
蒟蒻来讲题,还望大家喜。若哪有问题,大家尽可提! Hello, 大家好哇!本初中生蒟蒻讲解一下AtCoder Beginner Contest 292这场比赛的A-E题! A题 原题 Problem Statement You are given a string SSS consisting of lo…...

(蓝桥真题)最长不下降子序列(权值线段树)
样例输入: 5 1 1 4 2 8 5 样例输出: 4 分析:看到这种对其中连续k个数进行修改的我们就应该想到答案是由三部分组成,因为求的是最长不下降子序列,那么我们可以找到一个最合适的断点i,使得答案是由区间[1…...

数据类型及参数传递
1.数据类型 java中的基本数据类型: 数值型: 整数型:byte short long int 浮点型:float double 布尔型: boolean字符串: char java中的引用数据类型: 数组(array) 类(class…...

永春堂1300系统开发|解析永春堂1300模式商城的五大奖项
电商平台竞争越来越激烈,各种营销方式也是层出不穷,其中永春堂1300营销模式,以其无泡沫和自驱动性强等特点风靡一时。在这套模式中,虽然单型价格差异较大,但各种奖励的设计,巧妙的兼顾了平台和所有会员的利…...

最近一年我都干了什么——反思!!
过去一年不管是学习方式还是心态上都和以往有了许多不同的地方,比较昏昏沉沉。最近慢慢找到状态了,就想赶紧记录下来。 学习 在学习新技术的过程中开始飘了,总感觉有了一些开发经验后就觉得什么都不用记,知道思路就行遇到了现场百…...
save 和 export 命令的区别)
Docker学习(十七)save 和 export 命令的区别
Docker 中有两个命令可以将镜像导出为本地文件系统中的 tar 文件:docker save 和 docker export。尽管它们的作用类似,但它们之间有一个重要的区别。 1.使用方式的不同: docker save 的使用示例: docker save -o test.tar image…...
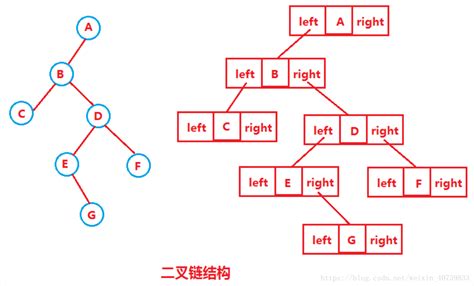
【数据结构初阶】详解“树”
目录 前言 1.树概念及结构 (1)树的概念 (2)树的名词介绍 (3)树的表示 编辑 2.二叉树概念及结构 (1)概念 (2)特殊的二叉树 (3࿰…...

20230304 CF855 div3 vp
Dashboard - Codeforces Round 855 (Div. 3) - Codeforces呃呃,评价是,毫无进步呃呃呃呃呃呃呃呃呃呃呃呃呃呃呃呃呃该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训了该加训…...
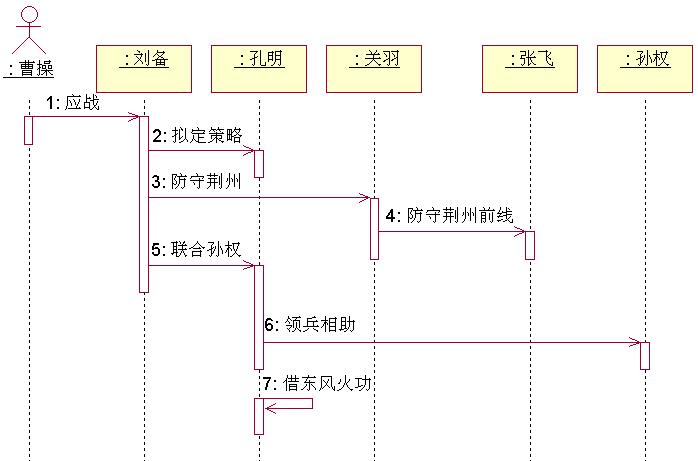
UML 时序图
时序图(Sequence Diagram)是显示对象之间交互的图,是按时间顺序排列的。 时序图中显示的是参与交互的对象及其对象之间消息交互的顺序。 时序图包括的建模元素主要有:对象(Actor)、生命线(Lif…...
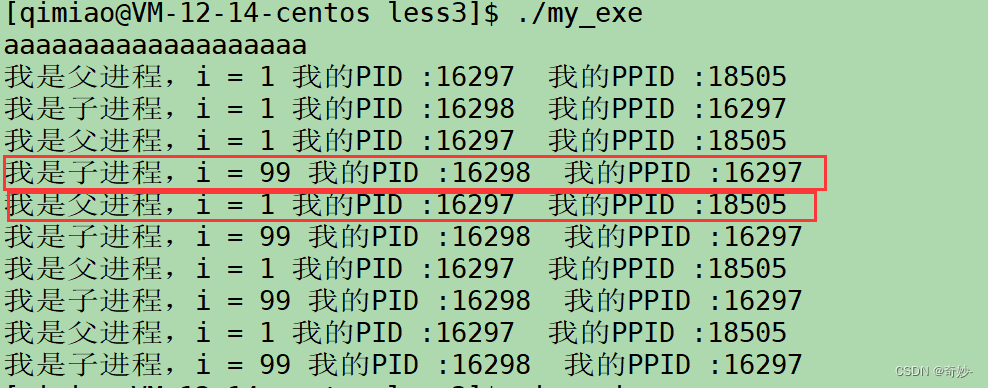
详解进程 及 探查进程
进程的概念PCB是什么task_struct的作用如何执行进程进程的探查什么是bashps命令的使用(查看进程)创建进程探究父子进程进程的概念 简而言之,进程就是正在在执行的程序 之前说过,程序执行的第一步Windows是双击程序Linux是 ./ &a…...
汇编相关问题
汇编语言期末复习题DX:单项选择题 DU:多项选择题 TK:填空题 MC:名词解释 v JD:简答题 CXFX:程序分析题 CXTK:程序填空题 BC:编程题第1章:基础知识1、在汇编语言程序的开发…...

华为OD机试Golang解题 - 火星文计算 2 | 包含思路
华为Od必看系列 华为OD机试 全流程解析+经验分享,题型分享,防作弊指南)华为od机试,独家整理 已参加机试人员的实战技巧华为od 2023 | 什么是华为od,od 薪资待遇,od机试题清单华为OD机试真题大全,用 Python 解华为机试题 | 机试宝典文章目录 华为Od必看系列使用说明本期题目…...

成功解决configure: error: the HTTP rewrite module requires the PCRE library
文章目录 前言问题复现问题解决思考环节总结前言 大家好,我是沐风晓月,本专栏是记录日常实验中的所有疑难杂症,教程的安装,程序的bug,甚至各类报错,如果你也遇到了困惑和问题,欢迎与我一起交流学习。 另外不要解决完问题就结束了,思考环节也要好好看看哦。 问题复现…...

UNIX--GDB调试
通常,在为调试而编译时,我们会关掉编译器优化选项(-O),并打开调试选项(-g)。另外,-Wall 在尽量不影响程序行为的情况下选项打开所有 warning,也可以发现许多问题,避免一些不必要的 BUG。 GDB 命令-启动、退…...

孤单数算法
1.背景 腾讯终面:孤单的QQ号码怎么找? 问题一:有n个QQ号码,除1个孤单的QQ号码外,其余的QQ号码都是成双成对的,求这个孤单的QQ号码,要求:时间复杂度为O(n), 空间复杂度为O(1). 问题…...

triangulate_object_model_3d算子总结
目录 1.去掉固定方向的点云干扰 2.增加八叉树深度,实现更高细节级别的三角测量 3.腐蚀和膨胀,得到更平滑的点云 1.去掉固定方向的点云干扰 例程:triangulate_object_model_3d_xyz_mapping.hdev...
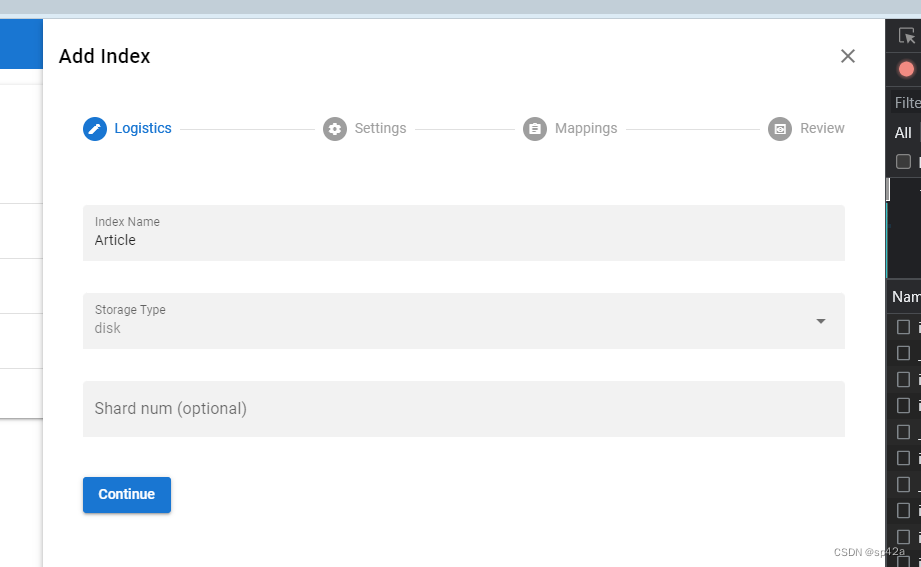
ZincSearch Java 客户端教程
ZincSearch Zinc 简单、强大,不了解的同学可以参见我之前的博客。今天我们这里谈谈 Java 环境如何集成 Zinc 客户端,跟如何使用的。 安装 Zinc 到 Github 的官方 Releases 下载: 我的是 Windows 开发环境,下载 zincsearch_0.4…...
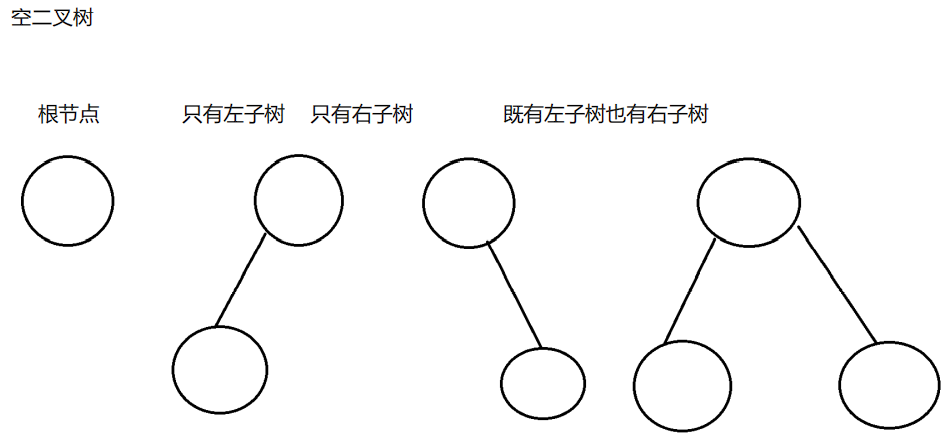
数据结构(一)(嵌入式学习)
数据结构干货总结(一)基础线性表的顺序表示线性表的链式表示单链表双链表循环链表循环单链表循环双链表栈顺序存储链式存储队列队列的定义队列的常见基本操作队列的顺序存储结构顺序队列循环队列队列的链式存储结构树概念二叉树二叉树的创建基础 数据&a…...

合成复用原则-快速理解
什么是合成/聚合复用原则? 合成/聚合复用原则是在一个新的对象里面使用一些已有的对象,使之成为新对象的一部分;新的对象通过向这些对象的委派达到复用已有功能的目的。 简述为:要尽量使用合成/聚合,尽量不要使用继承…...

Scala04 方法与函数
Scala04 方法与函数 Scala 中的也有方法和函数的概念。 Scala中的 方法 是类的一部分。 Scala中的 函数 是一个对象,可以赋值给变量。 在类中定义的函数就是方法 4.1 方法 Scala 中方法 与 Java 中类似,是组成类的一部分 4.1.1 语法结构 格式&#x…...

内存分配函数malloc kmalloc vmalloc
内存分配函数malloc kmalloc vmalloc malloc实现步骤: 1)请求大小调整:首先,malloc 需要调整用户请求的大小,以适应内部数据结构(例如,可能需要存储额外的元数据)。通常,这包括对齐调整,确保分配的内存地址满足特定硬件要求(如对齐到8字节或16字节边界)。 2)空闲…...
)
React Native 开发环境搭建(全平台详解)
React Native 开发环境搭建(全平台详解) 在开始使用 React Native 开发移动应用之前,正确设置开发环境是至关重要的一步。本文将为你提供一份全面的指南,涵盖 macOS 和 Windows 平台的配置步骤,如何在 Android 和 iOS…...

Leetcode 3577. Count the Number of Computer Unlocking Permutations
Leetcode 3577. Count the Number of Computer Unlocking Permutations 1. 解题思路2. 代码实现 题目链接:3577. Count the Number of Computer Unlocking Permutations 1. 解题思路 这一题其实就是一个脑筋急转弯,要想要能够将所有的电脑解锁&#x…...

1.3 VSCode安装与环境配置
进入网址Visual Studio Code - Code Editing. Redefined下载.deb文件,然后打开终端,进入下载文件夹,键入命令 sudo dpkg -i code_1.100.3-1748872405_amd64.deb 在终端键入命令code即启动vscode 需要安装插件列表 1.Chinese简化 2.ros …...
WordPress插件:AI多语言写作与智能配图、免费AI模型、SEO文章生成
厌倦手动写WordPress文章?AI自动生成,效率提升10倍! 支持多语言、自动配图、定时发布,让内容创作更轻松! AI内容生成 → 不想每天写文章?AI一键生成高质量内容!多语言支持 → 跨境电商必备&am…...
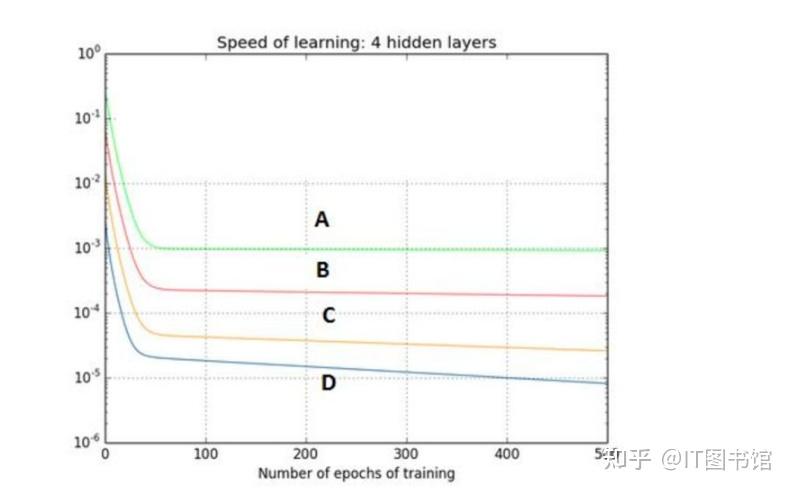
深度学习习题2
1.如果增加神经网络的宽度,精确度会增加到一个特定阈值后,便开始降低。造成这一现象的可能原因是什么? A、即使增加卷积核的数量,只有少部分的核会被用作预测 B、当卷积核数量增加时,神经网络的预测能力会降低 C、当卷…...
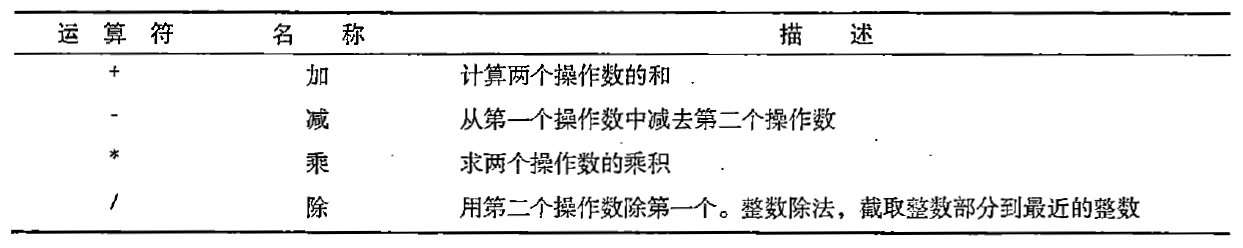
C# 表达式和运算符(求值顺序)
求值顺序 表达式可以由许多嵌套的子表达式构成。子表达式的求值顺序可以使表达式的最终值发生 变化。 例如,已知表达式3*52,依照子表达式的求值顺序,有两种可能的结果,如图9-3所示。 如果乘法先执行,结果是17。如果5…...

Cilium动手实验室: 精通之旅---13.Cilium LoadBalancer IPAM and L2 Service Announcement
Cilium动手实验室: 精通之旅---13.Cilium LoadBalancer IPAM and L2 Service Announcement 1. LAB环境2. L2公告策略2.1 部署Death Star2.2 访问服务2.3 部署L2公告策略2.4 服务宣告 3. 可视化 ARP 流量3.1 部署新服务3.2 准备可视化3.3 再次请求 4. 自动IPAM4.1 IPAM Pool4.2 …...

Python网页自动化Selenium中文文档
1. 安装 1.1. 安装 Selenium Python bindings 提供了一个简单的API,让你使用Selenium WebDriver来编写功能/校验测试。 通过Selenium Python的API,你可以非常直观的使用Selenium WebDriver的所有功能。 Selenium Python bindings 使用非常简洁方便的A…...

数据结构:递归的种类(Types of Recursion)
目录 尾递归(Tail Recursion) 什么是 Loop(循环)? 复杂度分析 头递归(Head Recursion) 树形递归(Tree Recursion) 线性递归(Linear Recursion)…...
