AC修炼计划(AtCoder Regular Contest 180) A~C
A - ABA and BAB
A - ABA and BAB (atcoder.jp)


这道题我一开始想复杂了,一直在想怎么dp,没注意到其实是个很简单的规律题。
我们可以发现我们住需要统计一下类似ABABA这样不同字母相互交替的所有子段的长度,而每个字段的的情况有(长度+1)/2种,最后所有字段情况的乘积就是最终答案。
#pragma GCC optimize(3) //O2优化开启
#include<bits/stdc++.h>
using namespace std;
#define int long long
typedef long long ll;
typedef unsigned long long ull;
typedef pair<int,int> PII;
// const int mod=1e9+7;
const int MX=0x3f3f3f3f3f3f3f3f;
//inline int read() //快读
//{
// int xr=0,F=1; char cr;
// while(cr=getchar(),cr<'0'||cr>'9') if(cr=='-') F=-1;
// while(cr>='0'&&cr<='9')
// xr=(xr<<3)+(xr<<1)+(cr^48),cr=getchar();
// return xr*F;
//}
//void write(int x) //快写
//{
// if(x<0) putchar('-'),x=-x;
// if(x>9) write(x/10); putchar(x%10+'0');
//}
// 比 unordered_map 更快的哈希表
// #include <ext/pb_ds/assoc_container.hpp>
// using namespace __gnu_pbds;
// const int RANDOM = chrono::high_resolution_clock::now().time_since_epoch().count();
// struct chash {
// int operator()(int x) const { return x ^ RANDOM; }
// };
// typedef gp_hash_table<int, int, chash> hash_t;
constexpr ll mod = 1e9 + 7; //此处为自动取模的数
class modint{ll num;
public:modint(ll num = 0) :num(num % mod){}ll val() const {return num;}modint pow(ll other) {modint res(1), temp = *this;while(other) {if(other & 1) res = res * temp;temp = temp * temp;other >>= 1;}return res;}constexpr ll norm(ll num) const {if (num < 0) num += mod;if (num >= mod) num -= mod;return num;}modint inv(){ return pow(mod - 2); }modint operator+(modint other){ return modint(num + other.num); }modint operator-(){ return { -num }; }modint operator-(modint other){ return modint(-other + *this); }modint operator*(modint other){ return modint(num * other.num); }modint operator/(modint other){ return *this * other.inv(); }modint &operator*=(modint other) { num = num * other.num % mod; return *this; }modint &operator+=(modint other) { num = norm(num + other.num); return *this; }modint &operator-=(modint other) { num = norm(num - other.num); return *this; }modint &operator/=(modint other) { return *this *= other.inv(); }friend istream& operator>>(istream& is, modint& other){ is >> other.num; other.num %= mod; return is; }friend ostream& operator<<(ostream& os, modint other){ other.num = (other.num + mod) % mod; return os << other.num; }
};int n;
string s;
void icealsoheat(){cin>>n;cin>>s;s=" "+s;int res=1;modint ans=1;for(int i=2;i<=n;i++){if(s[i]!=s[i-1]){res++;}else{if(res>=3){ans*=(res+1)/2;}res=1;}}if(res>=3){ans*=(res+1)/2;}cout<<ans;
}
signed main(){ios::sync_with_stdio(false); //int128不能用快读!!!!!!cin.tie();cout.tie();int _yq;_yq=1;// cin>>_yq;while(_yq--){icealsoheat();}
}
//
//⠀⠀⠀ ⠀⢸⣿⣿⣿⠀⣼⣿⣿⣦⡀
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⣀⠀⠀⠀ ⠀⢸⣿⣿⡟⢰⣿⣿⣿⠟⠁
//⠀⠀⠀⠀⠀⠀⠀⢰⣿⠿⢿⣦⣀⠀⠘⠛⠛⠃⠸⠿⠟⣫⣴⣶⣾⡆
//⠀⠀⠀⠀⠀⠀⠀⠸⣿⡀⠀⠉⢿⣦⡀⠀⠀⠀⠀⠀⠀ ⠛⠿⠿⣿⠃
//⠀⠀⠀⠀⠀⠀⠀⠀⠙⢿⣦⠀⠀⠹⣿⣶⡾⠛⠛⢷⣦⣄⠀
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⠀⣿⣧⠀⠀⠈⠉⣀⡀⠀ ⠀⠙⢿⡇
//⠀⠀⠀⠀⠀⠀⢀⣠⣴⡿⠟⠋⠀⠀⢠⣾⠟⠃⠀⠀⠀⢸⣿⡆
//⠀⠀⠀⢀⣠⣶⡿⠛⠉⠀⠀⠀⠀⠀⣾⡇⠀⠀⠀⠀⠀⢸⣿⠇
//⢀⣠⣾⠿⠛⠁⠀⠀⠀⠀⠀⠀⠀⢀⣼⣧⣀⠀⠀⠀⢀⣼⠇
//⠈⠋⠁⠀⠀⠀⠀⠀⠀⠀⠀⢀⣴⡿⠋⠙⠛⠛⠛⠛⠛⠁
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⣀⣾⡿⠋⠀
//⠀⠀⠀⠀⠀⠀⠀⠀⢾⠿⠋⠀
//
B - Improve Inversions
B - Improve Inversions (atcoder.jp)


这题确实不好想,但是get到点儿了就会觉得其实也不难。
我们需要尽可能的把逆序对最大化,从样例三我们可以发现,我们不妨确立左边一个要交换的下标i,然后找下标大于等于i+k,数值从大到小的找小于ai的数字,并依次与i的数字进行交换。为了尽可能减少替换的影响,我们按数值从小到大的次序去找这个下标i,从而参与上述的交换。因为我们是按从小到大的顺序的,所以小的数字参与交换并不会影响后面大的数字该交换的逆序对。
#pragma GCC optimize(3) //O2优化开启
#include<bits/stdc++.h>
using namespace std;
#define int long long
typedef long long ll;
typedef unsigned long long ull;
typedef pair<int,int> PII;
// const int mod=1e9+7;
const int MX=0x3f3f3f3f3f3f3f3f;
//inline int read() //快读
//{
// int xr=0,F=1; char cr;
// while(cr=getchar(),cr<'0'||cr>'9') if(cr=='-') F=-1;
// while(cr>='0'&&cr<='9')
// xr=(xr<<3)+(xr<<1)+(cr^48),cr=getchar();
// return xr*F;
//}
//void write(int x) //快写
//{
// if(x<0) putchar('-'),x=-x;
// if(x>9) write(x/10); putchar(x%10+'0');
//}
// 比 unordered_map 更快的哈希表
// #include <ext/pb_ds/assoc_container.hpp>
// using namespace __gnu_pbds;
// const int RANDOM = chrono::high_resolution_clock::now().time_since_epoch().count();
// struct chash {
// int operator()(int x) const { return x ^ RANDOM; }
// };
// typedef gp_hash_table<int, int, chash> hash_t;
// constexpr ll mod = 1e9 + 7; //此处为自动取模的数
// class modint{
// ll num;
// public:
// modint(ll num = 0) :num(num % mod){}// ll val() const {
// return num;
// }// modint pow(ll other) {
// modint res(1), temp = *this;
// while(other) {
// if(other & 1) res = res * temp;
// temp = temp * temp;
// other >>= 1;
// }
// return res;
// }// constexpr ll norm(ll num) const {
// if (num < 0) num += mod;
// if (num >= mod) num -= mod;
// return num;
// }// modint inv(){ return pow(mod - 2); }
// modint operator+(modint other){ return modint(num + other.num); }
// modint operator-(){ return { -num }; }
// modint operator-(modint other){ return modint(-other + *this); }
// modint operator*(modint other){ return modint(num * other.num); }
// modint operator/(modint other){ return *this * other.inv(); }
// modint &operator*=(modint other) { num = num * other.num % mod; return *this; }
// modint &operator+=(modint other) { num = norm(num + other.num); return *this; }
// modint &operator-=(modint other) { num = norm(num - other.num); return *this; }
// modint &operator/=(modint other) { return *this *= other.inv(); }
// friend istream& operator>>(istream& is, modint& other){ is >> other.num; other.num %= mod; return is; }
// friend ostream& operator<<(ostream& os, modint other){ other.num = (other.num + mod) % mod; return os << other.num; }
// };int n,k;
int a[200005];
int p[200005];
vector<PII>ans;
void icealsoheat(){cin>>n>>k;int bns=0;for(int i=1;i<=n;i++)cin>>a[i],p[a[i]]=i;for(int i=1;i<=n;i++){int id=p[i];int x=i;for(int j=i-1;j>=1;j--){if(p[j]>=id+k){// cout<<p[x]<<":::"<<p[j]<<"\n";ans.push_back({p[x],p[j]});a[id]=j;a[p[j]]=x;swap(p[x],p[j]);x=j;}}}cout<<ans.size()<<"\n";for(auto [i,j]:ans){cout<<i<<" "<<j<<"\n";}// for(int i=1;i<=n;i++){// cout<<a[i]<<" ";// }}
signed main(){ios::sync_with_stdio(false); //int128不能用快读!!!!!!cin.tie();cout.tie();int _yq;_yq=1;// cin>>_yq;while(_yq--){icealsoheat();}
}
//
//⠀⠀⠀ ⠀⢸⣿⣿⣿⠀⣼⣿⣿⣦⡀
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⣀⠀⠀⠀ ⠀⢸⣿⣿⡟⢰⣿⣿⣿⠟⠁
//⠀⠀⠀⠀⠀⠀⠀⢰⣿⠿⢿⣦⣀⠀⠘⠛⠛⠃⠸⠿⠟⣫⣴⣶⣾⡆
//⠀⠀⠀⠀⠀⠀⠀⠸⣿⡀⠀⠉⢿⣦⡀⠀⠀⠀⠀⠀⠀ ⠛⠿⠿⣿⠃
//⠀⠀⠀⠀⠀⠀⠀⠀⠙⢿⣦⠀⠀⠹⣿⣶⡾⠛⠛⢷⣦⣄⠀
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⠀⣿⣧⠀⠀⠈⠉⣀⡀⠀ ⠀⠙⢿⡇
//⠀⠀⠀⠀⠀⠀⢀⣠⣴⡿⠟⠋⠀⠀⢠⣾⠟⠃⠀⠀⠀⢸⣿⡆
//⠀⠀⠀⢀⣠⣶⡿⠛⠉⠀⠀⠀⠀⠀⣾⡇⠀⠀⠀⠀⠀⢸⣿⠇
//⢀⣠⣾⠿⠛⠁⠀⠀⠀⠀⠀⠀⠀⢀⣼⣧⣀⠀⠀⠀⢀⣼⠇
//⠈⠋⠁⠀⠀⠀⠀⠀⠀⠀⠀⢀⣴⡿⠋⠙⠛⠛⠛⠛⠛⠁
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⣀⣾⡿⠋⠀
//⠀⠀⠀⠀⠀⠀⠀⠀⢾⠿⠋⠀
//
C - Subsequence and Prefix Sum
C - Subsequence and Prefix Sum (atcoder.jp)


一道非常巧妙的dp题,他的状态转移非常的奇妙。
我们考虑前i位的数字对后面数字的贡献值。可以分成两种情况。
1.第i位数字没有被选中
2.第i位数字被选中
当第i位数字被选中时,每一个位数i它所能合成的状态数字都对后面i+1到n的数字有相应的贡献。而这里面0的情况比较特殊,如果第i位的合成数字是0,其实不会改变下一个选中的数字。
这里面有一种情况比较特殊
例如1 -1 5 5 .........
这里我们会发现,我们选择1和-1后,选择第3个5和第4个5的情况是重复的,所以我们要想办法将它去重。
#pragma GCC optimize(3) //O2优化开启
#include<bits/stdc++.h>
using namespace std;
#define int long long
typedef long long ll;
typedef unsigned long long ull;
typedef pair<int,int> PII;
// const int mod=1e9+7;
const int MX=0x3f3f3f3f3f3f3f3f;
//inline int read() //快读
//{
// int xr=0,F=1; char cr;
// while(cr=getchar(),cr<'0'||cr>'9') if(cr=='-') F=-1;
// while(cr>='0'&&cr<='9')
// xr=(xr<<3)+(xr<<1)+(cr^48),cr=getchar();
// return xr*F;
//}
//void write(int x) //快写
//{
// if(x<0) putchar('-'),x=-x;
// if(x>9) write(x/10); putchar(x%10+'0');
//}
// 比 unordered_map 更快的哈希表
// #include <ext/pb_ds/assoc_container.hpp>
// using namespace __gnu_pbds;
// const int RANDOM = chrono::high_resolution_clock::now().time_since_epoch().count();
// struct chash {
// int operator()(int x) const { return x ^ RANDOM; }
// };
// typedef gp_hash_table<int, int, chash> hash_t;
constexpr ll mod = 1e9 + 7; //此处为自动取模的数
class modint{ll num;
public:modint(ll num = 0) :num(num % mod){}ll val() const {return num;}modint pow(ll other) {modint res(1), temp = *this;while(other) {if(other & 1) res = res * temp;temp = temp * temp;other >>= 1;}return res;}constexpr ll norm(ll num) const {if (num < 0) num += mod;if (num >= mod) num -= mod;return num;}modint inv(){ return pow(mod - 2); }modint operator+(modint other){ return modint(num + other.num); }modint operator-(){ return { -num }; }modint operator-(modint other){ return modint(-other + *this); }modint operator*(modint other){ return modint(num * other.num); }modint operator/(modint other){ return *this * other.inv(); }modint &operator*=(modint other) { num = num * other.num % mod; return *this; }modint &operator+=(modint other) { num = norm(num + other.num); return *this; }modint &operator-=(modint other) { num = norm(num - other.num); return *this; }modint &operator/=(modint other) { return *this *= other.inv(); }friend istream& operator>>(istream& is, modint& other){ is >> other.num; other.num %= mod; return is; }friend ostream& operator<<(ostream& os, modint other){ other.num = (other.num + mod) % mod; return os << other.num; }
};int n,k;
int a[500005];
modint dp[105][5005];
modint sum[5005];
void icealsoheat(){cin>>n;for(int i=0;i<=20;i++)if(i!=10)sum[i]=1;for(int i=1;i<=n;i++)cin>>a[i];modint ans=1;for(int i=0;i<n;i++){dp[i][a[i]+1000]=dp[i][a[i]+1000]+sum[a[i]+10];sum[a[i]+10]=0;for(int j=0;j<=2000;j++){if(j==1000)continue;for(int o=i+1;o<=n;o++){// if(j+a[o]<0)cout<<"+++\n";if(j+a[o]<0)continue;dp[o][j+a[o]]+=dp[i][j];ans+=dp[i][j];}}for(int j=0;j<=20;j++){if(j!=10)sum[j]+=dp[i][1000];}}cout<<dp[2][1]<<"+++\n";cout<<ans;}
signed main(){ios::sync_with_stdio(false); //int128不能用快读!!!!!!cin.tie();cout.tie();int _yq;_yq=1;// cin>>_yq;while(_yq--){icealsoheat();}
}
//
//⠀⠀⠀ ⠀⢸⣿⣿⣿⠀⣼⣿⣿⣦⡀
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⣀⠀⠀⠀ ⠀⢸⣿⣿⡟⢰⣿⣿⣿⠟⠁
//⠀⠀⠀⠀⠀⠀⠀⢰⣿⠿⢿⣦⣀⠀⠘⠛⠛⠃⠸⠿⠟⣫⣴⣶⣾⡆
//⠀⠀⠀⠀⠀⠀⠀⠸⣿⡀⠀⠉⢿⣦⡀⠀⠀⠀⠀⠀⠀ ⠛⠿⠿⣿⠃
//⠀⠀⠀⠀⠀⠀⠀⠀⠙⢿⣦⠀⠀⠹⣿⣶⡾⠛⠛⢷⣦⣄⠀
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⠀⣿⣧⠀⠀⠈⠉⣀⡀⠀ ⠀⠙⢿⡇
//⠀⠀⠀⠀⠀⠀⢀⣠⣴⡿⠟⠋⠀⠀⢠⣾⠟⠃⠀⠀⠀⢸⣿⡆
//⠀⠀⠀⢀⣠⣶⡿⠛⠉⠀⠀⠀⠀⠀⣾⡇⠀⠀⠀⠀⠀⢸⣿⠇
//⢀⣠⣾⠿⠛⠁⠀⠀⠀⠀⠀⠀⠀⢀⣼⣧⣀⠀⠀⠀⢀⣼⠇
//⠈⠋⠁⠀⠀⠀⠀⠀⠀⠀⠀⢀⣴⡿⠋⠙⠛⠛⠛⠛⠛⠁
//⠀⠀⠀⠀⠀⠀⠀⠀⠀⣀⣾⡿⠋⠀
//⠀⠀⠀⠀⠀⠀⠀⠀⢾⠿⠋⠀
//
相关文章:

AC修炼计划(AtCoder Regular Contest 180) A~C
A - ABA and BAB A - ABA and BAB (atcoder.jp) 这道题我一开始想复杂了,一直在想怎么dp,没注意到其实是个很简单的规律题。 我们可以发现我们住需要统计一下类似ABABA这样不同字母相互交替的所有子段的长度,而每个字段的的情况有ÿ…...

云计算练习题
第一题:每周日晚上11点59分需要将/data目录打包压缩到/mnt目录下并以时间命名 #crontab -e 59 23 * * 7 /bin/tar czvf /mnt/date %F-data.tar.gz /data 59 23 * * 7 /bin/tar czvf /mnt/date %T.tar.gz /data 第二题:查找出系统中/application目录下所有…...
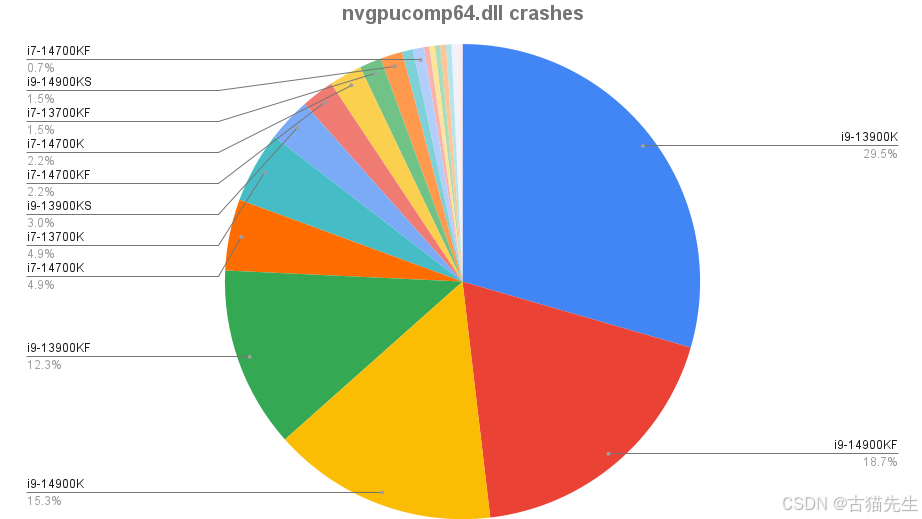
《战甲神兵》开发者报告:游戏崩溃问题80%发生在Intel可超频酷睿i9处理器上——酷睿i7 K系列CPU也表现出高崩溃率
在Intel持续面临第13代和第14代CPU崩溃问题的背景下,近日,《战甲神兵》(Warframe)的开发者们于7月9日披露了游戏崩溃的统计数据,并描述了诊断该问题的过程。根据开发团队的说法,一名未进行超频且使用全新PC的员工,即便…...
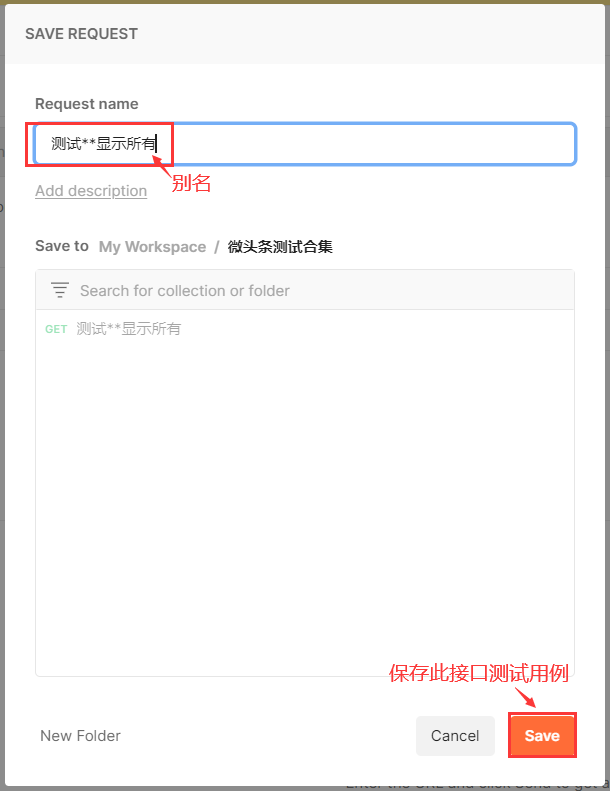
Postman下载及使用说明
Postman使用说明 Postman是什么? Postman是一款接口对接工具【接口测试工具】 接口(前端接口)是什么? 前端发送的请求普遍被称为接口 通常有网页的uri参数格式json/key-value请求方式post/get响应请求的格式json 接…...

什么是im即时通讯?WorkPlus im即时通讯私有化部署安全可控
IM即时通讯是Instant Messaging的缩写,指的是一种实时的、即时的电子信息交流方式,也被称为即时通讯。它通过互联网和移动通信网络,使用户能够及时交换文本消息、语音通话、视频通话、文件共享等信息。而WorkPlus im即时通讯私有化部署则提供…...

hnust 1794: 机器翻译
hnust 1794: 机器翻译 题目描述 小晨的电脑上安装了一个机器翻译软件,他经常用这个软件来翻译英语文章。 这个翻译软件的原理很简单,它只是从头到尾,依次将每个英文单词用对应的中文含义来替换。对于每个英文单词,软件会先在内存…...

AI人工智能开源大模型生态体系分析
人工智能开源大模型生态体系研究 "人工智能开源大模型生态体系研究报告v1.0"揭示,AI(A)的飞速发展依赖于三大核心:数据、算法和算力。这一理念已得到业界广泛认同,三者兼备才能推动AI的壮大发展。随着AI大模型的扩大与普及…...

ArkTS学习笔记_封装复用之@Styles装饰器
ArkTS学习笔记_封装复用之Styles装饰器 背景: 在开发中,如果每个组件的样式都需要单独设置,就会出现大量代码在进行重复样式设置,虽然可以复制粘贴,但为了代码简洁性和后续方便维护,给出的思路是ÿ…...

根据vue学习react
react的函数式组件与vue2是很像的 一、基础类似点 1、组件下拥有一个根节点,vue2是template,react是幽灵标签<> 2、vue2是{{}}以及v-model,react的绑定是{} 3、vue2编译html是v-html,react是{},并且react的jsx中…...

Hi3861 OpenHarmony嵌入式应用入门--HTTPD
httpd 是 Apache HTTP Server 的守护进程名称,Apache HTTP Server 是一种广泛使用的开源网页服务器软件。 本项目是从LwIP中抽取的HTTP服务器代码; Hi3861 SDK中已经包含了一份预编译的lwip,但没有开启HTTP服务器功能(静态库无法…...
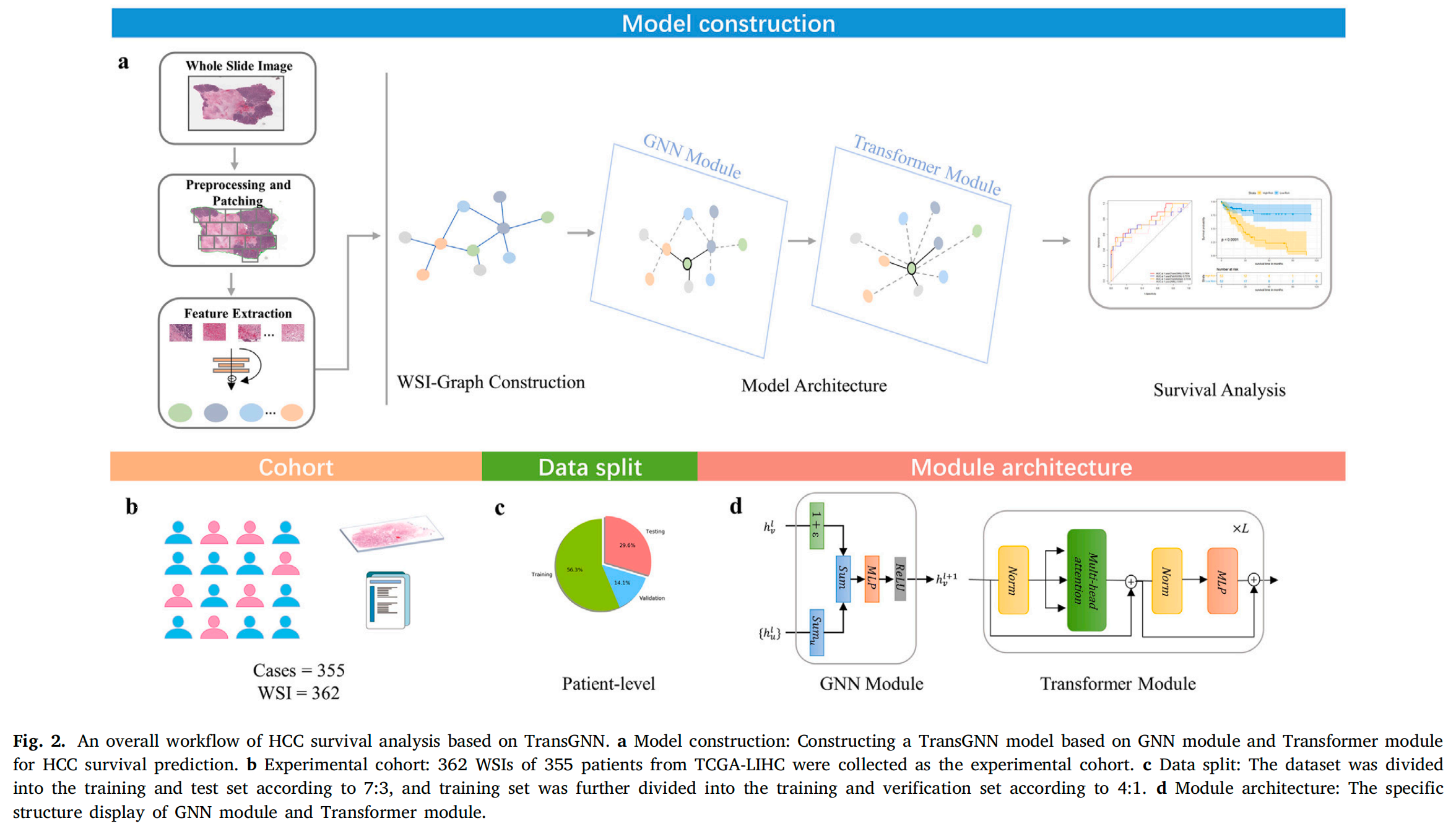
MICS2024|少样本学习、多模态技术以及大语言模型在医学图像处理领域的研究进展|24-07-14
小罗碎碎念 本期推文主题 今天的会议很多主题都集中在大模型、多模态这两个方面,很明显,这两个方向都是目前的研究热点。 所以,我这一期推文会先简单的分析一下秦文健(中科院)和史淼晶(同济大学)…...
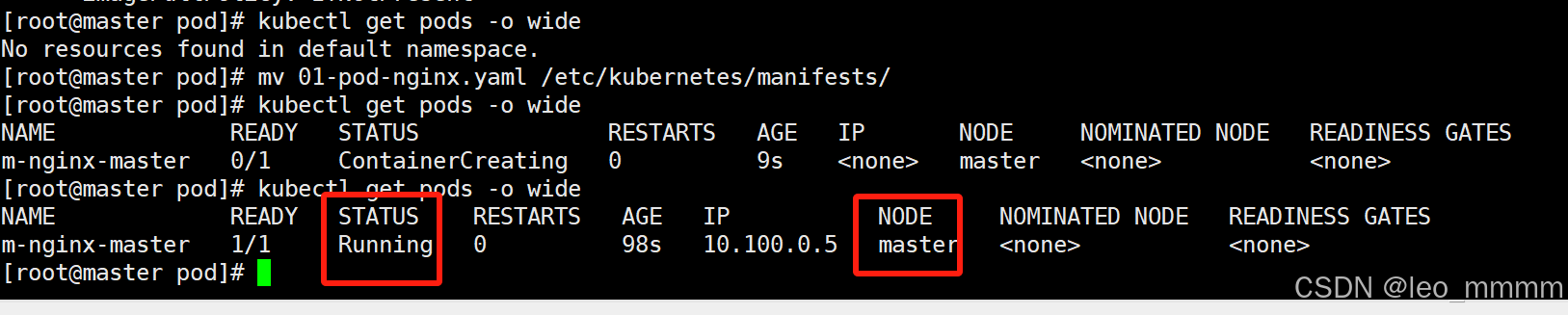
ConfigMap-secrets-静态pod
一.ConfigMap 1.概述 ConfigMap资源,简称CM资源,它生成的键值对数据,存储在ETCD数据库中 应用场景:主要是对应用程序的配置 pod通过env变量引入ConfigMap,或者通过数据卷挂载volume的方式引入ConfigMap资源 官方解释…...

SQL Error: 1406, SQLState: 22001
SQL错误代码1406和SQLState 22001通常表示“列数据过长”错误。这意味着尝试插入或更新列中的值,但该值的长度超过了该列允许的最大长度。 解决此问题的几个步骤: 检查列长度: 确定引起错误的列。检查数据库架构中该列允许的最大长度。 验证…...
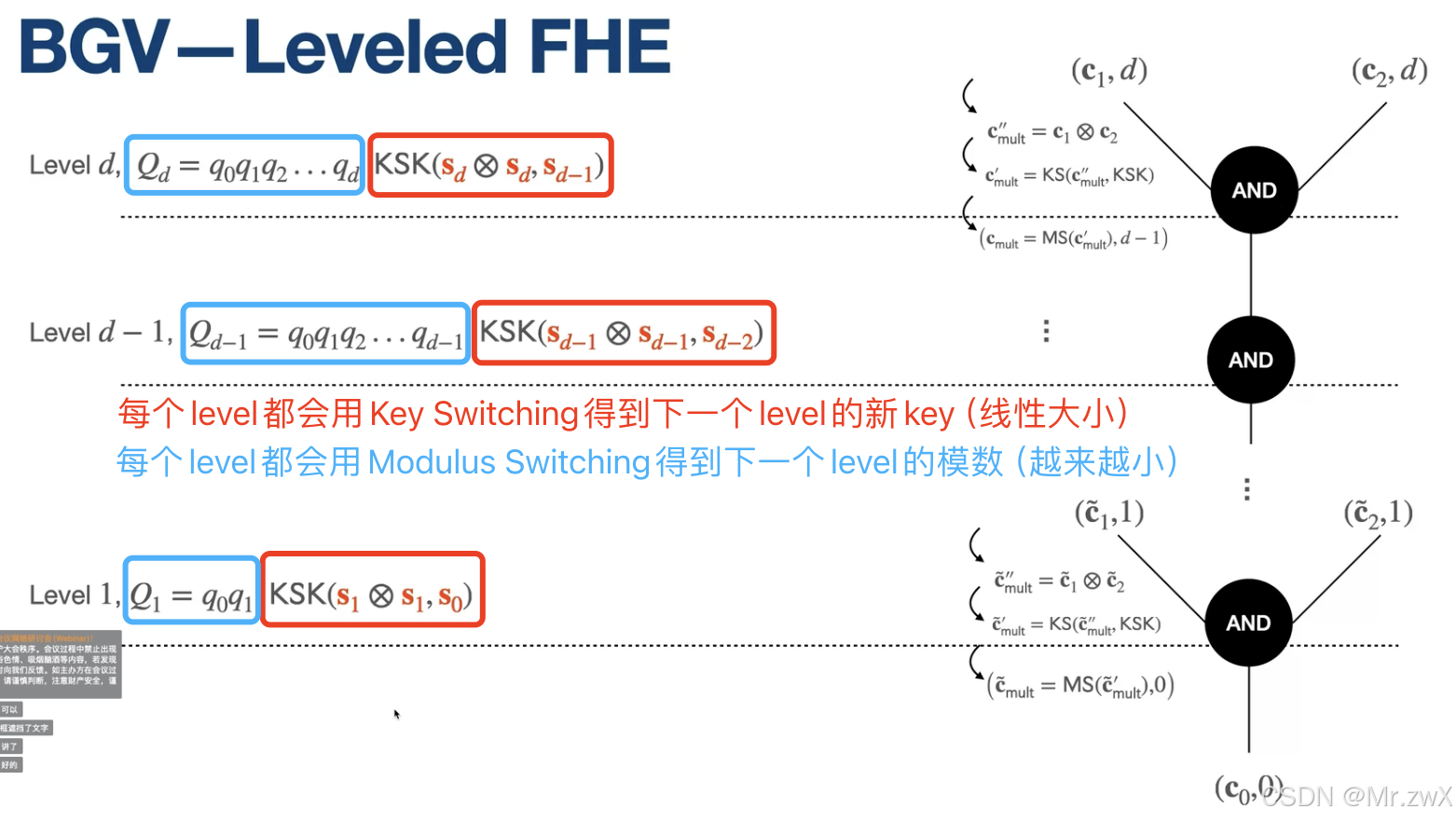
【密码学基础】基于LWE(Learning with Errors)的全同态加密方案
学习资源: 全同态加密I:理论与基础(上海交通大学 郁昱老师) 全同态加密II:全同态加密的理论与构造(Xiang Xie老师) 现在第二代(如BGV和BFV)和第三代全同态加密方案都是基…...
Linux - 基础开发工具(yum、vim、gcc、g++、make/Makefile、git)
目录 Linux软件包管理器 - yum Linux下安装软件的方式 认识yum 查找软件包 安装软件 如何实现本地机器和云服务器之间的文件互传 卸载软件 Linux编辑器 - vim vim的基本概念 vim下各模式的切换 vim命令模式各命令汇总 vim底行模式各命令汇总 vim的简单配置 Linux编译器 - gc…...

网络安全法律框架更新:最新合规要求与企业应对策略
网络安全法律框架的最新更新 近期,中国的网络安全法律框架经历了重要的更新。2022年,《网络安全法》迎来了首次修改,这一修订主要是为了与《数据安全法》和《个人信息保护法》等新实施的法律进行衔接协调,完善法律责任制度&#x…...

数仓工具—Hive语法之正则表达式函数
正则表达式函数 之前我们介绍过like rlike regexp 这些关键字,都是和匹配有关的,今天我们介绍一下hive 的REGEXP_REPLACE 和REGEXP_EXTRACT 函数,背景是使用Hive正则表达式函数提取数字 在我的其他文章中,我们已经看到了如何使用Hive正则表达式从字符串中提取日期值。正则…...
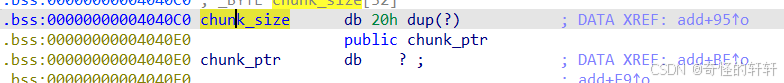
WKCTF 2024 easy_heap
很经典的house of orange unsortedbin attack FSOP 变量覆盖 不能 free,那首先想到就是 house of orange泄露Libc基址,然后unsortedbin attack。 但是只能show(8),就不能用largebin的套路来泄露堆地址了,那怎么办呢? …...

SQL 多变关联使用子查询去重
不去重状态 select a.*,b.recon_amt from free_settlement_first aleft join free_settlement_second b on a.settlement_first_id b.settlement_first_id 有2条数据出现了重复 使用子查询去重 select a.*,b.recon_amt from free_settlement_first aleft join free_settlem…...
)
php表单提交并自动发送邮件给某个邮箱(示例源码下载)
只需要将以下代码内容进行复制即可用到自己的程序/API接口中: <?php if(!empty($_POST[is_post]) && $_POST[is_post]1){$url "https://www.aoksend.com/index/api/send_email";$name $_POST[name];$email $_POST[email];$subject $_POS…...
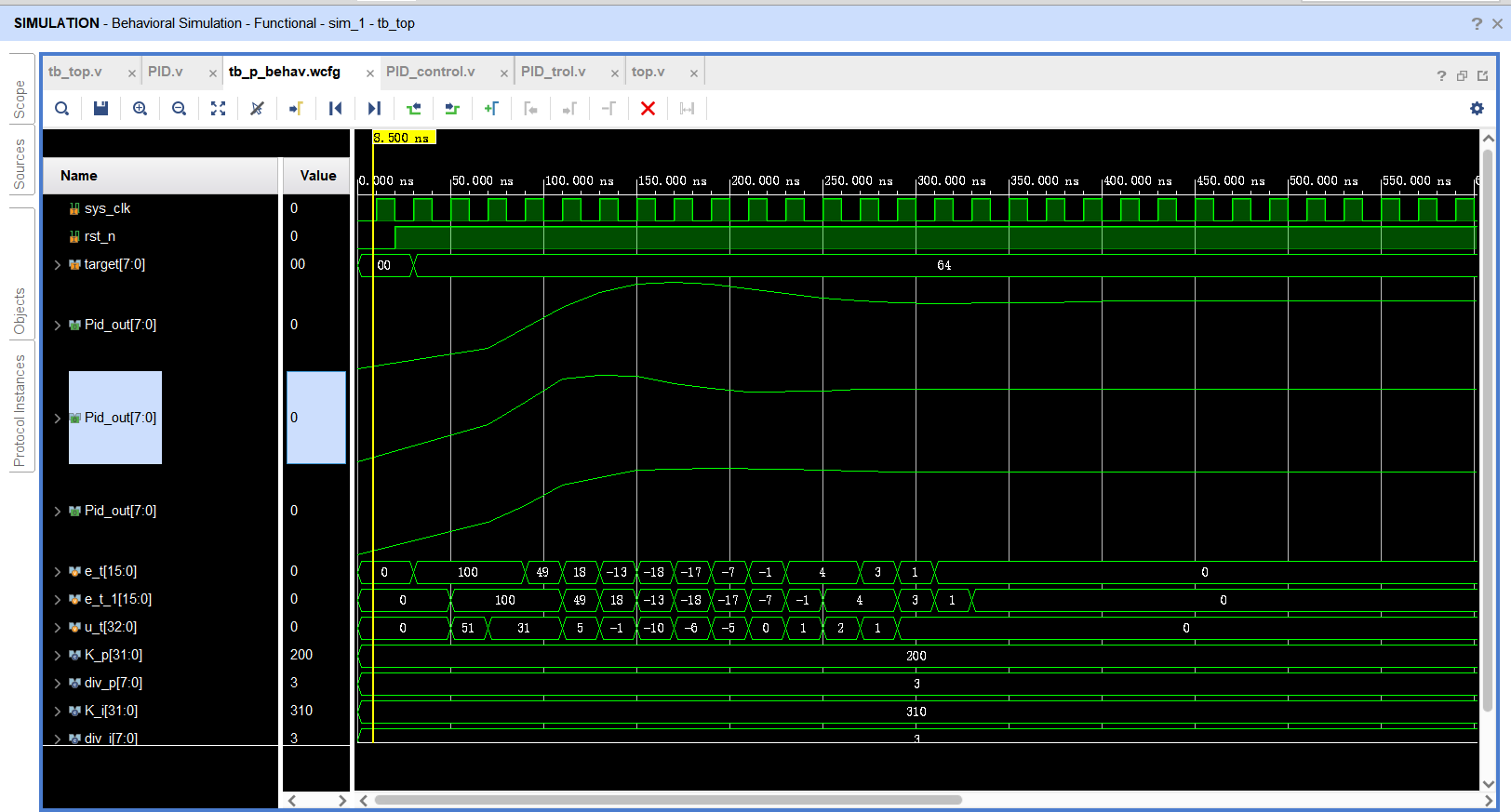
基于FPGA的PID算法学习———实现PID比例控制算法
基于FPGA的PID算法学习 前言一、PID算法分析二、PID仿真分析1. PID代码2.PI代码3.P代码4.顶层5.测试文件6.仿真波形 总结 前言 学习内容:参考网站: PID算法控制 PID即:Proportional(比例)、Integral(积分&…...

Leetcode 3576. Transform Array to All Equal Elements
Leetcode 3576. Transform Array to All Equal Elements 1. 解题思路2. 代码实现 题目链接:3576. Transform Array to All Equal Elements 1. 解题思路 这一题思路上就是分别考察一下是否能将其转化为全1或者全-1数组即可。 至于每一种情况是否可以达到…...
大数据零基础学习day1之环境准备和大数据初步理解
学习大数据会使用到多台Linux服务器。 一、环境准备 1、VMware 基于VMware构建Linux虚拟机 是大数据从业者或者IT从业者的必备技能之一也是成本低廉的方案 所以VMware虚拟机方案是必须要学习的。 (1)设置网关 打开VMware虚拟机,点击编辑…...

使用van-uploader 的UI组件,结合vue2如何实现图片上传组件的封装
以下是基于 vant-ui(适配 Vue2 版本 )实现截图中照片上传预览、删除功能,并封装成可复用组件的完整代码,包含样式和逻辑实现,可直接在 Vue2 项目中使用: 1. 封装的图片上传组件 ImageUploader.vue <te…...
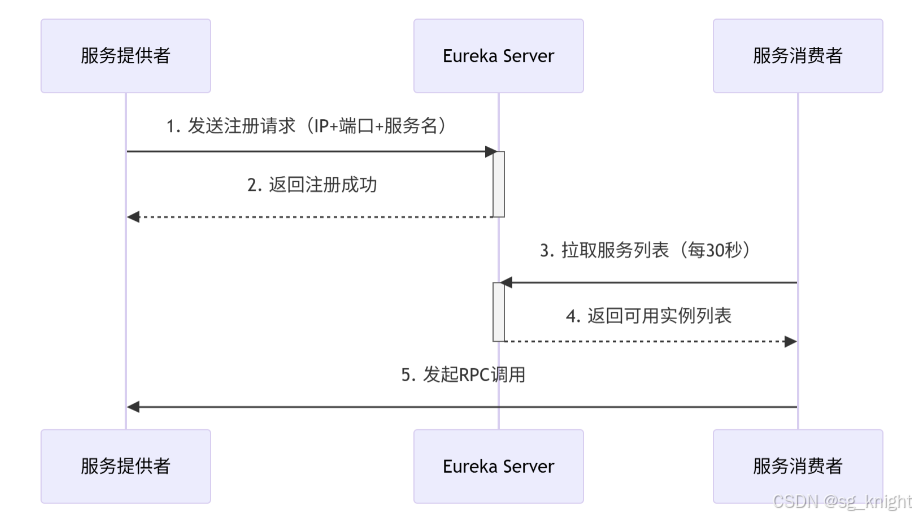
Springcloud:Eureka 高可用集群搭建实战(服务注册与发现的底层原理与避坑指南)
引言:为什么 Eureka 依然是存量系统的核心? 尽管 Nacos 等新注册中心崛起,但金融、电力等保守行业仍有大量系统运行在 Eureka 上。理解其高可用设计与自我保护机制,是保障分布式系统稳定的必修课。本文将手把手带你搭建生产级 Eur…...
Springboot社区养老保险系统小程序
一、前言 随着我国经济迅速发展,人们对手机的需求越来越大,各种手机软件也都在被广泛应用,但是对于手机进行数据信息管理,对于手机的各种软件也是备受用户的喜爱,社区养老保险系统小程序被用户普遍使用,为方…...

2025季度云服务器排行榜
在全球云服务器市场,各厂商的排名和地位并非一成不变,而是由其独特的优势、战略布局和市场适应性共同决定的。以下是根据2025年市场趋势,对主要云服务器厂商在排行榜中占据重要位置的原因和优势进行深度分析: 一、全球“三巨头”…...
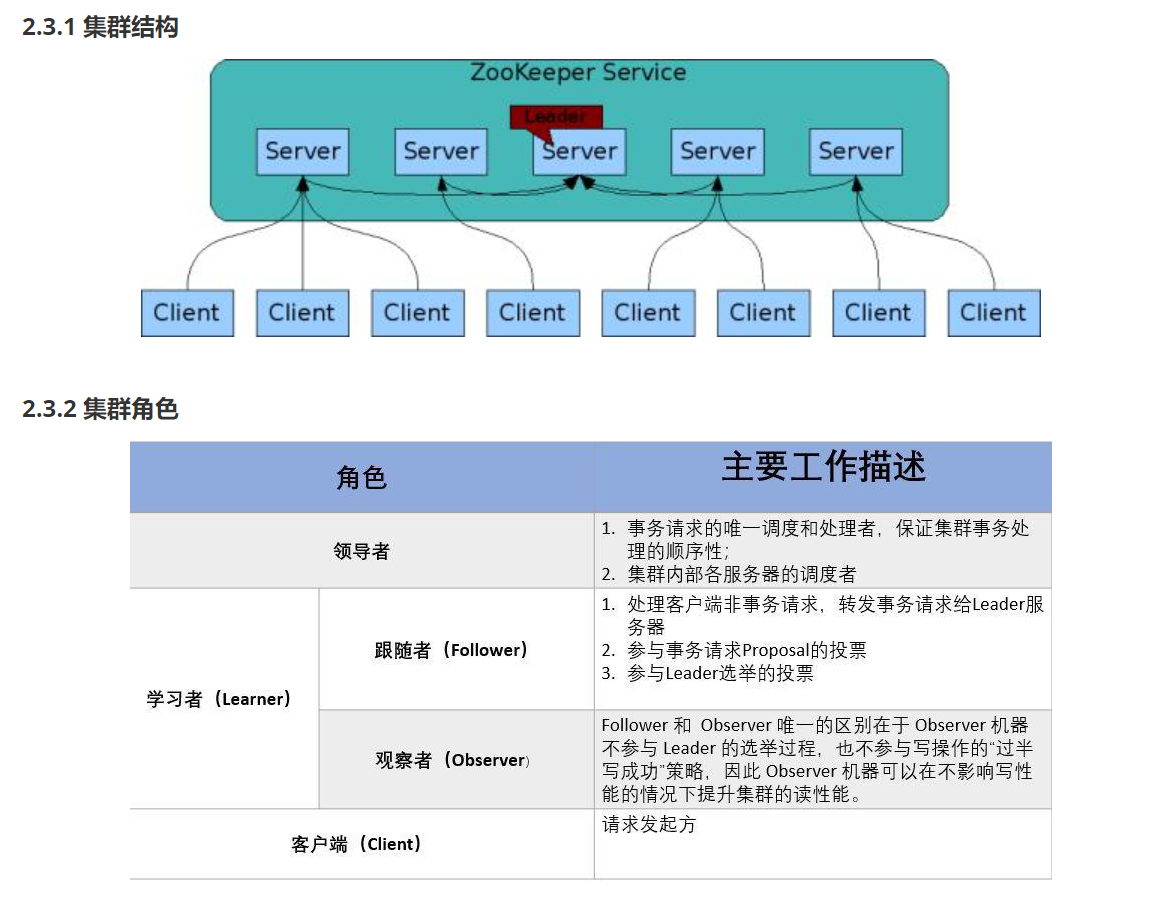
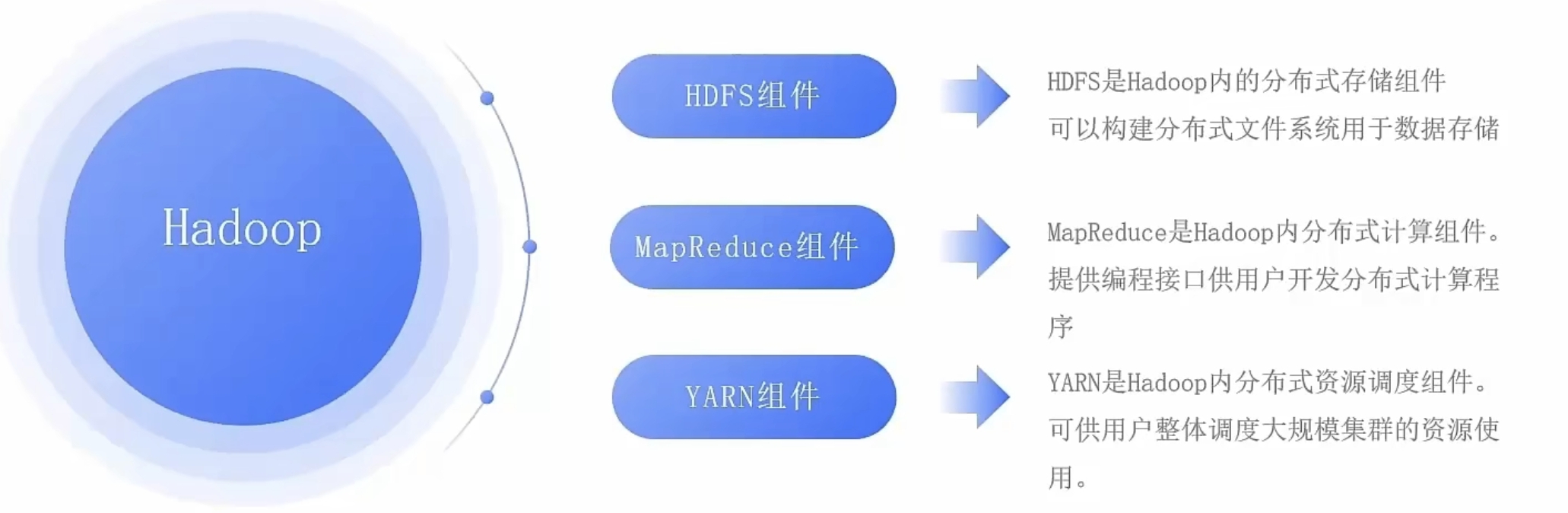
HDFS分布式存储 zookeeper
hadoop介绍 狭义上hadoop是指apache的一款开源软件 用java语言实现开源框架,允许使用简单的变成模型跨计算机对大型集群进行分布式处理(1.海量的数据存储 2.海量数据的计算)Hadoop核心组件 hdfs(分布式文件存储系统)&a…...

Git 3天2K星标:Datawhale 的 Happy-LLM 项目介绍(附教程)
引言 在人工智能飞速发展的今天,大语言模型(Large Language Models, LLMs)已成为技术领域的焦点。从智能写作到代码生成,LLM 的应用场景不断扩展,深刻改变了我们的工作和生活方式。然而,理解这些模型的内部…...

提升移动端网页调试效率:WebDebugX 与常见工具组合实践
在日常移动端开发中,网页调试始终是一个高频但又极具挑战的环节。尤其在面对 iOS 与 Android 的混合技术栈、各种设备差异化行为时,开发者迫切需要一套高效、可靠且跨平台的调试方案。过去,我们或多或少使用过 Chrome DevTools、Remote Debug…...
